Category: Class 7

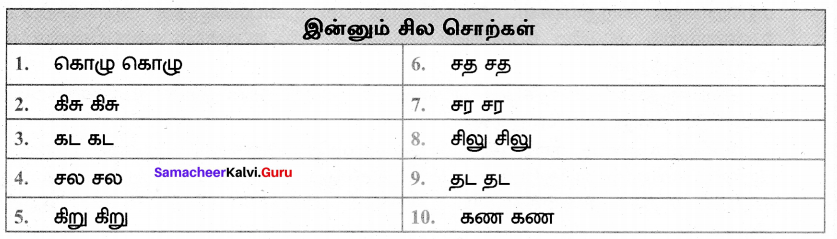
Students can Download Tamil Chapter 2.4 பள்ளி மறுதிறப்பு Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 2 Chapter 2.4 பள்ளி மறுதிறப்பு

மதிப்பீடு

Question 1.
மதிவாணன் பள்ளிக்குச் செல்ல முடிவெடுத்த நிகழ்வைச் சுருக்கி எழுதுக.
Answer:
மதிவாணன் கோடை விடுமுறையில் நாலைந்து நாள்கள் அக்கா வீடு, அத்தை வீடு என்று சென்று வந்தான். தொலைக்காட்சியைப் பார்த்துப் பொழுதுபோக்கினான்.
மதிவாணனின் நண்பன் கவின், “சும்மாதானே இருக்கே. வாடா பின்னலாடை நிறுவனத்துக்குப் போகலாம். புதிய உடைகள், எழுதுகோல்கள், குறிப்பேடுகள் வாங்கப் பணம் சம்பாதித்து விடுவாய்” என்றான். மதிவாணனும் சேர்ந்துவிட்டான்.

மதிவாணனுக்குப் புதிய அனுபவம் கிடைத்தது. சனிக்கிழமை வாரச் சம்பளம் கிடைத்தது. மேலும் வடை, தேநீர், இரவில் பரோட்டா, தோசை என்று சாப்பிட முடிந்தது.

வாழ்க்கை முழுவதும் தொழிலாளியா? எனச் சிந்தித்தான். தொழிலாளியாக இருப்பது கேவலமில்லை. ஆனால் படிக்கிற வயதில் வேலை தேவையா? எனச் சிந்தித்தான்.

படித்தால் வேறு வேலை கிடைக்கும். நல்ல வருமானம் வரும் எனத் தோன்றியது. கல்வியறிவு முதன்மையானது. ஒரு பட்டமாவது வாங்கவேண்டும் என எண்ணினான். அப்துல்கலாமும் அம்பேத்கரும் இருந்த விளம்பரப் பலகை கண்ணில் பட்டது. அவர்களைப் போல் உயர வேண்டுமெனில் படிக்க வேண்டும் என உணர்ந்தான்.

மதிவாணன் பேருந்து நிலையத்தில் நின்று கொண்டிருந்த போது படிப்பறிவில்லாத முதியவர் ஒருவர், அங்கு வந்து நின்ற பேருந்து நல்லூர் போகுமா?” என்று கேட்டார். ஒரு சிறுவன் பேருந்தின் முகப்பைப் பார்த்தான். எதுவும் பேசாமல் புன்முறுவல் பூத்தான்.

“என்னப்பா போகுமா?” என்று மீண்டும் கேட்டார். “யாருக்குத் தெரியும்? எங்களுக்குப் படிக்கத் தெரியாதே” என்று கூறியபடி ஒரு சிறுவன் முகத்தைத் திருப்பிக் கொண்டான். பெரியவர் “இதுகூட படிச்சுச் சொல்லத் தெரியாதா?” என்றார். ஒரு சிறுவன் பெரியவரே. இதப் படிக்கக் கூட உங்களுக்குத் தெரியாதா?” என்றான். மற்றவர்கள் சிரித்தனர். மதிவாணன் அப்பெரியவரிடம் “பேருந்து வந்தா சொல்றேன்” என்றான்.

முதியவர் கல்வியறிவு இல்லாததால் அவமானப்பட்டதைக் கவனித்தான். கல்வியறிவு இல்லையெனில் தானும் இவ்வாறு அவமானப்பட நேரும் என்ற எண்ணம் வந்தது.

நல்ல கல்வியறிவு தலைநிமிர்ந்து நிற்க வைக்கும். நல்ல படிப்புதான் சிறந்த மனிதனாக்கும் என்பதை உணர்ந்தான். வேலைக்குப் போவதை விடுத்துப் பள்ளிக்குச் செல்ல முடிவெடுத்தான்.

கற்பவை கற்றபின்

Question 1.
பள்ளி மறுதிறப்பு’ என்னும் கதையை வகுப்பில் நாடகமாக நடித்துக் காட்டுக.
Answer:
மாணவர்கள் தாங்களாகவே செய்ய வேண்டியவை.

Question 2.
எழுதப் படிக்கத் தெரியாதவர்களுக்கு எவ்வாறு உதவுவீர்கள்? வகுப்பில் கலந்துரையாடுக.
மாணவன் -1 : டேய் மணியைப் பார்த்தாயா? தேர்வில் முட்டை மதிப்பெண்
பெற்றுள்ளான்.
மாணவன் -2 : அப்படியெல்லாம் ஒருவரை ஏளனப்படுத்தாதே. அவன் நம் வகுப்பில் படிக்கும் மாணவன். அவனுக்கு என்ன பிரச்சனை? எதனால் படிக்க இயலவில்லை என்று சிந்தித்தாயா? அதை விட்டுவிட்டு ஏளனப்படுத்துகிறாயே?
மாணவன் -1 : என்னை மன்னித்துவிடு. ஏதோ விளையாட்டுத்தனமாகப் பேசிவிட்டேன் அவனுக்கு எவ்வாறு உதவலாம் என்று சொல்.
மாணவன் -2 : இதுதான் படிப்பவருக்கு அழகு. நீயும் நானும் சேர்ந்து அவனுக்குப் டு
பள்ளி வேளை நேரம் முடிந்ததும் படிப்பதற்குக் கற்றுக் கொடுக்கலாம். நீ அவனுடைய வீட்டிற்கு அருகில் இருக்கிறாய். தினமும் காலையில் கற்றுக்கொடு. நான் தினமும் மாலையில் பள்ளியிலேயே கற்றுக் கொடுக்கிறேன்.
மாணவன் -1 : நான் மொழிப் பாடங்களையும் அறிவியல் பாடத்தையும் கற்றுக் கொடுக்கிறேன்.
மாணவன் -2 : நான் கணிதம், சமூக அறிவியல் இரண்டு பாடத்தையும் கற்றுக் கொடுக்கிறேன்.
மாணவன் -1 : மொழிப்பாடத்தில் முதலில் எழுத்துகளை அடையாளப்படுத்தி புதிய
சொற்கள் உருவாக்குதல் போன்ற மொழிப்பயிற்சிகள் மூலம் எளிய முறையில் கற்றுக் கொடுப்பேன். சொந்தமாகப் பேசுதல், கடிதம், கட்டுரை எழுதுதல் ஆகியவற்றையும் கற்றுக் கொடுப்பேன்.
மாணவன் -2 : நானும் கணிதத்தை வாய்பாடுகள் படிக்க வைத்தல், எளிமையான வழிமுறைகளில் அறிவியலை இன்றைய வாழ்வியலுடனும் குடும்பத்திடனும் ஒப்பிட்டுப் பார்த்து புரிந்து கொள்ளச் செய்வேன்.
மாணவன் -1 : அறிவியல் பாடத்தை நாம் பயன்படுத்தும் பொருட்களுடன் ஒப்பிட்டுக்
கற்றுக் கொடுப்பேன்.

மாணவன் -2 : ஆம். நாம் இருவரும் சேர்ந்து மணியைப் படிக்க வைத்து அடுத்த
தேர்வில் நல்ல மதிப்பெண் பெறச் செய்வோம்.

Students can Download Tamil Chapter 1.1 விருந்தோம்பல் Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 1.1 விருந்தோம்பல்

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
மரம் வளர்த்தால் …………………………….. பெறலாம்.
அ) மாறி
ஆ) மாரி
இ) காரி
ஈ) பாரி
Answer:
ஆ) மாரி

Question 2.
‘நீருலையில் என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது …………….
அ) நீரு + உலையில்
ஆ) நீர் + இலையில்
இ) நீர் + உலையில்
ஈ) நீரு + இலையில்
Answer:
இ) நீர் + உலையில்

Question 3.
மாரி + ஒன்று என்பதனைச் சேர்த்தெழுதக் கிடைக்கும் சொல் …………….
அ) மாரியொன்று
ஆ) மாரி ஒன்று
இ) மாரியின்று
ஈ) மாரியன்று
Answer:
அ) மாரியொன்று

குறுவினா

Question 1.
பாரி மகளிரின் பெயர்களை எழுதுக.
Answer:
அங்கவை, சங்கவை என்போர் பாரியின் மகள்களாவர்.

Question 2.
பொருள் ஏதும் இல்லாத வீடுகளே இல்லை ‘ – எவ்வாறு?
Answer:
பாரி மகளிர் உலை நீரில் பொன் இட்டுத் தந்ததால் பொருள் ஏதும் இல்லாத வீடுகளே இல்லை என்பது புலனாகிறது.

சிந்தனை வினா

தமிழர்களின் பிற பண்பாட்டுக் கூறுகளை எழுதுக.

தமிழர்களின் பிற பண்பாட்டுக் கூறுகள் : (பண்பாடு – நம்மைப் பண்படுத்துதல்)
(i) ஒழுக்க ம்
(ii) சிறந்த கல்வியைப் பெறுதல்
(iii) பெரியோரை மதித்தல்
(iv) நன்றியுணர்வுடன் இருத்தல்
(V) ஈகைக் குணம்
(vi) விருந்தோம்பல்
(vii) முன்னோர் கூறியவற்றைப் பின்பற்றுதல்
(viii) மேலைநாட்டு உணவைத் தவிர்த்து நம் பாரம்பரிய உணவை உண்ணுதல்.
(ix) உடலை மறைக்கும் ஆடை அணிதல்.
(x) உறவினர்களைப் பேணி பாதுகாத்தல்
(xi) நாட்டுப்பற்றுடனும், மொழிப்பற்றுடனும் இருத்தல்.

கற்பவை கற்றபின்

Question 1.
வள்ளல்கள் எழுவரின் பெயர்களைத் தொகுத்து எழுதுக.
Answer:

Question 2.
விருந்தோம்பல் பண்பை விளக்கும் கதை ஒன்றை அறிந்து வந்து வகுப்பறையில் கூறுக.
Answer:
விருந்தோம்பல் பண்பு – கதை : மாறனார் என்ற சிவனடியார் பற்றிய கதை :
மாறனார் தம் முயற்சியினாலும் உழைப்பினாலும் உழவுத்தொழில் செய்து அதில் ஈட்டிய செல்வத்தைக் கொண்டு சிவனடியார்களுக்கும் நலிந்தவர்களுக்கும் அன்னமிட்டு மகிழ்வார். இவர் இளையான்குடி என்ற ஊரில் வாழ்ந்தவர். தன் மனைவி புனிதவதியுடன் இணைந்து இத்திருத்தொண்டை செய்தார்.

மாறனாரும், புனிதவதியும் சிவனடியார்களுக்குத் தினமும் அன்னமிட்டு அவர்களுக்குப் பாத பூஜை செய்து சிவபெருமானை வழிபட்டு வந்தனர். இவருடைய சிவத்தொண்டை உலகறியச் செய்ய எண்ணினார் சிவபெருமான். தன் திருவிளையாடலால் மாறனாரின் வீட்டில் வறுமை சூழச் செய்தார். ஆனாலும், மாறனார் கடன் வாங்கியும் நிலங்களை விற்றும் அன்னமிட்டார்.

ஒருநாள் கடும் மழை பெய்து கொண்டிருந்தது. மாறனாரும் அவரது மனைவியும் பசியில் வாடிக் கொண்டிருந்தனர். அன்று நள்ளிரவில் சிவபெருமான், அடியவர் கோலத்தில் மாறனார் வீட்டிற்கு வந்தார். அவரைப் பார்த்ததும் இருவரும் அகமகிழ்ந்தனர். ஆனால் அன்னமிடுவதற்கு என்ன செய்வது என்று வருந்தினர்.

புனிதவதி, மாறனாரிடம், “நாம் இன்று காலையில் வயலில் விதைத்து வந்த விதை நெல்லினைச் சேகரித்து எடுத்து வந்து தந்தால், நான் அதனை சமைத்து அடியவருக்கு நீ அன்னம் பரிமாறுவேன்” என்றாள்.

அடியவரைக் கொஞ்ச நேரம் காத்திருக்கும்படிக் கூறிவிட்டு, வயலுக்குச் சென்றார் – மாறனார். புனிதவதி மிச்சமிருந்த விறகை வைத்து தோட்டத்தில் இருந்த கீரையைச் சமைத்தாள். மாறனார் வருகைக்குக் காத்திருந்தாள். அடியவர் கண்ணயர்ந்து விட்டார்.

மாறனார் ஒரு வழியாக நீரில் மிதந்த விதைநெல்லை எடுத்து வந்தார். விறகு இல்லாததால் வீட்டின் கூரையில் இருந்த குச்சிகளை எடுத்துக் கொடுத்தார். புனிதவதி நெல்லை உரலில் இட்டுக் குத்தி அரிசியை எடுத்து உலையிலிட்டுச் சோறாக்கினாள்.

பிறகு உறங்கிக் கொண்டிருந்த அடியவரை உணவு உண்பதற்காக மாறனார் எழுப்பினார். அப்போது சோதி வடிவாக இறைவன் தோன்றினார். “மாறனாரே, உங்கள் இருவரின் விருந்தோம்பல் பண்பினை உலகிற்கு உணர்த்தவே நான் இவ்வாறு செய்தேன். ” இனி நீங்கள் செல்வங்கள் அனைத்தையும் பெற்று பல காலம் தொண்டு செய்து என்னை வந்து அடைவீர்களாக,” என்று கூறிவிட்டு மறைந்தார்.
இப்புராணக்கதை நமக்கு விருந்தோம்பல் பற்றி உணர்த்துகிறது.

கூடுதல் வினாக்கள்

நிரப்புக.

1: பழமொழி நானூறு நூலின் ஆசிரியர் முன்றுரை அரையனார்.
2. முன்றுறை அரையனாரின் காலம் கி.பி. நான்காம் நூற்றாண்டு.
3. முன்றுறை அரையனார் சமண சமயத்தைச் சேர்ந்தவர்.
4. பழமொழி நானூறு பதினெண்கீழ்க்கணக்கு நூல்களுள் ஒன்று.
5. நமது பாடப்பகுதியில் உள்ள பழமொழி நானூறு பாடலில் உள்ள பழமொழி ஒன்றாகு முன்றிலோ இல்

விடையளி :

Question 1.
பழமொழி நானூறு குறிப்பு வரைக.
Answer:

• பழமொழி நானூறு பதினெண்கீழ்க்கணக்கு நூல்களுள் ஒன்று.
• இது நானூறு பாடல்களைக் கொண்டது.
• ஒவ்வொரு பாடலின் இறுதியிலும் ஒரு பழமொழி இடம் பெற்றிருப்பதால் இது பழமொழி நானூறு என்னும் பெயர் பெற்றது.

Question 2.
முன்றுறை அரையனார் – குறிப்பு வரைக.
Answer:

• பழமொழி நானூறு நூலின் ஆசிரியர் முன்றுரை அரையனார் ஆவார்.
• இவர் கி.பி. நான்காம் நூற்றாண்டைச் சேர்ந்தவர் என்பர்.
• பழமொழி நானூறு நூலின் கடவுள் வாழ்த்துப் பாடல் மூலம் இவர் சமண சமயத்தைச்  சேர்ந்தவர் என அறிய முடிகிறது.

பாடலின் பொருள்

மழையின்றி வறட்சி நிலவிய காலத்தில், பாரி மகளிரான அங்கவை, சங்கவை ஆகியோரிடம் பாணர்கள் இரந்து நின்றனர். பாரிமகளிர் உலைநீரில் பொன் இட்டு அவர்களுக்குத் தந்தனர். அதனால் பொருள் ஏதும் இல்லாத வீடு எதுவும் இல்லை என்பதை அறியலாம்.

இப்பாடலில் இடம் பெற்றுள்ள பழமொழி ஒன்றாகு முன்றிலோ இல் என்பதாகும். ஒன்றுமில்லாத வீடு எதுவுமில்லை என்பது இதன் பொருள்.

Students can Download Tamil Chapter 1.2 வயலும் வாழ்வும் Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 1.2 வயலும் வாழ்வும்

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
உழவர் சேற்று வயலில் ………………………. நடுவர்.
அ) செடி
ஆ) பயிர்
இ) மரம்
ஈ) நாற்று
Answer:
ஈ) நாற்று

Question 2.
வயலில் விளைந்து முற்றிய நெற்பயிர்களை …………………… செய்வர்.
அ) அறுவடை
ஆ) உழவு
இ) நடவு
ஈ) விற்பனை
Answer:
அ) அறுவடை

Question 3.
தேர்ந்தெடுத்து’ என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது ……….
அ) தேர் + எடுத்து
ஆ) தேர்ந்து + தெடுத்து
இ) தேர்ந்தது + அடுத்து
ஈ) தேர்ந்து + எடுத்து
Answer:
ஈ) தேர்ந்து + எடுத்து

Question 4.
‘ஓடை + எல்லாம்’ என்பதனைச் சேர்த்தெழுதக் கிடைக்கும் சொல் ……………
அ) ஓடை எல்லாம்
ஆ) ஓடையெல்லாம்
இ) ஓட்டையெல்லாம்
ஈ) ஓடெல்லாம்
Answer:
ஆ) ஓடையெல்லாம்

பொருத்துக

வயலும் வாழ்வும் பாடலில் உள்ள மோனை, எதுகைச் சொற்களை எழுதுக

Answer:

பேச்சு வழக்குச் சொற்களை எழுத்து வழக்கில் எழுதுக
எ.கா. : போயி – போய்

குறுவினா

Question 1.
உழவர்கள் எப்போது நண்டு பிடித்தனர்?
Answer:

• உழவர்கள், நாற்றுப் பறிக்கும் பொழுது அங்குள்ள நண்டுகளையும் சேர்த்துப் பிடித்தனர்.
• கதிரடித்த நெல்தாள்களை மாடுகளைக் கொண்டு மிதிக்கச் செய்து நெற்கதிர்களில் இருந்து நெல்மணிகளைப் பிரித்தெடுத்தனர்.

Question 2.
நெற்கதிரிலிருந்து நெல்மணியை எவ்வாறு பிரிப்பர்?
Answer:

• அறுவடை செய்த நெற்கதிர்களைக் களத்தில் அடித்து நெல்லைப் பிரிப்பர்.
• நெல்தாளில் எஞ்சியிருக்கும் நெல்மணிகளைப் பிரிப்பதற்காக மாடுகளைக் கொண்டு மிதிக்கச் செய்து பிரித்தெடுப்பர்.

சிறுவினா

உழவுத்தொழிலின் நிகழ்வுகளை வரிசைப்படுத்தி எழுதுக.
Answer:
உழவுத்தொழிலின் நிகழ்வுகள் :

• மாடுகளை ஏரில் பூட்டி நிலத்தை உழுது பண்படுத்துவர்.
• நாற்றங்காலில் இருந்து பிடுங்கி எடுக்கப்பட்ட நாற்றை நடுவர். அவ்வாறு நடும்போது ஒரு சாணுக்கு ஒரு நாற்று வீதம் என்று பெண்கள் நாற்றை நடுவர்.
• பயிருக்குத் தேவையான அளவு நீரைப் பாய்ச்சுவர்.
• பிறகு பயிர்களுக்கு இடையே வளர்ந்துள்ள களைகளைப் பறிப்பர்.
• பயிர்களுக்குத் தேவைக்கேற்ப பூச்சிக்கொல்லி மருந்துகளைத் தெளிப்பர். இயற்கை உரங்களையும் பயன்படுத்துவர்.
• நெற்பயிர் வளர்ந்து நெல் முற்றியதும் அறுவடை செய்வர்.
• அறுவடை செய்த நெல்தாள்களைக் கட்டுகளாகக் கட்டி நெற்களத்தில் சேர்ப்பர்.
• கதிரடித்து நெல்மணியைப் பிரித்து எடுப்பர். எஞ்சியிருக்கும் நெல்மணிகளைப் பிரிப்பதற்காக மாடுகளைக் கொண்டு மிதிக்கச் செய்வர்.
• பிறகு நெல்மணிகளை வெயிலில் காயவைத்து மூட்டைகளாகக் கட்டி வீட்டில் கொண்டு வந்து சேர்ப்பர்.

சிந்தனை வினா

உழவுத்தொழிலில் காலந்தோறும் ஏற்பட்டுவரும் மாற்றங்கள் பற்றி எழுதுக.
Answer:
உழவுத்தொழிலில் காலந்தோறும் ஏற்பட்டு வரும் மாற்றங்கள் :
(i) பண்டைத் தமிழர்கள் வேளாண்மைத் தொழிலை மிகச் சிறப்பாகச் செய்துள்ளனர்.

(ii) குறிஞ்சி நில மக்கள் ஏர்கொண்டு உழாமல் வேளாண்மை செய்துள்ளனர்.

(iii) முல்லை நில மக்கள்தான் கலப்பையின் உதவியோடு பயிர் செய்துள்ளனர்.

(iv) ஆறு, குளம், ஏரி முதலிய நீர் நிலைகளில் இருந்து உழவர்கள் வயலுக்கு நீர் பாய்ச்சினர். பயிர் செய்தனர். வரகு, சாமை, நெல் எனப் பயிர் செய்தனர் தமிழர். அரிசியை உலகிற்கெல்லாம் கொடுத்தனர்.

(v) அதேபோல பிற பகுதிகளில் இருந்து வேறு சில பயிர்களைத் தமிழகத்திற்குப் பண்டைத் தமிழர்கள் கொண்டு வந்துள்ளனர்.

(vi) வானியல் பற்றி அறிந்ததால் கோள்களின் நிலை கண்டு மழை வரும் காலமறிந்து பயிர் செய்தனர். இயற்கை உரங்களான இலைதழைகளைப் பயன்படுத்தினர். கால்நடைகளை வளர்த்து அதன் சாணங்களை எருவாக்கினர். கால்நடைகளை வண்டி இழுக்கவும் ஏர் உழவும் பயன்படுத்தினர்.

(vii) இந்நிலை கொஞ்சம் கொஞ்சமாக மாறியது அதிக விளைச்சல் வேண்டி செயற்கை உரங்களைப் பயன்படுத்தினர். பலவகையான பூச்சிக்கொல்லி மருந்துகளைப் பயன்படுத்தினர். இதனால் மண் வளம் பாதிப்புக்குள்ளாகி விட்டது. மனிதன் செய்த வேலைகளையெல்லாம் தற்பொழுது இயந்திரங்கள் செய்கின்றன.

(viii) உழவுக் கருவிகள், களைப்பறித்தல், அறுவடை செய்தல் என எல்லாவற்றிற்கும் நவீன இயந்திரங்கள் பயன்படுத்தப்படுகின்றன. புதிய வகை வேளாண்மையின் விளைவாக மக்களுக்குப் புதிய நோய்கள் வந்தன. சுற்றுச்சூழல் பாதிக்கப்பட்டது. இந்நிலையை மாற்ற வேண்டி தற்போது இயற்கை வேளாண்மை என்று கூறப்படும் அங்கக வேளாண்மைக்கு மாறியுள்ளனர் விவசாயிகள். இவ்வாறு உழவுத்தொழில் காலந்தோறும் மாற்றமடைந்து கொண்டே வந்துள்ளது.

கற்பவை கற்றபின்

Question 1.
வேளாண்மை சார்ந்த கருவிகளின் பெயர்களை எழுதி வருக.
Answer:
வேளாண்மை சார்ந்த கருவிகள் : ஏர்க்கலப்பை, மண்வெட்டி, அரிவாள், கத்தி, உழவு இயந்திரம், கடப்பாரை.

தெரிந்து தெளிவோம்

அறுவடை செய்த நெற்கதிர்களைக் களத்தில் அடித்து நெல்லைப் பிரிப்பர். நெல்தாளில் எஞ்சியிருக்கும் நெல்மணிகளைப் பிரிப்பதற்காக மாடுகளைக் கொண்டு மிதிக்கச் செய்வர். இதற்குப் போரடித்தல் என்று பெயர்.

மாடுகட்டிப் போரடித்தால் மாளாது செந்நெல்லென்று ஆனைகட்டிப் போரடிக்கும் அழகான தென்மதுரை – நாட்டுப்புறப்பாடல்

கூடுதல் வினாக்கள்

நிரப்புக.

1. நாட்டுப்புறப் பாடல்களை மலை அருவி என்னும் நூலில் கி.வா.ஜகந்நாதன் தொகுத்துள்ளார்.
2. ‘குழி’ என்பது நில அளவைப் பெயர்.
3. சாண் என்பது நீட்டல் அளவைப் பெயர்.
4. நெற்பயிர் நடுவதற்கான இடைவெளி ஒரு சாண்.

விடையணி:

Question 1.
வாய்மொழி இலக்கியம் – குறிப்பு வரைக.
Answer:
நாட்டுப்புறங்களில் உழைக்கும் மக்கள் தங்கள் களைப்புத் தெரியாமல் இருப்பதற்காகப் பாடும் பாடலே நாட்டுப்புறப் பாடல் எனப்படுகிறது. இதனை வாய்மொழி இலக்கியம் என்றும் வழங்குவர்.

Question 2.
போரடித்தல் என்றால் என்ன?
Answer:

• அறுவடை செய்த நெற்கதிர்களைக் களத்தில் அடித்து நெல்லைப் பிரிப்பர்.
• நெல்தாளில் எஞ்சியிருக்கும் நெல்மணிகளைப் பிரிப்பதற்காக மாடுகளைக் கொண்டு மிதிக்கச் செய்வர். இதற்குப் போரடித்தல் என்று பெயர்.

Question 3.
போரடித்தல் பற்றிப் பாடும் நாட்டுப்புறப் பாடலை எழுதுக.
Answer:
“மாடு கட்டிப் போரடித்தால் மாளாது செந்நெல்லென்று
ஆனைகட்டிப் போரடிக்கும் அழகான தென்மதுரை
என்ற பாடல் போரடித்தல் பற்றிக் குறிப்பிடுகிறது.

Question 4.
சும்மாடு என்றால் என்ன?
Answer:
பாரம் சுமப்பவர்கள் தலையில் வைத்துக் கொள்ளும் துணிச்சுருள் சும்மாடு எனப்படும்.

Question 5.
நாற்றுப் பறிக்கும்போது ஆண்களும் பெண்களும் என்ன செய்தனர்?
Answer:
நாற்றுப் பறிக்கும் போது ஆண்களும் பெண்களும் வயல் வரப்பில் உள்ள நண்டுகளையும் பிடித்தனர்.

பாடலின் பொருள்

உழவு செய்யும் மக்கள் ஓடையைக் கடந்து சென்று ஒன்றரைக் குழி நிலத்தைத் தேர்ந்தெடுத்தனர். பெண்கள் புடவையை இறுகக் கட்டி நடவு செய்ய வயலில் இறங்கினர். நாற்று பறிக்கும் போது ஆண்களும் பெண்களும் வயல் வரப்பில் உள்ள நண்டுகளையும் பிடித்தனர்.

ஒரு சாணுக்கு ஒரு நாற்று வீதம் சுறுசுறுப்பாக நட்டனர். நடவு நட்ட வயலின் மண்குளிருமாறு மடைவழியே நீர்பாய்ச்சினர். நட்ட நெற்பயிர்கள் வரிசையாக வளர்ந்து செழித்தன. பால் பிடித்து முற்றிய நெல்மணிகள் மனம் மயங்குமாறு விளைந்தன. அறுவடை செய்யும் ஆட்களுக்குப் பணம் கொடுத்தனர்.

அறுவடை செய்த நெல்தாள்களைக் கட்டுகளாகக் கட்டித் தலைக்குச் சும்மாடு வைத்துத் தூக்கிச் சென்று களத்தில் சேர்த்தனர். கதிரடித்த நெல்தாள்களைக் கிழக்கத்தி மாடுகளைக் கொண்டு மிதிக்கச் செய்தனர். மாடுகள் மிதித்த நெற்கதிர்களில் இருந்து நெல்மணிகள் மணிமணியாய் உதிர்ந்தன

Students can Download Tamil Chapter 3.4 தமிழ் ஒளிர் இடங்கள் Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 2 Chapter 3.4 தமிழ் ஒளிர் இடங்கள்

மதிப்பீடு

Question 1.
நீங்கள் சுற்றுலா வழிகாட்டியாக இருந்தால், சுற்றுலாக் கையேடு என்னும் பகுதியில் உள்ள இடங்களைப் பார்வையிட வருபவர்களுக்கு எவ்வாறு விளக்கிக் கூறுவீர்கள்?
Answer:
நான் சுற்றுலா வழிகாட்டியாக இருந்தால், சுற்றுலாக் கையேடு என்னும் பகுதியில் உள்ள இடங்களைப் பார்வையிட வருபவர்களுக்கு இவ்வாறு விளக்கி கூறுவேன்.

தஞ்சை சரசுவதி மகால் நூலகம் :

• இதுதான் தஞ்சை சரசுவதி மகால் நூலகம்.
• இந்தியாவில் உள்ள தொன்மையான நூலகங்களுள் ஒன்று.
• கி.பி. 1122 முதல் இயங்கி வருகிறது என்று கல்வெட்டுச் செய்திகள் கூறுகின்றன.
• இங்குத் தமிழ், தெலுங்கு உள்ளிட்ட பல்வேறு மொழிகளின் ஓலைச் சுவடிகளும் கையெழுத்துப் படிகளும் உள்ளன.
• தலை சிறந்த ஓவியங்களும் தொன்மையான இசைக்கருவிகளும் இடம்பெற்றுள்ளன.

தமிழ்ப் பல்கலைக்கழகம் தஞ்சாவூர் :

• இப்பல்கலைக்கழகம் கி.பி. 1981 இல் தமிழக அரசால் தோற்றுவிக்கப்பட்டது.
• இதன் பரப்பளவு ஆயிரம் ஏக்கர்.
• வானத்தில் இருந்து பார்த்தால், தமிழ்நாடு’ என்ற எழுத்துகள் தெரியும் வகையில் இதன் கட்டட அமைப்பு உள்ளது.
• இந்திய நாகரித்தின் பண்பாட்டுக் கூறுகளை ஆராய்வதே இதன் நோக்கம்.
• இங்குக் கலை, சுவடி, வளர்தமிழ், மொழி, அறிவியல் என ஐந்து புலங்களும் இருபத்தைந்து துறைகளும் உள்ளன.
• இங்குச் சித்த மருத்துவத்துறை மூலம் மருத்துவத் தொண்டு செய்யப்படுகிறது.
• இந்திய ஆட்சிப் பணி பயிற்சியாளர்களுக்குத் தமிழ் மொழிப் பயிற்சி அளிக்கிறது.
• பல்வேறு நாடுகளைச் சேர்ந்த ஏராளமான மாணவர்கள் கல்வி கற்று வருகின்றனர்.

உ.வே.சா. நூலகம் – சென்னை :

• இந்நூலகம் கி.பி. 1942 இல் தொடங்கப்பட்டது.
• தமிழ், தெலுங்கு , வடமொழி உள்ளிட்ட பல்வேறு மொழி நூல்கள் உள்ளன.
• 2128 ஓலைச்சுவடிகளும் 2941 தமிழ் நூல்களும் உள்ளன.

கீழ்த்திசை நூலகம் – சென்னை

• இந்நூலகம் கி.பி. 1869ஆம் ஆண்டு தொடங்கப்பட்டது.
• இங்குத் தமிழ், தெலுங்கு, கன்னடம், மராத்தி உள்ளிட்ட பல்வேறு மொழிகளின் ஓலைச்சுவடிகள் உள்ளன.
• கணிதம், வானியல், மருத்துவம், வரலாறு உள்ளிட்ட பல்வேறு துறை நூல்களும் உள்ளன.
• தற்போது அண்ணா நூற்றாண்டு நூலகத்தின் ஏழாம் தளத்தில் இயங்கி வருகிறது.

கன்னிமாரா நூலகம் – சென்னை :

• இது கி.பி. 1896இல் தொடங்கப்பட்டது.
• தமிழ்நாட்டின் மைய நூலகம்.
• இந்திய நாட்டின் களஞ்சிய நூலகங்களில் ஒன்று.
• ஆறு இலட்சத்திற்கும் மேற்பட்ட நூல்கள் உள்ளன.
• இந்தியாவில் வெளியிடப்படும் புத்தகங்கள், நாளிதழ்கள், பருவ இதழ்கள் ஆகியவற்றின் ஒரு பிரதி இங்குப் பாதுகாக்கப்படுகிறது.
• மூன்றாம் தளத்தில் மறைமலை அடிகள் நூலகம் செயல்பட்டு வருகின்றது.

வள்ளுவர் கோட்டம் – சென்னை :

• இது திருவள்ளுவரின் புகழை உலகறியச் செய்கிறது.
• இதன் கட்டுமானப் பணிகள் கி.பி. 1973 இல் தொடங்கி 1976 இல் முடிக்கப்பட்டன.
• இதன் வடிவம் திருவாரூர் தேர் போன்று இருக்கும்.
• இரண்டு யானைகள் இழுத்துச் செல்வது போன்று கருங்கற்களால் உருவாக்கப்பட்டுள்ளது.
• இதன் அடிப்பகுதி இருபத்தைந்து அடி அகலமும் இருபத்தைந்து அடி நீளமும் உடையது.
• தேரின் மொத்த உயரம் 128 அடி.
• நான்கு சக்கரங்கள் தனிக் கற்களால் அமைக்கப்பட்டுள்ளன.
• கடைக்கோடி இரண்டு சக்கரங்கள் பெரியனவாகவும் நடுவில் இரண்டு சக்கரங்கள் சிறியனவாகவும் உள்ளன.
•  தேரின் மையத்தில் உள்ள எண்கோண வடிவக் கருவறையில் திருவள்ளுவரின் சிலை கவினுற உள்ளது. இங்கு நிகழ்ச்சிகள் நடத்துவதற்கான அரங்கம் ஒன்றும் உள்ளது.
• 1330 குறட்பாக்களும் செதுக்கப்பட்டுள்ளன.
• கருநிறப் பளிங்குக் கல்லில் அறத்துப்பால், வெண்ணிறப் பளிங்குக் கல்லில் பொருட்பால், செந்நிறப் பளிங்குக் கல்லில் இன்பத்துப்பால் எனப் பொறிக்கப்பட்டுள்ளன.
• இங்குள்ள ஓவியங்கள் திருக்குறள் கருத்துகளை விளக்குகின்றன.

திருவள்ளுவர் சிலை – கன்னியாகுமரி :

• இதுதான் திருவள்ளுவரின் சிலை.
• விவேகானந்தர் பாறைக்கு அருகில், கடல் நடுவே நீர் மட்டத்திலிருந்து முப்பது அடி உயரப் பாறை மீது இச்சிலை அமைக்கப்பட்டுள்ளது.
• இப்பணி 1990 ஆம் ஆண்டு தொடங்கியது. பொதுமக்கள் பார்வைக்காக 2000ஆம் ஆண்டு சனவரித் திங்கள் முதல் நாள் அன்று திறந்து வைக்கப்பட்டது.
• பாறையிலிருந்து சிலையின் உயரம் மொத்தம் 133 அடி. இது திருக்குறளின் மொத்த அதிகாரங்களைக் குறிக்கிறது.
• இச்சிலை அமைப்பதற்கு மூன்று டன் முதல் எட்டு டன் வரை எடை உள்ள 3,681 கருங்கற்கள் பயன்படுத்தப்பட்டுள்ளன.
• தமிழின் பெருமைமிகு அடையாளமாக இச்சிலை உயர்ந்து நிற்கிறது.

உலகத் தமிழ்ச் சங்கம் – மதுரை :

• இதுதான் உலகத் தமிழ்ச் சங்கம்.
• இது சுமார் எண்பத்தேழு ஆயிரம் சதுர அடி பரப்பளவில் மனத்தைக் கவரும் வகையில் கட்டப்பட்டுள்ளது.
• இதனுள் பன்னாட்டு அளவிலான கருத்தரங்கக் கூடங்கள், ஆய்வரங்கங்கள், நூலகம், பார்வையாளர் அரங்கம் ஆகியன கவினுற அமைக்கப்பட்டுள்ளன. வெளிப்புறச் சுற்றுச் சுவர்களில் 1330 குறட்பாக்கள் இடம் பெற்றுள்ளன.
• இதன் சுற்றுச் சுவர்களில் சங்க இலக்கியக் காட்சிகள் வண்ண ஓவியங்களாக வரையப்பட்டுள்ளன.

சிற்பக் கலைக்கூடம் – பூம்புகார் :

• இதுதான் பூம்புகார் சிற்பக் கலைக்கூடம்.
• கி.பி.(பொ.ஆ) 1973 ஆம் ஆண்டு பூம்புகார் கடற்கரையில் சிற்பக் கலைக்கூடம் ஒன்று ஏற்படுத்தப்பட்டது.
• இக்கூடம் ஏழுநிலை மாடங்களைக் கொண்டது. கண்ணகியின் வரலாற்றை விளக்கும் நாற்பத்தொன்பது சிற்பத் தொகுதிகள் இதில் இடம்பெற்றுள்ளன. மாதவிக்கு ஒரு நெடிய சிலை இங்கு நிறுவப்பட்டுள்ளது.

கலைக்கூடத்திற்கு அருகில் இலஞ்சிமன்றம், பாவைமன்றம், நெடுங்கல் மன்றம் ஆகியன அமைந்துள்ளன. இலஞ்சி மன்றத்திலும் பாவைமன்றத்திலும் வடிவமைக்கப்பட்டுள்ள பெண்களின் உருவங்கள் நம் கண்ணையும் கருத்தையும் கவர்கின்றன. நெடுங்கல் மன்றத்தில் நெடிய கற்றூண் ஒன்றும் அதைச் சுற்றி எட்டுச் சிறிய கற்றூண்களும் எட்டு மனித உருவங்களும் தற்காலச் சிற்பக்கலைக்கு எடுத்துக்காட்டுகளாய் நிற்கின்றன.

கற்பவை கற்றபின்

Question 1.
உங்கள் மாவட்டத்திலுள்ள சுற்றுலா இடங்களின் சிறப்புகளை எழுதி வருக.
Answer:
எங்கள் மாவட்டம் திருநெல்வேலி மாவட்டம். இம்மாவட்டம் கிழக்கு இந்தியா கம்பெனியால் 1790இல் உருவாக்கப்பட்டதாகும். தாமிரபரணி ஆற்றின் கரையில் அமைந்துள்ளது.

இம்மாவட்டத்திலுள்ள சுற்றுலாத்தலங்கள் :

• குற்றாலம்
• அகத்தியர் அருவி
• முண்டந்துறை வனவிலங்கு சரணாலயம்
• கூந்தன்குளம் பறவைகள் சரணாலயம்
• மணிமுத்தாறு அணை
• நெல்லையப்பர் கோயில்
• சங்கரன் கோயில்
• காசி விஸ்வநாதர் கோயில்

குற்றாலம் :
குளிப்பதற்கு ஓர் ஊர் குற்றாலம்’ என்று சொல்வதற்கேற்ப குற்றாலத்தில் நாள் 6 முழுவதும் குளிக்கலாம். எப்பொழுதும் குளுமையாக இருக்கும் இடம் குற்றாலம். வானம் சிறு மழையைத் தூவிக் கொண்டே இருக்கும். குற்றாலச் சாரலை அனுபவிக்க ஜூன், ஜூலை மாதங்களில் மக்கள் கூட்டம் அலைமோதும்.

இங்கு மலைகள், அருவிகள் என இயற்கை வளங்கள் நிறைந்துள்ளன. மூலிகைச் செடிகள் நிறைய உள்ளன. இம்மலையில் உள்ள பலவகையான அரிய மலர்களும் அழகைச் சேர்க்கின்றன.

இம்மலையில் மா, பலா, வாழை, கொய்யா எனப் பலவகையான பழங்களும், நெல்லிக்காய், தேங்காய் போன்றவைகளும் கிடைக்கின்றன. பாக்கு, தேன், கிழங்குகள் கிடைக்கின்றன. தேக்கு, கோங்கு , சந்தனம் ஆகிய மரங்களும் உள்ளன. இவற்றிற்கெல்லாம் மணிமகுடமாய்க் குற்றாலநாதர் வீற்றிருந்து அருள்பாலிக்கிறார்.

அகத்தியர் அருவி :
அகத்தியர் அருவி மேற்குத் தொடர்ச்சி மலைத் தொடரில் அமைந்துள்ளது. இங்கு அகத்தியருக்குக் கோயில் உள்ளது. சிவனும் பார்வதியும் அகத்தியருக்கு நேரில் காட்சி தந்த இடம் என்றும் கூறப்படுகிறது.

நெல்லையப்பர் கோயில் :
திருநெல்வேலியின் சிறப்பைப் பறை சாற்றுவதே நெல்லையப்பர் – காந்திமதி கோயில் தான். தேவாரப் பாடல் பாடப் பெற்ற பாண்டிய நாட்டுத் தலங்களில் ஒன்றாகும். ஆசியாவிலேயே மிகப்பெரிய சிவாலயமாகும். இக்கோயிலில் ‘ஏழிசை ஸ்வரங்கள் இசைக்கும் தூண்கள்’ உள்ளன. இக்கோயிலில் உள்ள தேர் தமிழ்நாட்டின் மூன்றாவது மிகப்பெரிய தேர் என்ற பெருமைக்குரியது.

சங்கரன் கோயில் :
சிவனும் பெருமாளும் ஒருவராய் இணைந்திருக்கும் கோயில் என்பதால் இந்தக் கோயிலில் உள்ள மூலவர் சங்கர நாராயணனார் என்றழைக்கப்படுகிறார். இரண்டு ஆண்டுகளுக்கு ஒருமுறை இந்தச் சிலையின் திருவடிகளைக் கதிரவன் தழுவுவதாகக் கூறப்படுகிறது.

முண்டந்துறை வனவிலங்கு சரணாலயம் :
இச்சரணாலயத்தின் பரப்பளவு 567 ச.மீட்டர். இங்கு புலி, சிங்கவால் குரங்கு, கரடி, காளை, ஆடு, ஓநாய், யானை மற்றும் பலவகையான மான்கள் பாதுகாக்கப்படுகின்றன.

காசி விஸ்வநாதர் கோயில் :
வட நாட்டுக்கு ஒரு காசி இருப்பது போல் தென்னாட்டில் உள்ளது தென்காசி. இங்கு உள்ள காசி விஸ்வநாதர் ஆலயம் மிகவும் புகழ்பெற்றது. இக்கோயிலின் பிரம்மாண்டமான  கோபுரத்தை 180 அடியில் 1456 ஆம் ஆண்டு பராக்கிரம பாண்டிய மன்னன் கட்டியுள்ளார். 1924 ஆம் ஆண்டு பேரிடியினால் அக்கோபுரம் தகர்ந்து விழுந்தது. தற்போது 168 அடி உயரத்தில் மீண்டும் கட்டி முடிக்கப்பட்டுள்ளது. இக்கோயிலிலில் 1927ஆம் ஆண்டு கப்பலோட்டிய தமிழன் வ.உ.சிதம்பரநாதரால் தொடங்கப்பட்ட திருவள்ளுவர் கழகம் இன்றும் சிறப்பாகத் தமிழ்ப் பணியில் ஈடுபட்டு வருகிறது.

மணிமுத்தாறு அணை :
இது மணிமுத்தாற்றின் குறுக்கே கட்டப்பட்ட அணைகளுள் ஒன்று. இந்த ஆறு மேற்குத் தொடர்ச்சி மலையில் களக்காடு பகுதியில் பச்சையாற்றின் பிறப்பிடத்திலிருந்து தனியாகப் பிரிந்து மணிமுத்தாறு அருவியாக மணிமுத்தாறு அணைக்கட்டில் வந்து விழுகிறது. இந்த அணை முன்னாள் தமிழக முதல்வர் காமராசரால் கட்டப்பட்டது.

கூந்தன்குளம் பறவைகள் காப்பகம் :
இக்காப்பகம் திருநெல்வேலி மாவட்டத்தில் நான்குநேரி வட்டத்தில் உள்ளது. கூந்தன் குளம் கிராம மக்களின் அரவணைப்பில் பறவைகள் யாவும், மனிதர் பயம் இன்றி அனைத்து வீடுகளின் மரங்களிலும் கூடுகள் அமைத்து முட்டையிட்டு, குஞ்சுகளைப் பாதுகாத்துக் கொள்கின்றன. இங்கு பல்வேறு பகுதிகளில் இருந்து பறவைகள் வந்து செல்லும். ஆண்டுதோறும் அதிகபட்சமாக ஒரு லட்சம் பறவைகள் வருவதாகப் பதிவு செய்யப்பட்டுள்ளது.

எங்கள் திருநெல்வேலி மாவட்டம் சிறியவர், பெரியவர் என அனைவருக்கும் ஏற்றபடி மிகச் சிறந்த சுற்றுலாத்தலமாய் அமைந்துள்ளது என்பதைப் பெருமிதத்துடன் கூறலாம்.

Question 2.
நீங்கள் கண்டுகளித்த இடங்களின் தனித்தன்மைகளை எழுதுக.
Answer:
குற்றாலம் :
குற்றாலத்தில் எப்போதும் சாரல் மழை இருந்து கொண்டே இருக்கும். அதனால் எப்பொழுதும் குளுமையாக இருக்கும். குற்றாலச் சாரல் என்பது, இயற்கை இந்த ஊருக்கு அளித்த மிகப்பெரிய கொடையாகும். ஒவ்வோர் ஆண்டும் ஜூன், ஜூலை, ஆகஸ்டு மாதங்களில் இச்சாரல் மழையை அனுபவிக்க மக்கள் கூட்டம் அலை மோதும்.

இங்கு மலைகள், அருவிகள் எனப்பல இயற்கை வளங்கள் உள்ளன. காட்டுப் பகுதியும் உள்ளது. அக்காட்டுப் பகுதியில் மூலிகைச் செடிகள் ஏராளமாகக் காணப்படுகின்றன.

குற்றால அருவி, பழத்தோட்ட அருவி, புது அருவி, சிற்றருவி, ஐந்தருவி, புலி அருவி, பேரருவி ஆகிய ஏழு அருவிகள் குற்றாலத்தில் உள்ள மலைகளில் இருந்து நிலம் நோக்கி விழும் அருவிகள் ஆகும். இவை காண்பதற்குக் கண்கொள்ளாக் காட்சியாகும்.

அருவிகளின் அணைப்பிலே உள்ள குற்றாலநாதர் கோயில் மிகவும் புகழ்பெற்றது. இது சங்கு வடிவில் அமைக்கப்பட்டுள்ள கோயில் ஆகும்.

அகத்திய முனிவர் இம்மலைக்கு வந்திருந்து, தமிழை வளர்த்தார் என்றும் கூறுவர். குற்றால நாதரைப் பாட்டுடைத் தலைவராக்கித் திரிகூட ராசப்பக் கவிராயர் குற்றாலக் குறவஞ்சி என்னும் சிற்றிலக்கியத்தை இயற்றியுள்ளார்.

சிதம்பரம், மதுரை, திருவாலங்காடு, திருநெல்வேலி, குற்றாலம் ஆகிய ஐந்து இடங்களிலும் உள்ள சிவாலயங்களில் நடராஜர் தன் நடனத்தால் சிறப்பித்த சபைகள் உள்ளன.

சிதம்பரத்தில் உள்ளது பொற்சபை, மதுரையில் உள்ளது வெள்ளி சபை, திருவாலங்காட்டில் உள்ளது ரத்தின சபை, திருநெல்வேலியில் உள்ளது தாமிர சபை, குற்றாலத்தில் உள்ளது சித்திர சபை ஆகும்.
இவ்வளவு சிறப்புகளையும் தனித்தன்மைகளையும் கொண்டது நான் கண்டுகளித்த குற்றாலம்.

Students can Download Tamil Chapter 2.2 அறம் என்னும் கதிர் Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 2.2 அறம் என்னும் கதிர்

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
காந்தியடிகள் எப்போதும் ……………………..ப் பேசினார்.
அ) வன்சொற்களை
ஆ) அரசியலை
இ) கதைகளை
ஈ) வாய்மையை
Answer:
ஈ) வாய்மையை

Question 2.
இன்சொல்’ என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது …………..
அ) இனிய + சொல்
ஆ) இன்மை + சொல்
இ) இனிமை + சொல்
ஈ) இன் + சொல்
Answer:
இ) இனிமை + சொல்

Question 3.
அறம் + கதிர் என்பதனைச் சேர்த்தெழுதக் கிடைக்கும் சொல் …….
அ) அற கதிர்
ஆ) அறுகதிர்
இ) அறக்கதிர்
ஈ) அறம்கதிர்
Answer:
இ) அறக்கதிர்

Question 4.
‘இளமை’ என்னும் சொல்லின் எதிர்ச்சொல் ……..
அ) முதுமை
ஆ) புதுமை
இ) தனிமை
ஈ) இனிமை
Answer:
அ) முதுமை

பொருத்துக

Answer:

குறுவினா

Question 1.
அறக்கதிர் விளைய எதனை எருவாக இட வேண்டும் என முனைப்பாடியார் கூறுகிறார்?
Answer:
அறக்கதிர் விளைய எருவாக இடவேண்டியது உண்மை பேசுதல் ஆகும்.

Question 2.
நீக்கவேண்டிய களை என்று அறநெறிச்சாரம் எதனைக் குறிப்பிடுகிறது?
Answer:
கடுஞ்சொற்களை நீக்கவேண்டிய களை என்று அறநெறிச்சாரம் குறிப்பிடுகிறது.

சிறுவினா

இளம் வயதிலேயே செய்ய வேண்டிய செயல்களாக முனைப்பாடியார் கூறுவன யாவை?
Answer:
இளம் வயதிலேயே செய்ய வேண்டிய செயல்கள் :

• இனிய சொல்லையே விளைநிலமாக கொள்ள வேண்டும். அதில் ஈகை என்னும் பண்பை விதையாக விதைக்க வேண்டும்.
• வன்சொல் என்னும் களையை நீக்க வேண்டும். உண்மை பேசுதல் என்னும் எருவினை இட வேண்டும்.
• அன்பாகிய நீரைப் பாய்ச்ச வேண்டும். அப்போதுதான் அறமாகிய கதிரைப் பயனாக பெற முடியும். இளம் வயதிலேயே இச்செயல்களைச் செய்ய வேண்டும் என முனைப்பாடியார் கூறுகிறார்.

சிந்தனை வினா

இளம் வயதிலேயே நாம் கற்றுக்கொள்ள வேண்டிய நற்பண்புகள் எவை எனக் கருதுகிறீர்கள்?
Answer:
இளம் வயதிலேயே நாம் கற்றுக்கொள்ள வேண்டிய நற்பண்புகள் :

கற்பவை கற்றபின்

Question 1.
பிறருடன் பேசும்போது நீங்கள் பயன்படுத்தும் இன்சொற்களைத் தொகுத்துக் கூறுக.
Answer:
பிறருடன் பேசும்போது நாங்கள் பயன்படுத்தும் இன்சொற்கள் :
இன்சொல் என்பது உதட்டளவில் தோன்றி வருவதல்ல. உள்ளத்திலிருந்து அன்புடன் தோன்றும். அச்சொல் மகிழ்ச்சியளிக்கும். இன்சொல் பேசுவது ஈகையைவிடச் சாலச் சிறந்தது. அறம் வளர்ந்த உள்ளத்திலே இனிய சொற்கள் பிறக்கும்.

நம்மைவிட வயதில் மூத்தவர்களிடம் பேசும்போது மிக்க மரியாதையுடன் பேசுவேன். இது ஒரு கடமை மட்டுமல்ல, அது ஒரு நல்லொழுக்கம் ஆகும். எவரையும் நீ, வா, போ என்று ஒருமையில் அழைப்பதைத் தவிர்ப்பேன். நீங்கள், வாருங்கள் என்று மரியாதைப் பன்மையுடன் தான் பேசுவேன். பிறரை மதிப்பதால் நான் எப்பொழுதும் தாழ்ந்து போக மாட்டேன் என்பதை நான் உணர்வேன்.

யாரையும் இகழ்ந்து பேசமாட்டேன். பேச்சில் வன்முறையைப் பயன்படுத்தமாட்டேன். எவரைப் பற்றியும் புறங்கூறமாட்டேன். பிறரை ஏளனப்படுத்திப் பேச மாட்டேன். அகந்தையுடன் பேசமாட்டேன். பிறருடைய மனம் புண்படாத வகையில் பேசுவேன்.
இவைபோன்ற சொற்களை நாங்கள் பயன்படுத்துவோம்.

Question 2.
உன் அன்னை பயன்படுத்திய இன்சொல்லால் நீ மகிழ்ந்த நிகழ்வு ஒன்றைக் கூறுக.
Answer:
என் அன்னை பயன்படுத்திய இன்சொல்லால் நான் மகிழ்ந்த நிகழ்வு :
என் அன்னை எப்போதும் இன்சொற்களையேத் தான் பயன்படுத்துவார். இதுவரை என் அம்மா யாரிடமும் கோபப்பட்டு பேசியதில்லை. ஒருமுறை என் வீட்டிற்கு என் உறவினருடைய குழந்தை வந்தது. அக்குழந்தையுடன் நான் விளையாடிக் கொண்டிருந்தேன். அக்குழந்தை என் புத்தகத்தைக் கிழித்துவிட்டது. அதனால் கோபம் வந்து அக்குழந்தையை அடித்துவிட்டேன். குழந்தை அழுதது. என் அம்மா பதற்றத்துடன் “என்னவாயிற்று” என்று கேட்டபடியே வேகமாக ஓடி வந்தார். நடந்ததைக் கேட்டறிந்தார்.

என்னை அடிப்பார்கள், திட்டுவார்கள் என்று பயந்தேன். ஆனால் முதலில் குழந்தையைச் சமாதானப்படுத்தினார்கள்.

பிறகு என்னிடம் வந்து “நீ யாரிடமும் கோபப்படக்கூடாது. குழந்தைக்குப் படிக்கும் புத்தகம் என்பது தெரியுமா? குழந்தை இருக்கும் போது நீதான் உன் புத்தகத்தைக் குழந்தையின் கைக்கெட்டாமல் வைத்திருக்க வேண்டும். நம்மைவிட வயதில் சிறியவர்களாக இருந்தாலும் தவறு செய்தால் தண்டிக்கக்கூடாது. நாம் கூறுவதைப் புரிந்து கொள்ளும் 5 வயதாக இருந்தால் சொல்லிப் புரிய வைக்க வேண்டும். இல்லையெனில் தவறு நிகழாமல் இருக்க வழி செய்ய வேண்டும். எந்தப் பொருளை எங்கு வைக்க வேண்டும் என்று அறிந்து அந்தந்த இடத்தில் வைக்க வேண்டும். எல்லோரிடமும் அன்போடு பழகு. நம்மைவிடச் சிறியவர்களுடன் விட்டுக்கொடுத்து வாழ்ந்திடு, மற்றவர்களுடைய கருத்துகளை ஏற்றுக்கொள்” என்று இன்சொற்களாலேயே அறிவுரை கூறினார். அவ்வுறிவுரையை நான் என்றும் மறவேன்.

அன்றிருந்து நான் அனைவரிடமும் அன்போடு பழகுகிறேன். அந்நாளை என்னால் மறக்கவியலாது.

கூடுதல் வினாக்கள்

நீரப்புக.

1. முனைப்பாடியார் சமண சமயத்தைச் சார்ந்தவர்.
2. முனைப்பாடியார் வாழ்ந்த காலம் பதின்மூன்றாம் நூற்றாண்டு.
3. அறநெறிச்சாரம் அறநெறிகளைத் தொகுத்துக் கூறுகிறது.

விடையளி :

Question 1.
முனைப்பாடியார் குறிப்பு எழுதுக
Answer:

• முனைப்பாடியார் திருமுனைப்பாடி என்னும் ஊரைச் சேர்ந்த சமணப் புலவர்.
• இவரது காலம் பதின்மூன்றாம் நூற்றாண்டு.
• இவர் 225 பாடல்களைக் கொண்ட அறநெறிச்சாரம் என்ற நூலை இயற்றியுள்ளார்.

Question 2.
முனைப்பாடியார் விளைநிலம் என்றும் வித்து என்றும் எவற்றைக் கூறுகிறார்?
Answer:
இனிய சொல்லை விளைநிலமாகவும், ஈகை என்னும் பண்பை விதையாகவும் முனைப்பாடியார் கூறுகிறார்.

பாடலின் பொருள்

இனிய சொல்லையே விளைநிலமாகக் கொள்ள வேண்டும். அதில் ஈகை என்னும் பண்பை விதையாக விதைக்க வேண்டும். வன்சொல் என்னும் களையை நீக்க வேண்டும். உண்மை பேசுதல் என்னும் எருவினை இட வேண்டும். அன்பாகிய நீரைப் பாய்ச்ச வேண்டும். அப்போதுதான் அறமாகிய கதிரைப் பயனாகப் பெற முடியும். இளம் வயதிலேயே இச்செயல்களைச் செய்ய வேண்டும்.

Students can Download Tamil Chapter 2.1 புதுமை விளக்கு Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 2.1 புதுமை விளக்கு

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
“இடர் ஆழி நீங்குகவே – இத்தொடரில் அடிக்கோடிட்ட சொல்லின் பொருள் ………………….
அ) துன்பம்
ஆ) மகிழ்ச்சி
இ) ஆர்வம்
ஈ) இன்பம்
Answer:
அ) துன்பம்

Question 2.
ஞானச்சுடர்’ என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது ………………….
அ) ஞான + சுடர்
ஆ) ஞானச் + சுடர்
இ) ஞானம் + சுடர்
ஈ) ஞானி + சுடர்
Answer:
இ) ஞானம் + சுடர்

Question 3.
இன்பு உருகு என்பதனைச் சேர்த்தெழுதக் கிடைக்கும் சொல் ………………….
அ) இன்பு உருகு
ஆ) இன்பும் உருகு
இ) இன்புருகு
ஈ) இன்பருகு
Answer:
இ) இன்புருகு

பொருத்துக

அன்பு – நெய்
ஆர்வம் – தகனி
சிந்தை – விளக்கு
ஞானம் – இடுதிரி
Answer:

குறுவினா

Question 1.
பொய்கையாழ்வாரும் பூதத்தாழ்வாரும் அகல்விளக்காக உருவகப்படுத்துகின்றனர்?
Answer:
பொய்கையாழ்வார் பூமியை அகல்விளக்காக உருவகப்படுத்துகின்றார். பூதத்தாழ்வார் அன்பை அகல்விளக்காக உருவகப்படுத்துகின்றார்.

Question 2.
பொய்கை ஆழ்வார் தற்காகப் பாமாலை சூட்டுகிறார் ?
Answer:
பொய்கையாழ்வார் தம்முடைய கடல் போன்ற துன்பம் நீங்குவதற்காகத் திருமாலுக்குப் பாமாலை சூட்டுகிறார்.

சிறுவினா

பூதத்தாழ்வார் ஞானவிளக்கு ஏற்றும் முறையை விளக்குக.
Answer:
பூதத்தாழ்வார் ஞான விளக்கேற்றும் முறை:
(i) விளக்கேற்றுவதற்கு அகல்விளக்கு, நெய், திரி ஆகியவை தேவை
(ii) இங்கு , பூதத்தாழ்வார் தம் உள்ளத்தில் எழுகின்ற அன்பை அகல்விளக்காவும்,
ஆர்வத்தை நெய்யாகவும், இனிமையால் உருகும் மனத்தையே இடுகின்ற திரியாகவும் கொண்டு ஞான ஒளியாகிய சுடர்விளக்கை மனம் உருக திருமாலுக்கு ஏற்றுகிறார்.

சிந்தனை வினா

பொய்கையாழ்வார் ஞானத்தை விளக்காக உருவகப்படுத்துகிறார். நீங்கள் எவற்றை எல்லாம் விளக்காக உருவகப்படுத்துவீர்கள்
Answer:
பொய்கையாழ்வார் ஞானத்தை விளக்காக உருவகப்படுத்துகிறார். நான் ஒழுக்கம், அன்பு ஆகியவற்றை விளக்காக உருவகப்படுத்துவேன்.

கற்பவை கற்றபின்

Question 1.
பன்னிரு ஆழ்வார்களின் பெயர்களைத் திரட்டுக.
Answer:

1. பொய்கை ஆழ்வார்
2. பூதத்தாழ்வார்
3. பேயாழ்வார்
4. திருமழிசை ஆழ்வார்
5. நம்மாழ்வார்
6. திருமங்கையாழ்வார்
7. தொண்டரடிப் பொடி ஆழ்வார்
8. பெரியாழ்வார்
9. ஸ்ரீ ஆண்டாள்
10. குலசேகர ஆழ்வார்
11. மதுரகவி ஆழ்வார்
12. திருப்பாணாழ்வார்

தெரிந்து தெளிவோம்

(i) ஒரு பாடலின் இறுதி எழுத்தோ அசையோ, சொல்லோ அடுத்து வரும் பாடலுக்கு முதலாக அமைவதை அந்தாதி என்பர். (அந்தம் – முடிவு, ஆதி – முதல்). இவ்வாறு அந்தாதியாக அமையும் பாடல்களைக் கொண்டு அமைவது அந்தாதி என்னும் சிற்றிலக்கிய வகையாகும்.

(ii) திருமாலைப் போற்றிப் பாடியவர்கள் பன்னிரு ஆழ்வார்கள். அவர்கள் பாடிய பாடல்களின் தொகுப்பு நாலாயிரத் திவ்விய பிரபந்தம் ஆகும். இதனைத் தொகுத்தவர் நாதமுனி ஆவார். பன்னிரு ஆழ்வார்களுள் பொய்கை யாழ்வார், பூதத்தாழ்வார், பேயாழ்வார் ஆகிய மூவரையும் முதலாழ்வார்கள் என்பர்.

கூடுதல் வினாக்கள்

நிரப்புக

1. உள்ளத் தூய்மையோடு நன்னெறியில் நடப்பதே சான்றோரின் இயல்பு.
2. பொய்கையாழ்வார் பிறந்த ஊர் காஞ்சிபுரத்திற்கு அருகில் உள்ள திருவெஃகா.
3. பொய்கையாழ்வார் நாலாயிரத் திவ்வியப் பிரபந்தத்தில் உள்ள முதல் திருவந்தாதியைப் பாடியுள்ளார்.
4. பூதத்தாழ்வார் பிறந்த ஊர் மாமல்லபுரம்.
5. நாலாயிரத்திவ்வியப் பிரபந்ததில் இரண்டாம் திருவந்தாதியைப் பாடியவர் பூதத்தாழ்வார்.
6. முதலாழ்வார்கள் பொய்கையாழ்வார், பூதத்தாழ்வார், பேயாழ்வார்.
7. திருமாலைப் போற்றிப் பாடியவர்கள் பன்னிரு ஆழ்வார்கள்.
8. ஆழ்வார்கள் மொத்தம் பன்னிருவர்.
9. ஆழ்வார்கள் பாடிய பாடல்களின் தொகுப்பு நாலாயிரத் திவ்விய பிரபந்தம்.
10. நாலாயிரத் திவ்விய பிரபந்தத்தைத் தொகுத்தவர் நாதமுனி.
11. பொய்கையாழ்வார் கடலை நெய்யாகவும், பூதத்தாழ்வார் ஆர்வத்தை நெய்யாகவும் பாடியுள்ளார்.
12. அன்பை அகல்விளக்காகப் பாடியவர் பூதத்தாழ்வார்.
13. பூமியை அகல்விளக்காகப் பாடியவர் பொய்கையாழ்வார்.

விடையளி :

Question 1.
அந்தாதி – குறிப்பு எழுதுக
Answer:

• ஒரு பாடலின் இறுதி எழுத்தோ , அசையோ, சொல்லோ அடுத்து வரும் பாடலுக்கு முதலாக அமைவதை அந்தாதி என்பர்.
• இவ்வாறு அந்தாதியாக அமையும் பாடல்களைக் கொண்டு அமைவது அந்தாதி என்னும் சிற்றிலக்கிய வகையாகும்.

Question 2.
பொய்கையாழ்வார் குறிப்பு எழுதுக.
Answer:

• பொய்கையாழ்வார் காஞ்சிபுரத்திற்கு அருகிலுள்ள திருவெஃகா என்னும் ஊரில் பிறந்தவர்.
• நாலாயிரத்திவ்வியப் பிரபந்தத்தில் உள்ள முதல் திருவந்தாதியைப் பாடியவர்.

Question 3.
பூதத்தாழ்வார் குறிப்பு வரைக.
Answer:

• பூதத்தாழ்வார் சென்னையை அடுத்துள்ள மாமல்லபுரத்தில் பிறந்தவர்.
• இவர் நாலாயிரத் திவ்வியப் பிரபந்தத்தில் இரண்டாம் திருவந்தாதியைப் பாடியவர்.

பாடலின் பொருள்

ஞானத்தமிழ் பயின்ற நான் அன்பையே அகல்விளக்காகவும், ஆர்வத்தையே நெய்யாகவும், இனிமையால் உருகும் மனத்தையே இடுகின்ற திரியாகவும் கொண்டு, ஞான ஒளியாகிய சுடர் விளக்கை மனம் உருக திருமாலுக்கு ஏற்றினேன்.

Students can Download Tamil Chapter 2.3 ஒப்புரவு நெறி Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 2.3 ஒப்புரவு நெறி

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
ஒருவர் எல்லாருக்காகவும் எல்லாரும் ஒருவருக்காக என்பது……….. நெறி.
அ) தனியுடமை
ஆ) பொதுவுடைமை
இ) பொருளுடைமை
ஈ) ஒழுக்கமுடைமை
Answer:
ஆ) பொதுவுடைமை

Question 2.
செல்வத்தின் பயன் ……………….. வாழ்வு.
அ) ஆடம்பர
ஆ) நீண்ட
இ) ஒப்புரவு
ஈ) நோயற்ற
Answer:
இ) ஒப்புரவு

Question 3.
வறுமையைப் பிணி என்றும் செல்வத்தை …………… என்றும் கூறுவர்.
அ) மருந்து
ஆ) மருத்துவர்
இ) மருத்துவமனை
ஈ) மாத்திரை
Answer:
அ) மருந்து

Question 4.
மருந்து] உலகம் உண்ண உண்; உடுத்த உடுப்பாய் என்று கூறியவர் ………….
அ) பாரதியார்
ஆ) பாரதிதாசன்
இ) முடியரசன்
ஈ) கண்ண தாசன்
Answer:
ஆ) பாரதிதாசன்

எதிர்ச்சொற்களைப் பொருத்துக

Answer:

தொடர்களில் அமைத்து எழுதுக

1. குறிக்கோள் -…………………………………
2. கடமைகள் -…………………………………
3. வாழ்நாள் -…………………………………
4. சிந்தித்து -…………………………………
Answer:
1. குறிக்கோள் – நான் மருத்துவர் ஆகவேண்டும் என்பது என் குறிக்கோள்.
2. கடமைகள் – பெற்றோரைப் பாதுகாப்பது இன்றியமையாத கடமைகளுள் ஒன்று.
3. வாழ்நாள் – வாழ்நாள் முழுவதும் உண்மையைப் பேசி உண்மைக்கு இலக்கணம் ஆனவர் அரிச்சந்திரன்.
4. சிந்தித்து – சிந்தித்துச் செயலாற்றினால் நாம் சிறப்படையலாம்.

குறுவினா

Question 1.
பொருளீட்டுவதை விடவும் பெரிய செயல் எது?
Answer:
பொருளீட்டுவதைவிடப் பெரிய செயல் அதை முறையாக அனுபவிப்பதும், கொடுத்து மகிழ்வதும் ஆகும்.

Question 2.
பொருளீட்டுவதன் நோக்கமாகக் குன்றக்குடி அடிகளார் கூறுவது யாது?
Answer:
பொருளீட்டுவதன் நோக்கம் : மற்றவர்களுக்கு வழங்கி, மகிழ்வித்து மகிழ, வாழ்வித்து வாழப் பொருள் தேவை. இதுவே பொருளீட்டுவதன் நோக்கம் என குன்றக்குடி அடிகளார் கூறுகிறார்.

சிறுவினா

Question 1.
ஒப்புரவுக்கு அடிகளார் தரும் விளக்கம் யாது?
Answer:
ஒப்புரவு :

• ஒருவர் செய்யும் செயலானது அது தரும் பயனைவிட, செய்பவரின் மனப்பாங்கு , உணர்வு ஆகியவற்றின் அடிப்டையிலேயே மதிப்பிடப்படுகிறது. தரத்தைக் காட்டுகிறது.  ஒருவருக்கொருவர் உதவி செய்து கொள்ளுதல் மட்டும் போதாது.
• தற்காப்புக்காகவும் இலாபத்திற்காகவும் உதவி செய்யலாம். இத்தகைய உதவிகள் ஒருவகையில் வாணிகம் போலத்தான்.
•  அதே உதவியைக் கட்டுப்பாட்டு உணர்வுடன், உதவி பெறுபவரை உறவுப்பாங்கில் எண்ணி, உரிமை உடையவராக நினைந்து, உதவி செய்வதற்குப் பதில் அவரே எடுத்துக் கொள்ளும் உரிமையை வழங்குதல் ஒப்புரவு ஆகும்.

Question 2.
ஊருணியையும் மரத்தையும் எடுத்துக்காட்டிக் குன்றக்குடி அடிகளார் கூறும் செய்திகள் யாவை?
Answer:
(i) ஊருணியை அகழ்ந்து, தண்ணீரைக் கொணர்ந்து தேக்குபவர் மனிதர்தாம் ஊருணியை அமைத்துத் தண்ணீரைத் தேக்கும் கடமை பொறுப்புணர்வுடன் – கூட்டுப் பொறுப்புடன் செய்யப் பெற்றால் தான் ஊருணியில் தண்ணீர் நிறையும். பலரும் எடுத்துக் குடிக்கலாம். பயன்தரும் மரங்களை வளர்த்தால்தான் கனிகள் கிடைக்கும். தின்று அனுபவிக்கலாம்.

(ii) இங்கும் மனிதனின் படைப்பைத் தொடர்ந்துதான் நுகர்வு வருகிறது. ஒப்புரவு வருகிறது. அதேபோல மருந்து மரங்களையும் நட்டு வளர்த்தால் தான் பயன்படுத்த முடியும். ஆதலால் ஒப்புரவாண்மையுடன் வாழ முதலில் தேவைப்படுவது உழைப்பு. கூட்டு உழைப்பு. பொருள்களைப் படைக்கும் கடமைகள் நிகழாத வரையில் ஒப்புரவு வாழ்வு மலராது. கடமைகள் இயற்றப்பெறாமல் ஒப்புரவு தோன்றாது.

(iii) ஒரேவழி தோன்றினாலும் நிலைத்து நில்லாது. கடமைகளில் பொருள் செய்தலில் ஒவ்வொருவரும் கூட்டு உழைப்பில் ஈடுபட்டால்தான் ஒப்புரவு நெறி தோன்றும்; வளரும்; நிலைத்து நிற்கும்.

சிந்தனை வினா

ஒப்புரவுக்கும் உதவி செய்தலுக்கும் வேறுபாடு யாது?
Answer:

கற்பவை கற்றபின்

Question 1.
பிறருக்காக உழைத்துப் புகழ்பெற்ற சான்றோர்கள் பற்றிய செய்திகத்தினத் திரட்டி வந்து வகுப்பறையில் பகிர்க.
Answer:
பிறருக்காக உழைத்துப் புகழ்பெற்ற சான்றோர்கள் பற்றிய செய்திகள் :
(i) சகோதரி நிவேதிதா :
சகோதரி நிவேதிதா சுவாமி விவேகானந்தரின் முதன்மை சீடர். இவர் அயர்லாந்தின் வடபகுதி மாகாணம் டைரோனில் உள்ள டங்கனன் எனும் ஊரில் பிறந்தவர். பெற்றோர் சாமுவேல் ரிச்மண்ட் , மேரி இசபெல் ஹாமில்டன் ஆவர். இவருடைய இயற்பெயர் மார்கரெட் எலிசபெத் நோபிள்.

தம்முடைய பதினெழு வயதில் ஆசிரியர் பணியைத் தொடங்கினார். சிறந்த கல்வியாளராக உயர்ந்தார். ரஸ்கின் பள்ளி’ என்ற பெயரில் ஒரு பள்ளியைத் தொடங்கினார். தன் கடின உழைப்பால் பலரும் போற்றும்படி உயர்ந்தார்.
நியூயார்க்கில் ராமகிருஷ்ண தொண்டர் சங்கம் அமைப்பை நிறுவினார். சுவாமி விவேகானந்தரின் சீடரானார். எளிய, தூய, புனித வாழ்வு வாழ்வது, அனைத்து உயிர்களையும் இறைவனாகக் கருதி அவர்களுக்குப் பணி செய்வது ஆகிய விவேகானந்தரின் கொள்கையை ஏற்று செயல்பட்டார்.

சுவாமி விவேகானந்தரின் மறைவிற்குப் பின் பன்மடங்கு உறுதியுடன் இந்தியாவிற்குப் பணி செய்தார். இந்திய விடுதலைக்காகப் போராட முனைந்தார். அரவிந்தருடன் இணைந்தார். உணர்ச்சி பொங்க பேசுவார். உணர்ச்சி ததும்ப எழுதுவார். 1902 ஆம் ஆண்டு சென்னைக்கு வந்தார். இந்தியாவின் ஒருமைப்பாடு என்ற தலைப்பில் உரையாற்றினார்.

மகாகவி பாரதியார் சகோதரி நிவேதிதாவைத் தன் குருவாக ஏற்றுக் கொண்டார். இந்தியப் பெண்களின் முன்னேற்றத்திற்காக உழைத்தவர். பாரதியார் பெண்ணடிமை நீங்க வேண்டும் என்று கருதியதற்கு இவரும் காரணமாவார். தனது சொத்துகளையும் எழுதிய நூல்களையும் இந்தியப் பெண்களுக்கு தேசியக் கல்வி வழங்க எழுதி வைத்துவிட்டார். இவ்வாறு நிவேதிதா தன் வாழ்நாள் முழுவதும் பிறருக்காக உழைத்து உயர்ந்தவர். அதனால் பலராலும் போற்றப்படுகிறார். நாமும் இவரைப் போல இயன்ற வரை பிறருக்காக உழைக்கலாம்.

(ii) பண்டித ரமாபாய் :
பண்டித ரமாபாய் கர்நாடக மாநிலத்தில் பிறந்தவர். பெண்கள் கல்வி பெற முடியாத சூழ்நிலை நிலவிய அக்காலத்தில் இவருடைய தந்தை இவரைப் படிக்க வைத்தார்.

பெண்கள் படும் கொடுமைகளையும் துன்பங்களையும் நேரில் கண்டு மனம் வருந்தினார். பதினாறாம் வயதில் தந்தையை இழந்தார். அதன்பிறகு புராணக் கதைகளைப் பரப்பும் பொருட்டு இந்தியா முழுதும் பயணம் செய்தார். அப்போது ஏராளமான குழந்தை விதவைகளைக் கண்டார். குழந்தைத் திருமண முறையை ஒழிக்க வேண்டும் என எண்ணினார்.

பூனாவுக்குச் சென்றார். ஆரிய மகிளா சமாஜ்’ என்னும் அமைப்பைத் தொடங்கினார். பெண் கல்வி பரவவும், குழந்தைத் திருமணம் ஒழியவும், பாடுபட்டார். 1886 இல் அமெரிக்கா சென்றார். 1889இல் இந்தியா வந்தவர் மும்பையில் சாரதா சதன் என்ற அமைப்பை உருவாக்கினார். குஜராத்திலும் மத்திய பிரதேசத்திலும் பஞ்சம் நிலவியபோது 2000 பெண்களையும் குழந்தைகளையும் ஒரு பண்ணையில் தங்க வைத்துக் காப்பாற்றினார். முக்தி சதன், பிரீதி சதன், சாந்தி சதன் ஆகியன ரமாபாய் உருவாக்கிய பெண்களுக்கான தொண்டு நிறுவனங்கள் ஆகும். பிறருக்காகவே தன் வாழ்நாள் முழுவதும் உழைத்தார்.

தெரிந்து தெளிவோம்

ஊருணி நீர்நிறைந்து அற்றே உலகவாம்
பேரறி வாளன் திரு. (குறள்.215)
உலகினர் விரும்புமாறு உதவி செய்து வாழ்பவரது செல்வமானது ஊருணியில் நிரம்பிய நீர்போலப் பலருக்கும் பயன்படும். பயன்மரம் உள்ளூர்ப் பழுத்து அற்றால் செல்வம்
நயனுடை யான்கண் படின். (குறள்.216)
நற்பண்பு உடையவரிடம் செல்வம் சேர்வது ஊருக்குள் பழமரத்தில் பழங்கள் பழுத்திருப்பதைப் போன்றது.

கூடுதல் வினாக்கள்

நிரப்புக.

1. வாழ்க்கை குறிக்கோள் உடையது.
2. மக்கள் பணியை இறைப் பணியாக எண்ணித் தம் வாழ்நாள் முழுவதும் தொண்டு செய்தவர் தவத்திரு குன்றக்குடி அடிகளார்.
3. குன்றக்குடி அடிகளார் எழுதிய நூல்கள் நாயன்மார் அடிச்சுவட்டில், குறட்செல்வம், ஆலயங்கள் சமுதாய மையங்கள்.
4. குன்றக்குடி அடிகளார் நடத்திய இதழ்கள் அருளோசை, அறிக அறிவியல்.
5. செல்வத்துப் பயனே ஈதல்’ என்று பாடிய நூல் புறநானூறு.
6. வறுமையைப் பிணி என்றும், செல்வத்தை மருந்து என்றும் கூறுவது தமிழ் மரபு.
7. உலகம் உண்ண உண், உடுத்த உடுப்பாய்’ என்று பாடியவர் பாவேந்தர் பாரதிதாசன்.

குறுவினா :

Question 1.
திருவள்ளுவரின் வாழும் நெறி யாது?
Answer:
ஒருவர் எல்லாருக்காகவும் எல்லாரும் ஒருவருக்காவும் என்னும் பொதுவுடைமை நெறியே திருவள்ளுவரின் வாழும் நெறியாகும்.

Question 2.
பொருளீட்டலுக்கு உரிய கரு எது?
Answer:
மற்றவர்களுக்கு வழங்கி, மகிழ்வித்து மகிழ , வாழ்வித்து வாழப் பொருள் தேவை என்பதே பொருளீட்டலுக்கு உரிய கரு ஆகும்.

Question 3.
சமூகத்தின் பொதுநிலை என்று குன்றக்குடி அடிகளார் கூறுவது யாது?
Answer:
இரப்பார்க்கு இல்லென்று இயைவது கரத்தல் அறிவியல் அன்று, அறமும் அன்று. செய்வது செய்து பொருள் ஈட்டி இரப்பார் துன்பத்தை மாற்றுவதே சமூகத்தின் பொதுநிலை என்று குன்றக்குடி அடிகளார் கூறுகிறார்.

சிறுவினா:

Question 1.
நாலாயிரத்திவ்விய பிரபந்தம் பற்றி எழுதி, முதலாழ்வார்களின் பெயர்களை எழுதுக.
Answer:

• திருமாலைப் போற்றிப் பாடியவர்கள் பன்னிரு ஆழ்வார்கள்.
• அவர்கள் பாடிய பாடல்களின் தொகுப்பு நாலாயிரத் திவ்விய பிரபந்தம் ஆகும்.
• இதனைத் தொகுத்தவர் நாதமுனி ஆவார்.
  முதலாழ்வார்கள் : பொய்கையாழ்வார், பூதத்தாழ்வார், பேயாழ்வார் ஆகிய மூவரும் முதலாழ்வார்கள் ஆவர்.

Question 2.
ஒப்புரவு நெறி’ என்ற தலைப்பில் குன்றக்குடி அடிகளார் நிறைவாக கூறியவற்றை எழுதுக.
Answer:

• நாம் இன்று வாழ்வது உண்மை . நமக்கு வாய்த்திருக்கும் வாய்ப்புகளும்
  அருமையானவை.
• ஏன் காலம் கடத்த வேண்டும்? இன்று நன்று, நாளை நன்று என்று எண்ணிக் காலத்தைப் பாழடிப்பானேன்? இன்றே வாழத் தொடங்குவோம்.
• வாழத் தொடங்கியதன் முதற்படியாகக் குறிக்கோளைத் தெளிவாகச் சிந்தித்து முடிவு செய்வோம்.
• இந்தப் புவியை நடத்தும் பொறுப்பை ஏற்போம். பொதுமையில் இந்தப் புவியை நடத்துவோம். பொதுவில் நடத்துவோம்.
• உலகம் உண்ண உண்போம். உலகம் உடுத்த உடுத்துவோம்.
• எங்கு உலகம் தங்கியிருக்கிறதோ அங்கேயே நாமும் தங்குவோம். மண்ணகத்தில் விண்ணகம் காண்போம்.

Students can Download Tamil Chapter 3.1 மலைப்பொழிவு Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 3.1 மலைப்பொழிவு

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
மனித வாழ்க்கையில் தேவைப்படுவது ….
அ) பணம்
ஆ) பொறுமை
இ) புகழ்
ஈ) வீடு
Answer:
ஆ) பொறுமை

Question 2.
சாந்த குணம் உடையவர்கள் ………………… முழுவதையும் பெறுவர்.
அ) புத்தகம்
ஆ) செல்வம்
இ) உலகம்
ஈ) துன்பம்
Answer:
இ) உலகம்

Question 3.
மலையளவு’ என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது ………….
அ) மலை + யளவு
ஆ) மலை + அளவு
இ) மலையின் + அளவு
ஈ) மலையில் + அளவு
Answer:
ஆ) மலை + அளவு

Question 4.
‘தன்னாடு’ என்னும் சொல்லைப் பிரித்து எழுதக் கிடைப்பது ….
அ) தன் + னாடு
ஆ) தன்மை + னாடு
இ) தன் + நாடு
ஈ) தன்மை + நாடு
Answer:
இ) தன் + நாடு

Question 5.
இவை + இல்லாது என்பதனைச் சேர்த்தெழுதக் கிடைக்கும் சொல் …….
அ) இவையில்லாது
ஆ) இவை இல்லாது
இ) இவயில்லாது
ஈ) இவஇல்லாது
Answer:
அ) இவையில்லாது

பொருத்துக

குறுவினா

Quesiton 1.
இந்த உலகம் யாருக்கு உரியது?
Answer:
இந்த உலகம் முழுவதும் சாந்தம் என்னும் அமைதியான பண்புடையவர்களுக்கே

Quesiton 2.
உரியது. உலகம் நிலைதடுமாறக் காரணம் என்ன?
Answer:
உலகம் நிலைதடுமாறக்காரணம்: பலவேறுபட்ட சாதிகளாலும் கருத்துவேறுபாடுகளாலும் இவ்வுலகம் நிலைதடுமாறுகிறது.

Quesiton 3.
வாழ்க்கை மலர்ச்சோலையாக மாற என்ன செய்ய வேண்டும்?
Answer:
வாழ்க்கை மலர்ச்சோலையாக மாற செய்யவேண்டுவன :
(i) மனிதன் ஆசையில் விழுந்துவிட்டால் அவனது வாழ்வு பாலைவனம் போல் பயனற்றதாகிவிடும்.
(ii) வாழ்க்கை மலர்ச்சோலையாக மாற வேண்டுமானால் மனிதன் நல்ல உள்ளத்தோடு வாழவேண்டும்.

சிறுவினா

சாந்தம் பற்றி இயேசு காவியம் கூறுவன யாவை?
Answer:
சாக்கம் பற்றி இயேசு காவியம் கூறுவன:
சாந்தம் என்னும் அமைதியான பண்பு கொண்டவர்களுக்கே இவ்வுலகம் முழுவதும் உரியது. அவர்களே தலைவர்கள் ஆவர் என்ற உண்மையை இயேசுநாதர் கூறினார். மனித வாழ்க்கையில் தேவைப்படுவது பொறுமை. அது மண்ணையும் விண்ணையும் ஆட்சி செய்யும் பெருமையுடையது என்றார்.

இவ்வுலகம் சாதிகளாலும் கருத்து வேறுபாடுகளாலும் நிலைதடுமாறுகிறது. அறம் என்கிற ஒன்றனை நம்பிய பிறகு சண்டைகள் நீங்கி உலகம் அமைதியாகிவிடும். பொருள் – ஈட்டுவதிலும் அறவழியைப் பின்பற்ற வேண்டும். இவ்வுலகம் ஏற்றத்தாழ்வு இல்லா வாழ்வைப் – பெற வேண்டும்.

இரக்கம் உடையவர்கள் பிற உயிர்களின் மீது இரக்கம் காட்டி இறைவனின் இரக்கத்தைப் பெறுவர். இதுதான் அவர்களுக்கான பரிசு . மனிதன் ஆசையில் விழுந்துவிட்டால் அவனது வாழ்வு பாலைவனம் போல் பயனற்றதாகிவிடும். நல்ல உள்ளத்தோடு வாழ்ந்தால் அவனுடைய வாழ்க்கை மலர்ச்சோலையாக மாறிவிடும்.

மனிதர்கள் சண்டை சச்சரவுகளால் தாமும் துன்புற்றுப் பிறரையும் துன்புறுத்துகின்றனர். அவர்கள் தன்னாடு , பிறநாடு என்று பேசி உண்மையில்லா உறவுகளாக வாழ்கின்றனர்.

கண்ணிமைக்கும் நேரத்திற்குள் நடக்கும் ஆயிரம் போட்டிகளால் பயனற்ற கனவுகள் தாம் தோன்றுகின்றன. இவை இல்லாமல் அமைதியாக வாழ்ந்தால் இதயம் மலையளவு உயர்ந்ததாக மாறும்.

சிந்தனை வினா

எல்லா மக்களும் ஒற்றுமையாக வாழ என்ன செய்ய வேண்டும்?
Answer:
எல்லா மக்களும் ஒற்றுமையாக வாழ செய்ய வேண்டுவன :
(i) மாணவர்கள் பள்ளியில் தன் நண்பர்களுடன் ஒற்றுமையைக் கடைப்பிடிக்க வேண்டும். சக மாணவர்களின் இன்ப துன்பங்களில் பங்கேற்க வேண்டும். அவர்கள் கற்பதற்கும் பாடங்களைப் படிப்பதற்கும் உதவி புரிய வேண்டும்.

(ii) வீட்டில் தன் அக்கா, தங்கை, அண்ணன், தம்பி ஆகியோருடன் விட்டுக் கொடுத்துப் பழக வேண்டும். நான், நீ என்று போட்டிப் போடக்கூடாது. ஆணவமின்றி இருக்க வேண்டும். நாம் சொல்வதை மட்டும் எல்லோரும் செய்ய வேண்டும் என்று நினைக்கக்கூடாது.

(iii) பொது இடங்களில் (சமுதாயம்) சாதி மத பேதங்களை பார்க்கக்கூடாது. இயற்கை அனைவருக்கும் பொது என்பதனைப் போல் தெய்வமும் பொதுவானது என உணர வேண்டும். பிற மதத் தெய்வங்களை வணங்கவில்லை யென்றாலும், அத்தெய்வங்களைப் பற்றியோ, மதத்தினர் பற்றியோ அவதூறு பேசக்கூடாது. உயர்ந்த சாதி, தாழ்ந்த சாதி என்று பாராமல் அனைவரும் ஒரே மனித சாதி என
எண்ண வேண்டும்.

(iv) யாதும் ஊரே யாவரும் கேளிர்’ என்று கணியன் பூங்குன்றனார் கூறியதை நினைவில் வைத்து வாழ்ந்தால் அனைவரும் ஒற்றுமையுடன் வாழ முடியும்.

கற்பவை கற்றபின்

இயேசுவின் வாழ்வில் நடந்த சுவையான நிகழ்வு ஒன்றினை அறிந்து வந்து வகுப்பறையில் பகிர்க.
Answer:
(i) ஒரு பெண் பன்னிரண்டு ஆண்டுகளாகத் தீராத நோயினால் துன்பப்படுகின்றாள். அவள் எப்படியாவது அந்நோய் குணமாக வேண்டும் என்று நினைத்தாள். அவள் கூட்டத்திற்கிடையில் நின்றிருந்த இயேசுவின் பின்னால் போய் அவருடைய அங்கியின் ஓரத்தைத் தொட்டாள். உடனடியாக அவள் குணமாகிவிட்டாள். அப்போது இயேசு , “யார் என்னைத் தொட்டது?” என்று கேட்டார். அந்தப் பெண் பயந்து போனாள். பிறகு அவர் முன்னால் வந்து உண்மையைச் சொன்னாள். இயேசு அவளிடம் மகளே , நிம்மதியாகப் போ’ என்று ஆறுதலாகச் சொன்னார்.

(ii) இன்னொரு முறை, யவீரு என்ற அதிகாரி இயேசுவிடம் வந்து, தயவுசெய்து என் வீட்டுக்கு வாருங்கள். என்னுடைய குட்டிப் பெண் மிகவும் உடம்பு முடியாமல் இருக்கிறாள் என்று கெஞ்சினார். ஆனால், இயேசு அங்கே போவதற்கு முன்பே அவள் இறந்து விட்டாள். இருந்தாலும், யவீருவின் வீட்டுக்கு இயேசு போனார். அங்கே நிறைய பேர் அந்தக் குடும்பத்தாருடன் சேர்ந்து புலம்பி அழுது கொண்டிருந்தார்கள். இயேசு அவர்களிடம், “அழாதீர்கள், அவள் தூங்குகிறாள்” என்று சொன்னார். பிறகு, அந்தப் பெண்ணின் கையைப் பிடித்து, சிறுமியே, எழுந்திரு!” என்று சொன்னார். உடனே அவள் எழுந்து உட்கார்ந்தாள். சாப்பிட அவளுக்கு ஏதாவது கொடுக்கும்படி இயேசு அவளுடைய அப்பா, அம்மாவிடம் சொன்னார். அந்த பெற்றோர் அளவிலா ஆனந்தம் அடைந்தனர். இதேபோல் பல நிகழ்வுகள் இயேசுவின் வாழ்வில் நிகழ்ந்துள்ளன.

கூடுதல் வினாக்கள்

நிரப்புக.

1. கண்ணதாசனின் இயற்பெயர் முத்தையா.
2. கண்ண தாசனின் சிறப்புப் பெயர் கவியரசு .
3. கண்ணதாசன் தமிழக அரசவைக் கவிஞராக இருந்துள்ளார்.
4. இயேசுவின் வாழ்க்கை வரலாற்றையும் அவரது அறிவுரைகளையும் கூறும் நூல் இயேசு காவியம் ஆகும்.
5. மலைப்பொழிவு என்னும் பகுதி இயேசு காவியம் என்னும் நூலிலிருந்து எடுக்கப்பட்டுள்ளது.
6. இவ்வுலகம் ஏற்றத்தாழ்வு இல்லா வாழ்வைப் பெற வேண்டும்.
7. போட்டி இல்லாமல் அமைதியாக வாழ்ந்தால் இதயம் மலையளவு உயர்ந்ததாக மாறும்.

விடையளி :

Question 1.
கவிஞர் கண்ணதாசன் எவ்வகையான நூல்களை எழுதினார்?
Answer:
காவியங்கள், கவிதைகள், கட்டுரைகள், சிறுகதைகள், நாடகங்கள், புதினங்கள் போன்ற இலக்கிய வடிவங்களில் பல்வேறு நூல்களை எழுதியுள்ளார் கண்ணதாசன்.

Question 2.
தலைவர்கள் என்று இயேசுநாதர் குறிப்பிடப்பட்டவர் யாவர்?
Answer:
சாந்தம் என்னும் அமைதியான பண்பு கொண்டவர்கள் பேறு பெற்றவர்கள். இந்த உலகம் முழுவதும் அவர்களுக்கே உரியது. அவர்களே தலைவர்கள் ஆவர் என்று இயேசுநாதர் கூறுகிறார்.

Question 3.
அறம் பற்றி இயேசுநாதர் கூறியவை பற்றி எழுதுக.
Answer:
இவ்வுலகம் சாதிகளாலும் கருத்து வேறுபாடுகளாலும் நிலைதடுமாறுகிறது. அறம் என்கிற ஒன்றனை நம்பிய பிறகு சண்டைகள் நீங்கி உலகம் அமைதியாகி விடும். பொருள் ஈட்டுவதிலும் அறவழியைப் பின்பற்ற வேண்டும் என்று இயேசுநாதர் கூறியுள்ளார்.

Question 4.
உண்மையில்லா உறவுகளாக இயேசுநாதர் கூறுவது யாது?
Answer:
மனிதர்கள் சண்டை சச்சரவுகளால் தாமும் துன்புற்றுப் பிறரையும் துன்புறுத்துகின்றனர். மேலும், அவர்கள் தன்னாடு, பிறர்நாடு என்றும் பேசி உண்மையில்லா உறவுகளாக வாழ்கின்றனர்.

பாடலின் பொருள்

(தம் சீடர்களுக்கு அறிவுரை கூற எண்ணிய இயேசுநாதர் ஒரு குன்றின் மீது ஏறி நின்று பேசத் தொடங்கினார்.)

சாந்தம் என்னும் அமைதியான பண்பு கொண்டவர்கள்தான் பேறு பெற்றவர்கள். இந்த உலகம் முழுவதும் அவர்களுக்கே உரியது. அவர்களே தலைவர்கள் ஆவர் என்ற உண்மையை இயேசுநாதர் கூறினார். மனித வாழ்க்கையில் தேவைப்படுவது பொறுமை. அது மண்ணையும் விண்ணையும் ஆட்சி செய்யும் பெருமையுடையது என்றார்.

இவ்வுலகம் சாதிகளாலும் கருத்து வேறுபாடுகளாலும் நிலைத்தடுமாறுகிறது. அறம் என்கிற ஒன்றனை நம்பிய பிறகு சண்டைகள் நீங்கி உலகம் அமைதியாகி விடும். பொருள் ஈட்டுவதிலும் அறவழியைப் பின்பற்ற வேண்டும். இவ்வுலகம் ஏற்றத்தாழ்வு இல்லா வாழ்வைப் பெற வேண்டும்.

இரக்கம் உடையோரே பேறுபெற்றவர் ஆவர். அவர்கள் பிற உயிர்களின் மீது இரக்கம் காட்டி இறைவனின் இரக்கத்தைப் பெறுவர். இதுதான் அவர்களுக்கான பரிசு . மனிதன் ஆசையில் விழுந்துவிட்டால் அவனது வாழ்வு பாலைவனம் போல் பயனற்றதாகிவிடும். அவன் நல்ல உள்ளத்தோடு வாழ்ந்தால் அவன் வாழ்க்கை மலர்ச்சோலையாக மாறிவிடும்.

மனிதர்கள் சண்டை சச்சரவுகளால் தாமும் துன்புற்றுப் பிறரையும் துன்புறுத்துகின்றனர். மேலும் அவர்கள் தன்னாடு என்றும், பிறர்நாடு என்றும் பேசி உண்மையில்லா உறவுகளாக வாழ்கின்றனர்.

கண்ணிமைக்கும் நேரத்திற்குள் நடக்கும் ஆயிரம் போட்டிகளால் பயனற்ற கனவுகள்தாம் தோன்றுகின்றன. இவை இல்லாமல் அமைதியாக வாழ்ந்தால் இதயம் மலையளவு உயர்ந்ததாக மாறும்.

Students can Download Tamil Chapter 2.4 உண்மை ஓளி Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 2.4 உண்மை ஓளி

மதிப்பீடு

Question 1.
உண்மை ஒளி’ படக்கதையைச் கதையாகச் சுருக்கி எழுதுக.
Answer:
ஜென் குரு ஒருவர் மாணவர்களுக்குப் பாடம் கற்பித்துக் கொண்டிருந்தார். குழந்தைகளிடம் உண்மையான ஒளி எது?’ என்பதைப் பற்றி இன்று அறிந்து கொள்ளப் போகிறோம் என்று கூறிவிட்டு தொடங்கினார்.

பிறப்பின் அடிப்படையில் எல்லா உயிரும் ஒன்றே. பசி, தாகம், தூக்கம் ஆகியவை எல்லா உயிர்களுக்கும் உண்டு. அதுமட்டுமில்லாமல் இரவும் பகலும் மாறி மாறி வருவதுபோல வாழ்வில் இன்பமும் துன்பமும் மாறி மாறி வரும் என்று கூறினார்.

பிறகு, “இருள் கலைந்து வெளிச்சம் வந்துவிட்டது என்பதை எந்த நொடியில் நீங்கள் அறிவீர்கள்?” என்று மாணவர்களிடம் கேட்டார். அதற்கு ஒரு மாணவன், “தொலைவில் விலங்கு குதிரையா? கழுதையா? என்பதை அடையாளம் காணக்கூடிய நொடியில் வெளிச்சம் வந்துவிட்டதை நான் அறிவேன் ஐயா” என்றான். அதனைக் குரு ஏற்றுக் கொள்ளவில்லை.

மற்றொரு மாணவன் “தூரத்திலிருக்கும் மரம் ஆலமரமா? அரசமரமா? என்பதை அடையாளம் காணக்கூடிய நேரத்தில் உண்மையாக விடிந்துவிட்டது என்பதை அறியலாம். சரிதானே ஐயா?” என்றான்.

குரு “வேறு யாருக்காவது தெரியுமா?” என்று கேட்டார். உடனே மாணவர்கள் “எங்களுக்குத் தெரியவில்லை ஐயா, தாங்களே கூறிவிடுங்கள்” என்று கேட்டுக் கொண்டனர். ஒரு மனிதரைக் காணும்போது இவர் என் உடன்பிறந்தவர் என்று எப்போது நீங்கள் உணர்கிறீர்களோ, அப்போதுதான் உண்மையான ஒளி உங்களுக்குள் ஏற்படுகிறது என்பது பொருள்” என்று குரு கூறினார்.

“இரவும் பகலும் வெறும் காலவேறுபாடுகள் தான். உண்மையான ஒளி உள்ளத்தின் உள்ளே ஏற்பட வேண்டியது என்பதை நீங்கள் அளித்த விளக்கத்தின் மூலம் நாங்கள் புரிந்து கொண்டேம் ஐயா” என்று மாணவர்கள் கூறினர். உள்ளுக்குள் ஒளி இல்லையென்றால் உச்சி வெயில் கூடக் காரிருளே என்பதைப் புரிந்து கொள்ளுங்கள் என்று கூறிவிட்டு அங்கிருந்து சென்றுவிட்டார்.

வகுப்பு முடிந்த பிறகு குரு அருகில் உள்ள சிற்றூருக்குப் புறப்பட்டுச் சென்றார். இருட்டுவதற்குள் ஊரை அடைய வேண்டும் என்று எண்ணிக்கொண்டே சென்றார். சாலையோரம் ஒருவன் மயங்கிக் கிடந்தான். குதிரையை நிறுத்தி, கீழே இறங்கிய குரு அந்த மனிதனை எழுப்பிப் பார்த்தார். அவன் மயங்கிய நிலையிலேயே இருந்தான். மீண்டும் மீண்டும் எழுப்பியதில் மயங்கியவன் எழுந்தான்.

குரு , கொஞ்சம் நீரைக் கொடுத்துக் குடிக்கச் செய்தார். மயங்கியவன், தான் பசியால் மயங்கி விழுந்துவிட்டதாகவும் பக்கத்து ஊருக்குச் செல்ல வேண்டும் என்றும் கூறினான். மேல் பரிதாபப்பட்டு அவனைக் கிரையில் அன வனைக் குதிரையில் அழைத்துச் செல்ல N எண்ணினார். அதனால் அவனை மெதுவாகக் குதிரையின் மீது குரு ஏற்றினார்.

குதிரையில் ஏறிய அவன் குதிரையை அடித்து விரட்டத் தொடங்கினான். குதிரையைத் – திருடுவதற்குகாகத்தான் மயங்கியவன் போல் நடித்துள்ளான் என்பதைக் குரு உணர்ந்தார்.

குரு குதிரையில்லாமல் எங்கும் செல்ல இயலாது என்பதால், எப்படியாவது ஒரு கை குதிரையை வாங்கிக் கொண்டுதான் ஊருக்குத் திரும்ப வேண்டும் என்று முடிவெடுத்தார். அந்தச் சந்தையில் குருவின் குதிரை நின்று கொண்டிருந்தது. குரு அவன் தோளைத் தொட்டார். குழந்தாய்! என்று அழைத்தார். ஒன்றும் தெரியாதவன் போல் குருவைப் பார்த்து “ஆ! நீங்களா?” என்று கேட்டான். குரு அவனிடம், ” யாரிடமும் சொல்லாதே!” என்றார். குதிரையைத் திருடியவன் எதை? ஏன்?” என்று கேட்டான். குதிரையை நீயே வைத்துக்கொள். ஆனால், இது உனக்கு எப்படிக் கிடைத்தது என்று யாரிடமும் சொல்லாதே” என்று குரு கூறினார். திருடன் இவர் ஏமாந்தது யாருக்காவது தெரிந்தால் அவமானம் என்று நினைக்கிறார் போலிருக்கிறது என்று மனதிற்குள் நினைத்துக் கொண்டான்.

உடனே, குரு “நீ நினைப்பது எனக்குப் புரிகிறது குழந்தாய்! ஆனால், நான் ஏமாந்து போனது தெரிந்தால் எதிர்காலத்தில் உண்மையிலேயே யாராவது சாலையில் மயங்கிக் கிடந்தால் கூட அவர்களுக்கு யாரும் உதவ முன்வரமாட்டார்கள். புரிகிறதா? குறுகிய தன்னலத்துக்காக நல்ல கோட்பாடுகளை அழித்துவிடக்கூடாது. இதை நீ தெரிந்துகொள்” என்று கூறினார்.
குதிரையைத் திருடியவன் குருவின் பெருந்தன்மையை உணர்ந்து வெட்கித் தலை குனிந்தான்.

கற்பவை கற்றபின்

Question 1.
ஜென் கதைகளில் வேறு சிலவற்றை அறிந்து வந்து வகுப்பறையில் பகிர்க.
Answer:
ஜென் கதை – வெற்றியைத் தீர்மானிப்பது கடவுளா?
இரு நாட்டுப் படைகளுக்கும் இடையே வெகு மும்முரமாக சண்டை நடந்து கொண்டிருந்தது. எதிரி நாட்டுப் படையிடம் கிட்டத்தட்ட தோற்றுவிட்ட நிலை. ஆனாலும் தாய்நாட்டுப் படைத்தளபதிக்கு போரை இழக்க மாட்டோம் என்ற அசாத்திய நம்பிக்கை. ஆனால் துணைத் தளபதி உள்ளிட்ட அவன் வீரர்களுக்கு அந்த நம்பிக்கை கொஞ்சம் கூட இல்லை. எல்லோரும் ஓடுவதில் குறியாக இருந்தனர்.

என்னதான் நம்பிக்கை இருந்தாலும் வீரர்களில்லாமல் தனி ஆளாய் என்ன செய்ய இயலும்? கடைசி நாள் சண்டை. போர்க்களத்துக்குப் போகும் வழியில் ஒரு கோயிலைக் கண்டார்கள். உடனே தளபதி வீரர்களை அழைத்து, “சரி வீரர்களே… நாம் ஒரு முடிவுக்கு வருவோம். இதோ இந்தக் கோயிலுக்கு முன் ஒரு நாணயத்தைச் சுண்டி விடுகிறேன். அதில் தலைவிழுந்தால் வெற்றி நமக்கே. பூ விழுந்தால் நாம் தோற்பதாக அர்த்தம். இப்படியே திரும்பி விடுவோம். வெற்றியா? தோல்வியா? நமக்கு மேல் உள்ள சக்தி தீர்மானிக்கட்டும்… சரியா?” “ஆ…. நல்ல யோசனை. அப்படியே செய்வோம்.”

நாணயத்தைச் சுண்டினான் தளபதி. காற்றில் மிதந்து, விர்ரென்று சுழன்று தரையில் விழுந்தது நாணயம்.
தலை…!
வீரர்கள் உற்சாகத்தில் மிதந்தனர். வெற்றி, வெற்றி என்று எக்காளமிட்டபடி போர்க்களம் நோக்கி ஓடினர். வெகு வேகமாக எதிரி நாட்டினருடன் சண்டையிட்டனர்.

அம்! என்ன ஆச்சர்யம். அந்த சிறிய படை, எதிரி நாட்டின் பெரும் படையை வீழ்த்திவிட்டது. துணைத் தளபதி வந்தான். “நாம் வென்றுவிட்டோம். கடவுள் தீர்ப் முடியாதல்லவா….” என்றான் உற்சாகத்துடன்.
“ஆமாம்… உண்மைதான்” என்று கூறியபடி அந்த நாணயத்தைத் துணைத் தளபதியிடம் கொடுத்தான் தளபதி.

நாணயத்தின் இருபக்கங்களிலும் தலை.

இக்கதையில் மூலம் நாம் உணர்வது தன்னம்பிக்கைதான் வெற்றியின் முதல்படி என்பதாகும்.

Question 2.
உண்மை ஒளி’ படக்கதையை வகுப்பறையில் நாடகமாக நடித்துக் காட்டுக.
Answer:

காட்சி -1

ஜென் குரு : குழந்தைகளே! உண்மையான ஒளி எது என்பதைப் பற்றி இன்று அறிந்து கொள்ளப் போகிறோம்.
மாணவர்கள் : அறிந்து கொள்ள ஆவலாக உள்ளோம் ஐயா!
ஜென் குரு : பிறப்பின் அடிப்படையில் எல்லா உயிரும் ஒன்றே. பசி, தாகம், தூக்கம் ஆகியவை எல்லா உயிர்களுக்கும் உண்டு.

மேலும் இரவும் பகலும் மாறி மாறி வருவது போல வாழ்வில் இன்பமும் துன்பமும் மாறி மாறி வரும். இப்பொழுது உங்களை ஒரு கேள்வி கேட்கட்டுமா? இருள் கலைந்து வெளிச்சம் வந்துவிட்டது என்பதை எந்த நொடியில் நீங்கள் அறிவீர்கள்?
மாணவன் : தொலைவில் நிற்கும் விலங்கு குதிரையா? கழுதையா? என்பதை அடையாளம் காணக்கூடிய நொடியில் வெளிச்சம் வந்துவிட்டதை நான் அறிவேன் ஐயா.

ஜென் குரு : இல்லை. வேறு யாராவது கூறுங்கள் பார்ப்போம்.
மாணவன் : தூரத்திலிருக்கும் மரம் ஆலமரமா? அரசமரமா? என்பதை அடையாளம் காணக்கூடிய நேரத்தில் உண்மையாக விடிந்துவிட்டது என்பதை அறியலாம். சரிதானே ஐயா!
ஜென் குரு : இல்லை. வேறு யாருக்காவது தெரியுமா? மாணவர்கள் எங்களுக்குத் தெரியவில்லை ஐயா. தாங்களே கூறி விடுங்கள்.
ஜென் குரு : ஒரு மனிதரைக் காணும் போது இவர் உடன்பிறந்தவர் என்று எப்போது நீங்கள் உணர்கிறீர்களோ, அப்போதுதான் உண்மையான ஒளி உங்களுக்குள் ஏற்படுகிறது என்பது பொருள்.
மாணவர்கள் : இரவும் பகலும் வெறும் கால வேறுபாடுகள் தான். உண்மையான ஒளி உள்ளத்தின் உள்ளே ஏற்பட வேண்டியது என்பதை தாங்கள் புரிந்துகொண்டோம் ஐயா.
ஜென் குரு : உள்ளுக்குள் ஒளி இல்லையென்றால் உச்சி வெயில் கூடக் காரிருளே என்பதைப் புரிந்து கொள்ளுங்கள். மீண்டும் நாளை சந்திப்போம். வகுப்பு முடிந்து மாணவர்கள் கலைந்து செல்கின்றனர்.)

காட்சி – 2

(குரு அருகில் உள்ள சிற்றூருக்குப் புறப்படுகிறார்.)
ஜென் குரு : (மனதிற்குள்) இருட்டுவதற்குள் ஊரை அடைய வேண்டும்.
ஜென் குரு :(வழியில்) ஆ! யாரது சாலையோரம் படுத்துக்கிடப்பது.
(குதிரையை நிறுத்தி, கீழே இறங்கிய குரு அந்த மனிதனை எழுப்புகிறார்)
ஜென் குரு : குழந்தாய்! எழுந்திரு. நீ யார்? ஏன் இங்கே படுத்திருக்கிறாய்?
(மனதிற்குள்) இவன் மயக்கம் அடைந்திருக்கிறான்.
(படுத்திருப்பவனுக்கு நீரைப் பருகத் தருகிறார் குரு.)
ஜென் குரு : குழந்தாய்! எழுந்திரு . இந்த நீரைக் கொஞ்சம் குடி.
(மயக்கமடைந்தவன் எழுந்து உட்காருகிறான்.)

வழிப்போக்கன் : பசியால் மயங்கி விழுந்து விட்டேன். ஐயா. நான் பக்கத்து ஊருக்குச் செல்ல வேண்டும்.
ஜென் குரு : அப்படியா! சரி என்னிடம் குதிரையிருக்கிறது. நான் உன்னை அழைத்துச் செல்கிறேன். (குரு , அவனைத் தன் குதிரையின் மீது உட்கார வைக்கிறார்.)
ஜென் குரு : மெதுவாக ஏறுப்பா! பார்த்து உட்கார். (குதிரையில் ஏறிய அவன் குதிரையை அடித்து விரட்டத் தொடங்குகிறான்.)
ஜென் குரு : ஆ! என்ன இது? ஓ! இவன் திருடன் போல இருக்கிறது. என் குதிரையைத் திருடவே இப்படி நடித்திருக்கிறான். (குரு ஏமாற்றத்துடன் நடந்து ஊரை அடைகிறார்.)

காட்சி – 3

இடம் : சந்தை
ஜென் குரு – (மனதிற்குள்) இங்கு எப்படியாவது ஒரு குதிரையை வாங்கிக் கொண்டுதான் ஊருக்குத் திரும்ப வேண்டும்.
ஜென் குரு : ஆ! அதோ அங்கு நிற்பது என்னுடைய குதிரையைப் போல் உள்ளதே
(குருவிடம் குதிரையைத் திருடியவன் அங்கு நிற்கிறான். குரு அவன் தோளைத் தொடுகிறார்)
ஜென் குரு : குழந்தாய்!
திருடன் : ஆ. நீங்களா? (குரு மெல்லச் சிரிக்கிறார்)
ஜென் குரு : யாரிடமும் சொல்லாதே !
திருடன் : எதை? ஏன்?

ஜென் குரு : குதிரையை நீயே வைத்துக்கொள். ஆனால், இது உனக்கு எப்படிக் கிடைத்தது என்று யாரிடமும் சொல்லாதே.
திருடன் : (மனத்திற்குள்) இவர் ஏமாந்தது யாருக்காவது தெரிந்தால் அவமானம் என்று நினைக்கிறார் போலிருக்கிறது.
ஜென் குரு : நீ நினைப்பது எனக்குப் புரிகிறது குழந்தாய்! ஆனால், நான் ஏமாந்து போனது தெரிந்தால் எதிர்காலத்தில் உண்மையிலேயே யாராவது சாலையில் மயங்கிக் கிடந்தால் கூட அவர்களுக்கு யாரும் உதவ முன்வர மாட்டார்கள். புரிகிறதா? குறுகிய தன்னலத்துக்காக நல்ல கோட்பாடுகளை அழித்துவிடக்கூடாது. இதை நீ தெரிந்து கொள்.

Students can Download Tamil Chapter 3.5 ஆகுபெயர் Questions and Answers, Summary, Notes Pdf, Samacheer Kalvi 7th Tamil Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 7th Tamil Solutions Term 3 Chapter 3.5 ஆகுபெயர்

மதிப்பீடு

சரியான விடையைத் தேர்ந்தெடுத்து எழுதுக.

Question 1.
பொருளின் பெயர் அதன் உறுப்புக்கு ஆகி வருவது ……………..
அ) பொருளாகு பெயர்
ஆ) சினையாகு பெயர்
இ) பண்பாகு பெயர்
ஈ) இடவாகு பெயர்
Answer:
அ) பொருளாகு பெயர்

Question 2.
இந்த வேலையை முடிக்க ஒரு கை குறைகிறது என்பது …………
அ) முதலாகு பெயர்
ஆ) சினையாகு பெயர்
இ) தொழிலாகு பெயர்
ஈ) பண்பாகு பெயர்
Answer:
ஆ) சினையாகு பெயர்

Question 3.
மழை சடசடவெனப் பெய்தது. இத்தெடரில் அமைந்துள்ளது …………..
அ) அடுக்குத்தொடர்
ஆ) இரட்டைக்கிளவி
இ) தொழிலாகு பெயர்
ஈ) பண்பாகுபெயர்
Answer:
ஆ) இரட்டைக் கிளவி

Question 4.
அடுக்குத் தொடரில் ஒரே சொல் …………………… முறை வரை அடுக்கி வரும்.
அ) இரண்டு
ஆ) மூன்று
இ) நான்கு
ஈ) ஐந்து
Answer:
இ) நான்கு

குறுவினா

Question 1.
ஒரு பெயர்ச்சொல் எப்போது ஆகுபெயர் ஆகும்?
Answer:
ஒரு பெயர்ச்சொல் அதனைக் குறிக்காமல் அதனோடு தொடர்புடைய வேறு ஒன்றிற்கு ஆகி வரும்போது அது ஆகுபெயர் ஆகிறது.

Question 2.
இரட்டைக் கிளவி என்பது யாது? சான்று தருக.
Answer:
சொற்கள் இரட்டையாக இணைந்து வந்து, பிரித்தால் தனிப்பொருள் தராத சொற்கள் இரட்டைக் கிளவி எனப்படும். சான்று : விறுவிறு , கலகல, மளமள மாலா கலகலவெனச் சிரித்தாள்.

சிறுவினா 

Question 1.
பொருளாகு பெயரையும் சினையாகு பெயரையும் வேறுபடுத்துக.
Answer:

Question 2.
இரட்டைக்கிளவி, அடுக்குத்தொடர் – ஒப்பிடுக.
Answer:

கற்பவை கற்றபின்

Question 1.
பள்ளி நூலகத்திலிருந்து நூல் ஒன்றை எடுத்துவந்து அந்நூலில் ஆகுபெயர்களாக இடம் பெற்றுள்ள பெயர்ச்சொற்களைத் தொகுக்க.
Answer:
முகிலன் தன் பெற்றோருடன் தீபாவளிக்குப் புதிய ஆடை வாங்குவதற்குக் கடைக்குச் சென்றான். முகிலனின் அப்பா அவன் அம்மாவிடம் “காஞ்சி வேணுமா? ஆரணி வேணுமா?” என்று கேட்டார். முகிலனின் அம்மா, எனக்கு காஞ்சிதான் வேணும்” என்று கூறினார். பார்த்தவுடனேயே தேர்ந்தெடுத்துவிட்டார். பிறகு முகிலனுக்கு முழுக்கால் சட்டைக்கும் மேல் சட்டைக்கும் துணி எடுக்க ஆண்கள் பிரிவுக்குச் சென்றனர். கடைக்காரரிடம் ‘2 மீட்டர் கொடு’ என்று கேட்டார். தனக்கும் எடுத்துக் கொண்டு கடையை விட்டு வெளியேறினர். முகிலன் தன் தந்தையிடம் பசிக்கிறது என்று கூறியதால் உணவகம் சென்றனர்.

மூவரும் பொங்கல் உண்டனர். முகிலனின் அம்மா முருங்கை சாம்பார் நன்றாக உள்ளது என்று கூறினார். அங்கு முகிலனின் மாமாவைப் பார்த்தனர். அவருடன் பேசிக் கொண்டே பேருந்து நிலையம் சென்றனர். முகிலனின் அப்பா மாமாவிடம், “வயலில் என்ன பயிர் வைத்துள்ளாய்?” என்று கேட்டார். மாமா , “கீரை விதைத்துள்ளேன்” என்றார். மேலும், “செவலைக்குக் காலில் அடிப்பட்டு விட்டது. கால்நடை மருத்துவமனைக்கு அழைத்துச் செல்ல வேண்டும். ஒரே அலைச்சலாக இருக்கிறது” என்று கூறினார். விவசாயம் செய்வதிலும் பல துன்பங்கள் இருக்கத்தான் செய்கிறது. என்ன செய்வது? சரி பேருந்து வந்து விட்டது. நாங்கள் புறப்படுகிறோம்” என்று கூறிவிட்டுப் புறப்பட்டனர்.

Question 2.
அன்றாடப் பேச்சு வழக்கில் இடம்பெறும் அடுக்குத்தொடர், இரட்டைக் கிளவி ஆகியவற்றைத் தொகுக்க.
Answer:
அடுக்குத் தொடர் :
1. ஓட்டப்பந்தயத்தில் ஓடிய நண்பனை நான் ஓடு ஓடு என்று உற்சாகப்படுத்தினேன்.
2. எழுத எழுத கையெழுத்து அழகாக இருக்கும்.
3. இவ்விழாவிற்கு வருகை தந்துள்ள அனைவரையும் வருக வருக என வரவேற்றார் பள்ளி முதல்வர்.
4. கந்தன் பாம்பு பாம்பு என்று அலறினான்.
5. பேச்சுப்போட்டியில் பேசிய மாணவனை அனைவரும் அருமை அருமை என்று பாராட்டினார்.

இரட்டைக் கிளவி :

1. முல்லை மலர் கமகம என் மணம் வீசுகிறது.
2. மரக்கிளை மடமட என முறிந்தது.
3. வெளியில் சென்று வந்தாலே கசகச என வியர்வை வந்து விடும்.
4. கொடைக்கானல் சென்ற போது கிடுகிடு பள்ளத்தைப் பார்த்தேன்.
5. வாக்குச்சாவடிக்கு வாக்களிக்க குடுகுடு கிழவர் வந்தார்.

கூடுதல் வினாக்கள்

நிரப்புக.

1. ஒன்றன் பெயர் அதனைக் குறிக்காமல் அதனோடு தொடர்புடைய வேறு ஒன்றிற்கு ஆகி வருவது ஆகுபெயர் எனப்படும்.
2. பொருளின் பெயர் அதன் சினையாகிய உறுப்புக்கு ஆகி வருவது பொருளாகு பெயர்.
3. சினையின் (உறுப்பின்) பெயர் முதலாகிய பொருளுக்கு ஆகிவருவது சினையாகு பெயர்.
4. இந்தியா வென்றது – இடவாகுபெயர்.
5. திசம்பர் சூடினாள் – காலவாகுபெயர்.
6. இனிப்பு தின்றான் – பண்பாகு பெயர்
7. சுண்டல் தின்றான் – தொழிலாகு பெயர்
8. இரட்டைச் சொற்களாகவே வரும் சொற்கள் இரட்டைக்கிளவி.
9. ஒரே சொல் இரண்டு முதல் நான்கு முறை வரை வரும் சொல் அடுக்குத்தொடர்.

மொழியை ஆள்வோம்

கேட்க.

மனித நேயம் பற்றித் தலைவர்கள் ஆற்றிய உரைகளைக் கேட்டு மகிழ்க.
Answer:
மாணவர்கள் தாங்களாகவே மனித நேயம் பற்றித் தலைவர்கள் ஆற்றிய உரைகளைக் கேட்டு மகிழ வேண்டும்.

கீழ்க்காணும் தலைப்புகளுள் ஒன்றைப் பற்றி இரண்டு நிமிடம் பேசுக.

அ) உண்மை
Answer:
அனைவருக்கும் வணக்கம்!
உண்மை – வாய்மை என்றும் மெய் என்றும் கூறப்படும். இவ்வாய்மையை வள்ளுவர்
“வாய்மை எனப்படுவதுயாதெனின் யாதொன்றும்
தீமை இலாத சொலல்.”

– என்று கூறுகிறார். வாய்மை என்றால் பிறர் மனம் புண்படாதபடி பேசுதல் என்கிறார்.

ஒருவரைப் பெரியவர் சிறியவர் என்று இனம் காண்பது அவர்கள் செய்யும் செயல்களை வைத்துதான். எல்லோருக்கும் உண்மையாக இருங்கள். பொய் கூறாதீர்கள். ஆயிரம் நன்மைகள் கிடைத்தாலும் பொய்மையை அகற்றி உண்மையைக் கடைப்பிடிக்க வேண்டும்.

உண்மை என்றவுடன் நம் நினைவிற்கு வருபவர்கள் இரண்டு பேர். ஒருவர் அரிச்சந்திரன். மற்றொருவர் காந்தியடிகள். அரிச்சந்திரன் பல துன்பங்களுக்கு ஆளாக்கப்பட்டாலும் உண்மையை மட்டுமே பேசியவன். அந்த உறுதிப்பாட்டினால் என்றென்றும் மக்கள் மனதில் நிற்கிறான்.

அரிச்சந்திரன் நாடகத்தைப் பார்த்துதான் காந்தியடிகள் தாம் உண்மையைப் பேச வேண்டும் என்று முடிவெடுத்தவர்.

உண்மை என்பது நாம் கடைப்பிடிக்க வேண்டிய அறங்களில் முதன்மையானதாகும். நேர்மை என்றால் நேர்த்தியான வழியில் செல்லுதல், நியாயம் பார்த்து நடத்தல், குறுக்கு வழியில் சாதிக்க நினைக்காமல், உண்மைப் பாதையை விட்டு நீங்காது இருத்தலும் ஆகும்.

எச்செயல் செய்தாலும் உண்மையை மறவாமல் அதன் நினைவைத் துறவாமல் செய்வது அவசியம் என்பது நம் முன்னோர்களின் கருத்து. அப்படிச் செய்யும் செயல்கள் நமக்கு நன்மையைத் தரும்.

உள்ளதை உள்ளவாறே கூறுதல் பற்றி, மகாவீரர், “நீ உண்மை பேசுவதினால், ஒருவர் மனம் நோகும் என்றாலோ, உன் பேச்சு ஒருவருக்கும் பிடிக்காது என்றாலோ, பிற உயிர்களுக்குத் துன்பம் நேரும் என்றாலோ, அதைப் பேசாதிருத்தல் நலம். அது உண்மையாக இருந்தாலும் உனக்குப் பாவமே நல்கும்” என்று கூறுகிறார்.

வாய்மையைப் பேசுவோம். எந்தச் சொல்லிலும் பொருளை மட்டும் நாடாமல் வாய்மையை நாடுவோம். நமக்கும் பிறருக்கும் ஒரு தீங்கும் இல்லாத நலம் தரும் சொற்களைக் கூறுவோம் என்று கூறி வாய்ப்பளித்தமைக்கு நன்றி கூறி விடைபெறுகிறேன்.

ஆ) மனித நேயம்
Answer:
அனைவருக்கும் வணக்கம்!
மனித நேயம் என்பது பிறர்மீது நாம் காட்டும் அக்கறை, ஆறுதல், நேர்மறை உணர்வு, கருணை உள்ளம், இரக்க குணம், எல்லோருடனும் காட்டும் அன்பு போன்றவற்றின் தொகுப்பே ஆகும். இவற்றை ஒவ்வொருவரும் பெற்றிருத்தல் அவசியம். மனிதநேயம் தன்னலம் அற்ற சேவையாகும்.

நம் உறவினர்களுடன், நண்பர்களுடன் காட்டும் அன்பு, பாசம், கருணை போன்றவை மனிதநேயமாகாது. எந்தத் தொடர்பும் இல்லாதவரின் துன்பத்தைப் பொறுக்காமல் இரக்கப்படுகிறோமே அதுவே மனித நேயம்.

அன்னை தெரசாவைக் கொண்டாடுகிறோம். எதற்காக? அவர் சுயநலமற்றவராய் வாழ்ந்ததால் தான். அவர் வாழ்க்கையில் கண்டவை, தியாகம், அறம், மனிதநேயம், ஏழை எளியவர்மீது அக்கறை, கருணை, மதநல்லிணக்கம், விட்டுக்கொடுத்தல் ஆகிய ஒட்டுமொத்த உயர்பண்புகள்தாம்.

மனிதநேயம் என்பது மனிதன், மனிதன் மேல் மட்டும் கொள்ளும் நேயம் என்றல்லாமல் – சற்றே விரிந்த பார்வையோடு இயற்கையின் மாபெரும் படைப்பான மனிதன், தன்னைப் படைத்த இயற்கை முதல் தான் படைத்த விஞ்ஞானம் ஈறாக அனைத்தினிடமும் காட்டுவதே மனிதநேயம் ஆகும் என்பதை உணர வேண்டும். அதற்கு உதாரணமாகத் திகழ்ந்தவர் இராமலிங்க அடிகள் ஆவார்.

மனித நேயத்திற்கு இவரைவிட வேறு யாரையும் எடுத்துக்காட்டாகக் காட்ட இயலாது. – ஏனெனில் இவர் “வாடிய பயிரைக் கண்ட போதெல்லாம் வாடினேன் என்று ஓரறிவு * உயிரிடமும் இரக்கம் காட்டியவர். இவர் சாதி, மதம், மொழி, தேசம் போன்ற அனைத்து வேற்றுமைகளுக்கும் அப்பாற்பட்டு அனைத்து உயிர்களும் இன்புற்று வாழ்க! கொல்லா நெறியே குருவருள் நெறி என்றார். அனைத்து உயிர்களும் இறைவனின் குழந்தைகள், அவற்றைச் சமமாக பாவிப்பதும் கருணையால் உபசரிப்பதும் மட்டுமே திருவருளுக்கு உகந்தது என்றார்.

ஒருநாளில் இருபது மணி நேரம் பிறருக்காகவே வாழ்ந்தவர் புளோரன்ஸ் நைட்டிங்கேல். இவர் கை விளக்கேந்திய காரிகை’ என்று அழைக்கப்படுபவர். இவர் தமது 31 ஆவது வயதில் தனது குடும்பத்தின் செல்வத்தையும் சுகபோகங்களையும் துறந்து, நோயாளிகளையும் போரில் காயமடைந்தவர்களையும் கவனித்துக் கொள்ளும் தமது நீண்ட நாள் விருப்பதை நிறைவேற்றிக்கொள்ள புறப்பட்டவர். இவர்களெல்லாம் மனித நேயத்தைத் தம்முடையதாக்கிக் கொண்டவர்கள். நாமும் இவர்களைப் போல் மனித நேயத்துடன் வாழ்ந்து மனிதம் காப்போம்.

இ) உதவி செய்து வாழ்தல்
Answer:
அனைவருக்கும் வணக்கம்!
நான் உதவி செய்து வாழ்தல் பற்றிப் பேச வந்துள்ளேன். உலகில் தோன்றும் அனைத்து உயிர்களும் ஒன்றையொன்று சார்ந்து வாழ்கின்றன. மரம், செடி, கொடிகள், பறவைகள், விலங்குகள் எல்லாம் அவ்வாறே வளர்கின்றன. இவை நமக்கு உதவி செய்து வாழ்தலை உணர்த்துகிறது.

பிறருடைய உதவி தமக்குத் தேவையில்லை என்று யாராலும் தனித்து வாழ இயலாது. ஏதாவது ஒரு காலக்கட்டத்தில் பிறரது உதவியை மனிதன் நாடித்தான் ஆகவேண்டும். இவ்வாறு உதவி செய்வதை நன்றி என்கிறோம்.

பிறரிடம் உதவியைப் பெற்றுக் கொண்டு நாம் யாருக்கும் உதவாமல் இருக்கக்கூடாது. நாம் நம்மால் இயன்றவரை பிறருக்கு உதவி செய்ய வேண்டும். நாம் கார்மேகம் போல கைம்மாறு கருதாமல் உதவி செய்ய வேண்டும். தோண்டத் தோண்ட சுரக்கும் நீர் போல, நாம் பிறருக்கு உதவி செய்யச் செய்ய நமக்கு உதவிகள் கிட்டும். இதனை உணர்த்தும் பழமொழி “முன்கை நீண்டால் முழங்கை நீளும்”.

நாம் மரம், செடி, கொடிகளை நீர் ஊற்றி உரமிட்டு வளர்க்கிறோம். அது வளர்ந்து நமக்குப் பல பயன்களைத் தரும். பிறரால் நமக்கு நன்மைகள் கிடைக்கிறதோ இல்லையோ நாம் உதவி செய்தலை வாழ்க்கையின் ஒரு பகுதியாகக் கை கொண்டு வாழ வேண்டும்.

அது மட்டுமின்றி நாம் எவருக்காவது உதவி செய்தால் அதனை மறந்துவிட வேண்டும். தான் செய்த உதவியைப் பலரிடம் கூறிப் பெருமை தேடிக் கொள்ளக் கூடாது. வலது கை கொடுப்பது இடது கைக்குத் தெரியக்கூடாது.

செல்வத்துப் பயனே ஈதல்’ என்பதை உணர வேண்டும். நாம் பெற்ற செல்வத்தைப் பிறருக்குக் கொடுப்பதால் குறைந்து விடுமே என எண்ணாமல் கொடுத்து உதவ வேண்டும். அவ்வாறு செய்யவில்லை எனில் துன்பப்படுவர் என்று புறநானூறு கூறுகிறது.

ஒரு சிலர் தானும் யாருக்கும் உதவி செய்ய மாட்டார்கள். உதவி செய்பவரையும் செய்யவிடாமல் தடுத்து விடுவார்கள். இவ்வாறு இருக்காமல் நாம் நம்மால் இயன்றவரை உதவி செய்து வாழ்வில் உயர்வோம்.

சொல்லக் கேட்டு எழுதுக

1. மனித வாழ்க்கையில் தேவைப்படுவது பொறுமை.
2. குயில் குளிரில் நடுங்கியது; மழையில் ஒடுங்கியது; வெயிலில் காய்ந்தது.
3. இரக்கம் உடையோர் அருள்பெற்றவர் ஆவர்.
4. காயிதே மில்லத் என்னும் சொல்லுக்குச் “சமுதாய வழிகாட்டி” என்று பொருள்.
5. விடியும் போது குளிரத் தொடங்கிவிட்டது.

கீழ்க்காணும் தலைப்புகளில் ஒன்றைத் தேர்ந்தெடுத்துக் கவிதை எழுதுக

சரியான இணைப்புச் சொல்லால் நிரப்புக.

(எனவே, ஏனெனில், அதனால், ஆகையால், அதுபோல, இல்லையென்றால், மேலும் ) (எ.கா) காயிதே மில்லத் அவர்கள் மகிழுந்தில் பயணம் செய்வதை விரும்பாதவர்.

ஏனெனில் அவர் எளிமையை விரும்பியவர்.

Question 1.
நாம் இனிய சொற்களைப் பேச வேண்டும்………………. துன்பப்பட நேரிடும்.
Answer:
இல்லையென்றால்

Question 2.
குயிலுக்குக் கூடு கட்டத் தெரியாது ………………… காக்கையின் கூட்டில் முட்டையிடும்.
Answer:
அதனால்/ஆகையால்

Question 3.
அதிக அளவில் மரங்களை வளர்ப்போம்……………. மரங்கள்தான் மழைக்கு அடிப்படை.
Answer:
ஏனெனில்

Question 4.
பிறருக்குக் கொடுத்தலே செல்வத்தின் பயன் ………….. பிறருக்குக் கொடுத்து மகிழ்வோம்.
Answer:
எனவே

Question 5.
தமிழகத்தில் மழை பெய்துவருகிறது ………………. இரண்டு நாட்கள் மழை நீடிக்கும் என வானிலை ஆய்வுமையம் அறிவிப்பு.
Answer:
மேலும்

கடிதம் எழுதுக.

உங்கள் ஊரில் நடைபெறும் திருவிழாவைக் காணவருமாறு அழைப்பு விடுத்து உறவினர் ஒருவருக்குக் கடிதம் எழுதுக.
Answer:

அஸ்தம்பட்டி, சேலம்
20-12-2019

அன்புள்ள மாமா,
இங்கு நாங்கள் அனைவரும் நலமாக இருக்கிறோம். அங்கு உங்கள் நலத்தையும் அத்தையின் நலத்தையும் அறிய ஆவலாக இருக்கிறேன்.

நம் ஊரில் வருகின்ற மாட்டுப் பொங்கலன்று ஏறுதழுவுதல்’ என்ற நம் பண்பாட்டு – நிகழ்வான காளையை அடக்குதல் விழா வெகு சிறப்பாகக் கொண்டாட ஏற்பாடு – செய்யப்பட்டுள்ளது. தென்மாவட்டங்களில் நடைபெறுவதைப் போன்றே இங்கும் இவ்விழா தி ஆண்டுதோறும் கொண்டாடப்படுவதை நீங்கள் அறிவீர்கள். நீங்கள், அத்தையுடன் அடுத்த மாதம் இவ்விழாவைக் காண இங்கு வரவேண்டும் என்று விரும்புகிறேன். அப்பாவும், அம்மாவும் உங்கள் வருகையை ஆவலுடன் எதிர்நோக்குகிறார்கள். ஆகவே மற்றவை நேரில் .

இங்ஙனம்,
உங்கள் அன்புள்ள ,
ம. இளங்குமரன்.

மொழியோடு விளையாடு

குறிப்புகளைப் பயன்படுத்தி இடமிருந்து வலமாகக் கட்டங்களை நிரப்புக.

1. நூலகத்தில் இருப்பவை…………………………. நூல்கள். நூல்கள் நிறைந்துள்ள இடம் ……………………
2. உலகப்பொதுமறை ………………………. புரட்சிக்கவிஞர் ……………………………..
3. முனைப்பாடியார் இயற்றியது …………………. நீதிநெறி விளக்கம் பாடியவர் குமரகுருபரர்.
4. குற்றால மலைவளத்தைக் கூறும் நூல் ………………………………………………. சுரதா என்பதன் விரிவாக்கம்  ………………….
5. குற்றாலக் குறவஞ்சி பாடியவர்  ………………….
Answer:

1. நூலகத்தில் இருப்பவை. நூல்கள். நூல்கள் நிறைந்துள்ள இடம் நூலகம்.
2. உலகப்பொதுமறை திருக்குறள். புரட்சிக்கவிஞர் பாரதிதாசன்
3. முனைப்பாடியார் இயற்றியது அறநெறிச்சாரம். நீதிநெறி விளக்கம் பாடியவர் குமரகுருபரர்.
4. குற்றால மலைவளத்தைக் கூறும் நூல் குற்றாலக் குறவஞ்சி. சுரதா என்பதன் விரிவாக்கம் சுப்பிரமணியதாசன்.
5. குற்றாலக் குறவஞ்சி பாடியவர் திரிகூடராசப்பக் கவிராயர்.

கீழ்க்காணும் படங்களைப் பார்த்து இரட்டைக்கிளவி அமையுமாறு தொடர்
உருவாக்குக.

Answer:

எ.கா : மழை சட சடவெனப் பெய்தது.

1. பறவைகள் படபட என பறந்தன.
2. ரயில் தடதட என ஓடியது.
3. மரக்கிளை மடமட என முறிந்தது

தீ விபத்தும் பாதுகாப்பு முறைகளும்

வினாக்கள் :

Question 1.
தீ விபத்தினால் பெரிய பாதிப்பு ஏற்படாமல் இருப்பதற்கு, செய்ய வேண்டிய முன்னெச்சரிக்கை நடவடிக்கைகள் யாவை?
Answer:
முன்னெச்சரிக்கை நடவடிக்கைகள் :

• வீடுகளிலும் பொது இடங்களிலும் தீ பாதுகாப்புக் கருவிகள் பொருத்தப்பட்டிருக்க வேண்டும்.
• பொது இடங்களில் தீத்தடுப்பு எச்சரிக்கை ஒலிப்பான்கள் பொருத்தப்பட்டிருக்க வேண்டும்.
• எச்சரிக்கை ஒலி எவ்வாறு இருக்கும் என்பதைப் பணியாளர்கள் அறிந்திருக்க வேண்டும்.
• தரமான மின் சாதனங்களையே பயன்படுத்த வேண்டும்.
• சமையல் செய்யும் போது இறுக்கமான ஆடைகளை உடுத்திக் கொள்ள வேண்டும்.
• பட்டாசுகளைப் பாதுகாப்பான இடங்களில், பெரியவர்களின் மேற்பார்வையில் தான் வெடிக்க வேண்டும்.
• நிறுவனங்களில் பணிபுரியும் பணியாளர்களுக்குத் தீத்தடுப்புப் பயிற்சிகள் அளிக்கப்பட வேண்டும்.
• பொதுமக்கள் கூடும் இடங்களில் தீ விபத்து ஏற்பட்டால் உடனடியாக வெளியேறும் வகையில் அவசரகால வழிகள் அமைக்கப்பட்டிருக்க வேண்டும்.

Question 2.
தீ விபத்து ஏற்பட்டால் உடனடியாகச் செய்ய வேண்டிய செயல்கள் யாவை?
Answer:

• உடனடியாகத் தீயணைப்பு மீட்புப் பணித் துறைக்குத் தொலைபேசி வழியாகத் தகவல் தெரிவிக்க வேண்டும்.
• அவ்வாறு தகவல் தெரிவிக்கும் பொழுது தீ விபத்து ஏற்பட்டுள்ள இடத்தினைத் தெளிவாகத் தெரிவிக்க வேண்டும்.
• தீ விபத்து ஏற்பட்டு இருக்கும் பகுதியில் உடனடியாக மின் இணைப்பைத் துண்டிக்க வேண்டும்.
• தீ விபத்து ஏற்பட்ட உடனே அங்குள்ள தீயணைப்பான்களைக் கொண்டு ஆரம்பக் கட்டத்திலேயே தீயை அணைக்க முயற்சி செய்ய வேண்டும்.
• உடுத்தியிருக்கும் ஆடையில் தீப்பிடித்தால் உடனே தரையில் படுத்து உருள வேண்டும் தீக்காயம் பட்ட இடத்தை உடனடியாகத் தண்ணீரைக் கொண்டு குளிர வைக்க வேண்டும்.
• பொது இடங்களில் தீ விபத்து ஏற்பட்டால் பதற்றமடைந்து ஓடாமல், அவசரகால வழியில் வெளியேற வேண்டும்.
• அருகில் இருக்கும் கட்டடங்களுக்குத் தீ பரவாமல் இருக்கும் வகையில் முன்னெச்சரிக்கை செய்ய வேண்டும்.

Question 3.
பொது இடங்களில் தீ விபத்து ஏற்பட்டால் வெளியேறும் முறையைக் கூறுக.
Answer:
பொது இடங்களில் தீ விபத்து ஏற்பட்டால் பதற்றமடைந்து ஓடாமல், அவசரகால வழியில் வெளியேற வேண்டும்.

Question 4.
தீ விபத்து ஏற்பட்டால் செய்யக்கூடாதவை யாவை?
Answer:

• தீ விபத்து ஏற்பட்டுள்ள கட்டடத்தின் மின் தூக்கியைப் பயன்படுத்தக்கூடாது.
• எண்ணெய் உள்ள இடத்தில் ஏற்பட்ட தீயை அணைக்கத் தண்ணீரைப் பயன்படுத்தக் கூடாது.
• தீக்காயம் பட்ட இடத்தில் எண்ணெய், பேனா மை போன்றவற்றைத் தடவக் கூடாது.

Question 5.
உடலில் தீப்பற்றினால் செய்ய வேண்டிய முதலுதவி யாது?
Answer:

• உடலில் தீப்பற்றினால் தண்ணீரை ஊற்றி அணைக்க வேண்டும். அப்பொழுதுதான் அந்த இடம் குளிர்ச்சி அடையும்.
• பிறகு துணியைக் கொண்டு தீக்காயம் அடைந்த இடத்தை மூடவேண்டும்.
• தண்ணீர் இல்லாவிட்டால் கம்பளி, கோணி போன்றவற்றால் மூடி தீயை அணைக்க வேண்டும். தீக்காயம் அடைந்தவருக்குத் தாகம் இருந்தால் தண்ணீர் கொடுக்கலாம்.
• பதற்றம் அடையாமல் உடனடியாக கீழே விழுந்து புரள வேண்டும். (மண் தரையாக இருந்தால் சிறப்பு)

நிற்க அதற்குத் தக

என் பொறுப்புகள்…

1. நான் எப்போதும் எளிமையைக் கடைப்பிடிப்பேன்
2. அனைவரிடமும் அன்புடன் நடந்து கொள்வேன்.
3. என் பணிகளை நேர்மையாகச் செய்வேன்.
4. நான் என்றும் பொறுமையுடன் இருப்பேன்.

கலைச்சொல் அறிவோம்