Samacheer Kalvi 11th Maths Solutions Chapter 7 Matrices and Determinants Ex 7.2

You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 7 Matrices and Determinants Ex 7.2

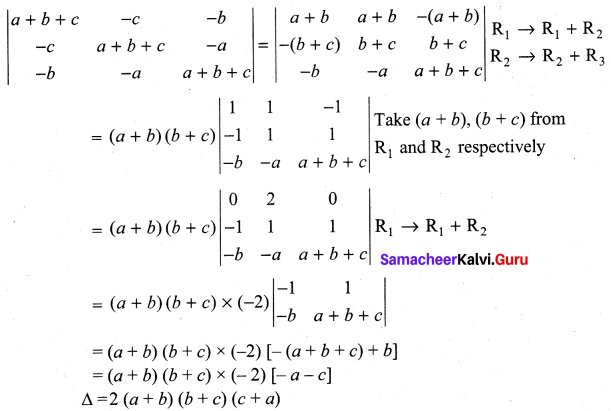
11th Maths Exercise 7.2 Question 1.
Without expanding the determinant, prove that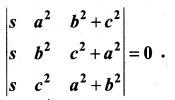
Solution:

11th Maths Exercise 7.2 Answers Question 2.
Show that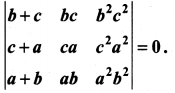
Solution:

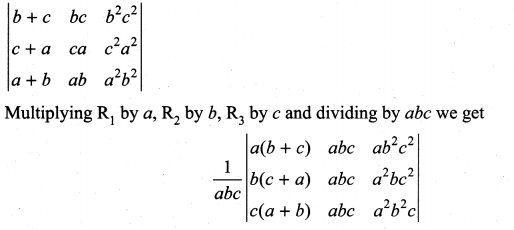
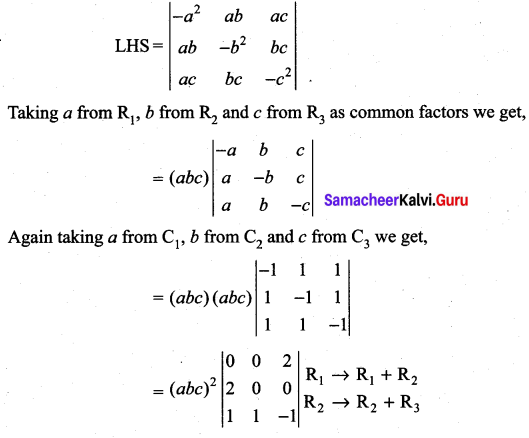
Matrices And Determinants Class 11 Solutions Pdf Question 3.
Prove that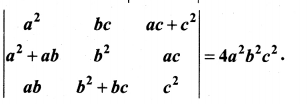
Solution:
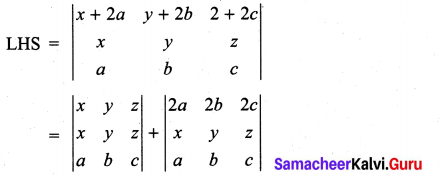
LHS
Taking a from C₁, b from C₂ and c from C₃ we get

Expanding along R₁ we get
(2c) (abc) (1) [ab + ab] = abc (2c) (2ab)
1 = (abc) (4abc) = 4a²b²c²
= RHS

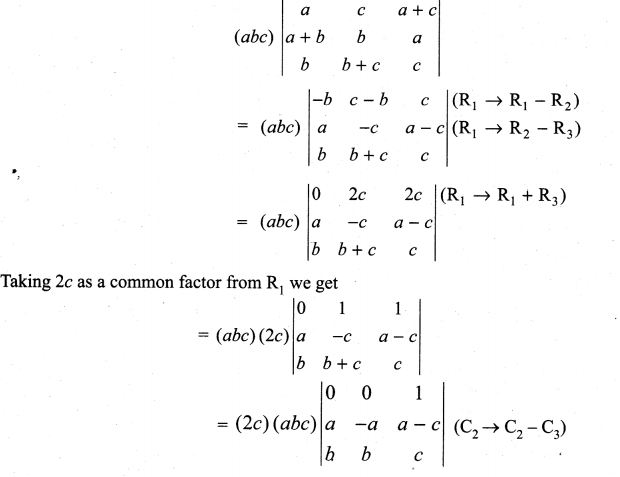
Matrices And Determinants Class 11 State Board Solutions Question 4.

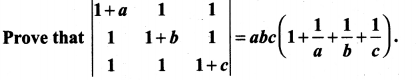
Solution:

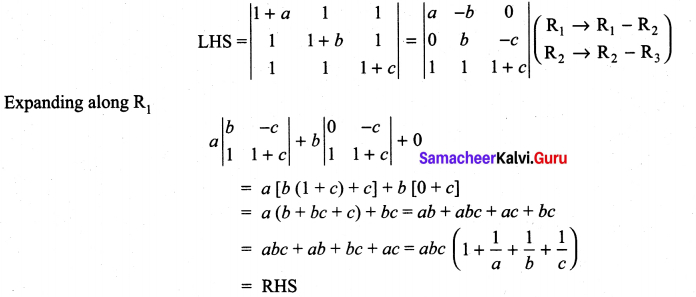
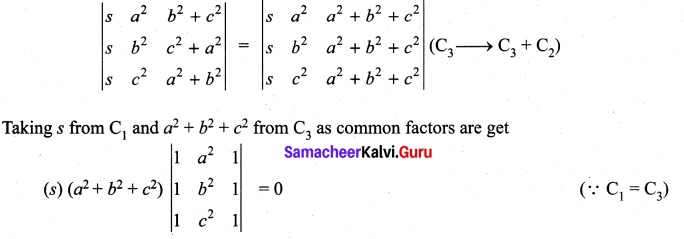
11th Maths Matrices And Determinants Solutions Question 5.
Prove that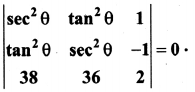
Solution:

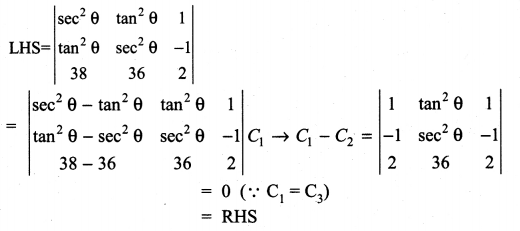
11th Maths Exercise 7.2 In Tamil Question 6.
Show that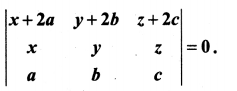
Solution:

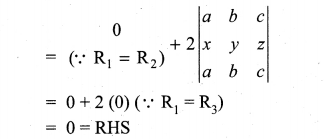
11th Maths Determinants Solutions Question 7.
Write the general form of a 3 × 3 skew-symmetric matrix and prove that its determinant is 0.
Solution:

11th Maths Exercise 7.2 Samacheer Kalvi Question 8.

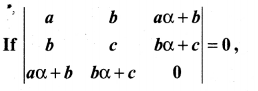
Solution:

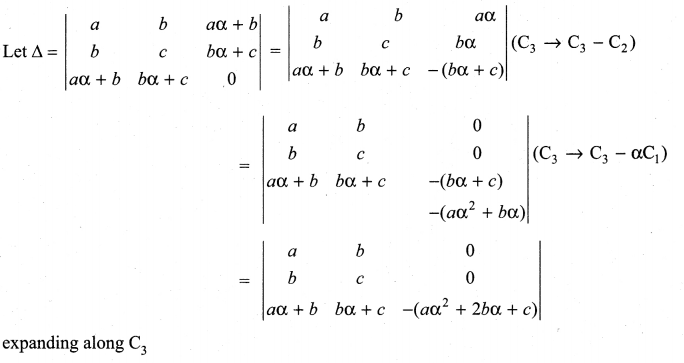
we get – (aα² + 2bα + c) [ac – b²]
So Δ = 0 ⇒ (aα² + 2bα + c) (ac -b²) = – 0 = 0
⇒ aα² + 2bα + c = 0 or ac – b² = 0
(i.e.) a is a root of ax² + 2bx + c = 0
or ac = b²
⇒ a, b, c are in G.P.

11th Maths Matrix Solutions Question 9.
Prove that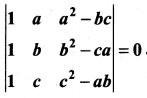
Solution:

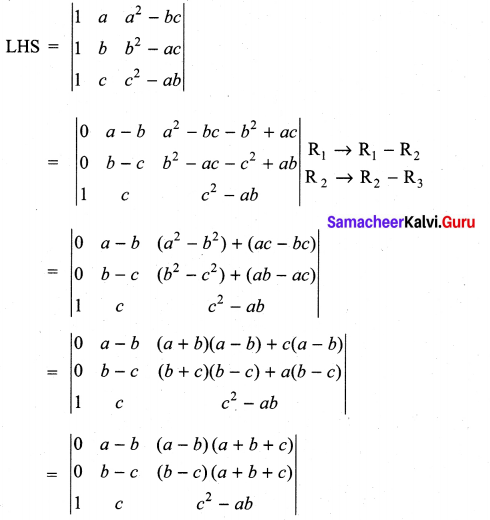
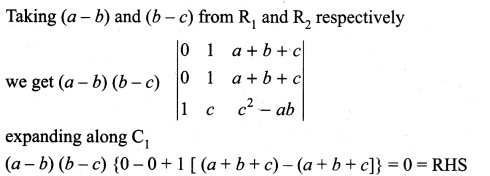
Exercise 7.2 Class 11 Maths Solutions State Board Question 10.
If a, b, c are p^th, q^th and r^th terms of an A.P., find the value of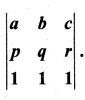
Solution:
We are given a = t_p,b = t_q and c = t_r
Let a be the first term and d be the common difference

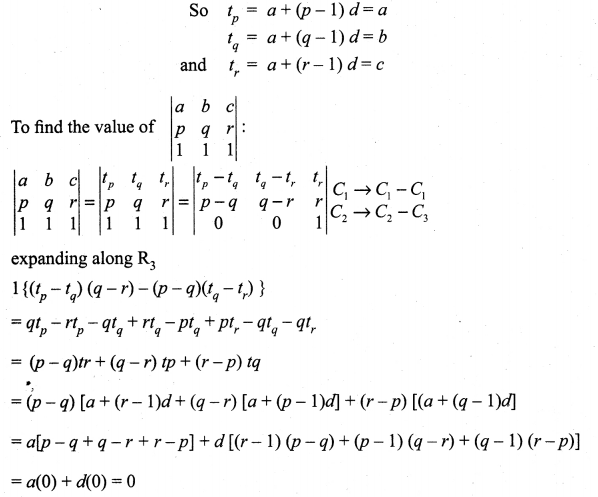
11th Maths Matrix And Determinants Question 11.
Show that 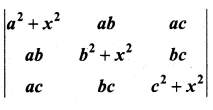is divisible by x⁴
Solution:

Multiplying R₁ by a, R₂ by b and R₃ by c and
taking out a from C₁ b from C₂ and c from C₃ we get
==

11th Maths Ex 7.2 Question 12.
If a, b, c are all positive, and are p^th, q^th and r^th terms of a G.P., show that

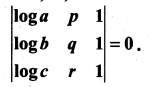
Solution:

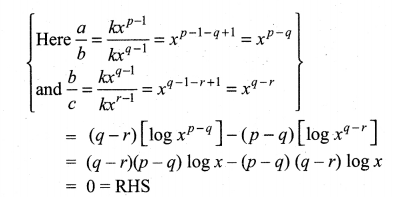
Exercise 7.2 Class 11 Maths Solutions Question 13.
Find the value of 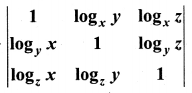if x, y, z ≠ 1.
Solution:
Expanding the determinant along R₁

11th Std Maths Determinants Solutions Question 14.

Solution:

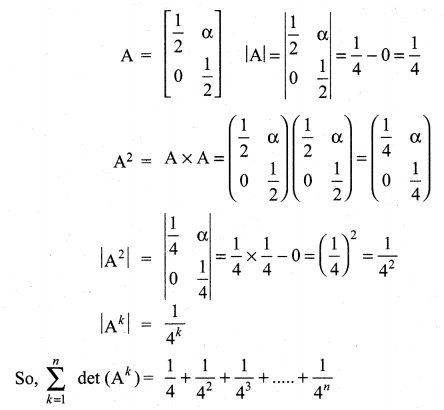
11th Maths Book Volume 2 Chapter 7 Question 15.
Without expanding, evaluate the following determinants:

Solution:

10th Maths Exercise 7.2 Samacheer Kalvi Question 16.
If A is a square matrix and |A| = 2, find the value of |AA^T|.
Solution:
|A| = 2 (Given) |A^T| = 2
Now |AA^T| = |A| |A^T| = 2 × 2 = 4.

12th Maths Exercise 7.2 Samacheer Kalvi Question 17.
If A and B are square matrices of order 3 such that |A| = -1 and |B| = 3, find the value of |3AB|.
Solution:
Given |A| = -1 : |B| = 3
Given A and B are square matrices of order 3.
∴ |kAB| = k³ |AB|
Here k = 3 ∴ |3AB| = 3³ |AB|
= 27 |AB|
= 27 (-1) (3)
= -81

Class 11 Maths Exercise 7.2 Solutions Question 18.
If λ = -2, determine the value of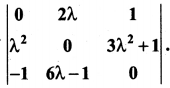
Solution:
Given λ = -2
∴ 2λ = -4; λ² = (-2)²; 3λ² + 1 = 3 (4) + 1 = 13
6λ – 1 = 6(-2) – 1 = -13

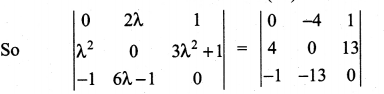
expanding along R₁
0(0) + 4 (0 + 13) + 1 (-52 + 0) = 52 – 52 = 0
Aliter: The determinant value of a skew-symmetric matrix is zero

Matrices And Determinants Class 11 Solutions Question 19.
Determine the roots of the equation
Solution:

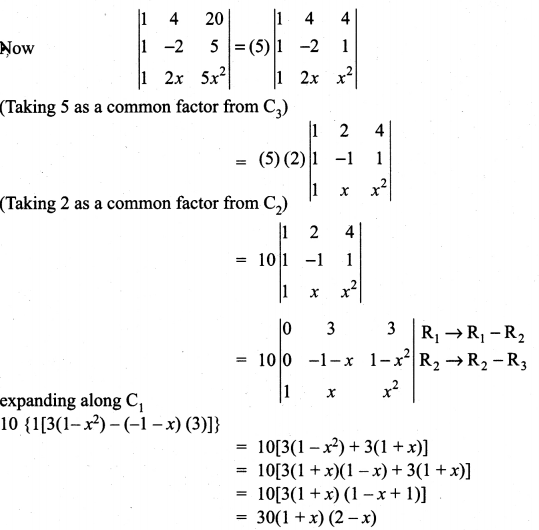
Given the determinant value is 0
⇒ 30(1 + x) (2 – x) = 0
⇒ 1 + x = 0 or 2 – x = 0
⇒ x = -1 or x = 2
So, x = -1 or 2.

Matrices And Determinants Class 11 Exercise Question 20.
Verify that det (AB) = (det A) (det B) for
Solution:


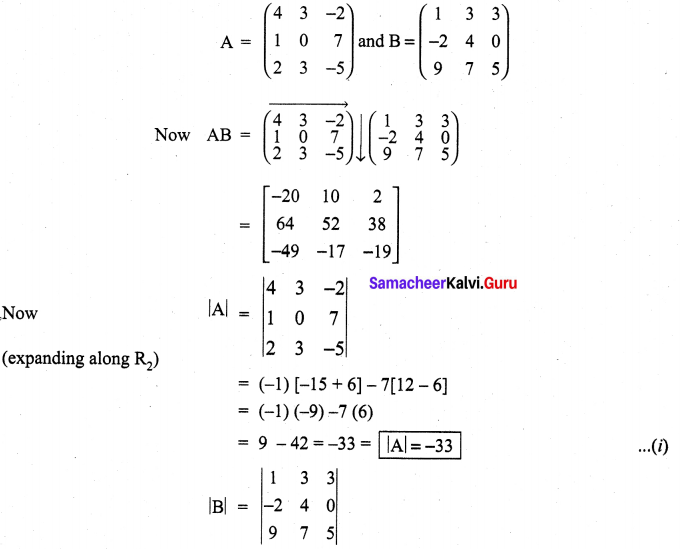
{(-20)(52) (-19) + (10)(38)(—49) + (2)(64)(-17)} – {(-49)(52) (2) + (-17)(38)(-20) + (-19)(64)(10)}
= (19760 – 18620 – 2176) – (-5096 + 12920 – 12160)
= (19760 + 5096 + 12160) – (18620 + 2176 + 12920)
= 37016 – 33716 = 3300 ….(3)
Now (1) × (2) = (3)
(i.e.,) (-33) (-100) = 3300
⇒ det (AB) = (det A), (det B)

Determinants Class 11 State Board Solutions Question 21.
Using cofactors of elements of second row, evaluate |A|, where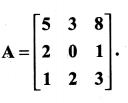
Solution:

Samacheer Kalvi 11th Maths Solutions Chapter 7 Matrices and Determinants Ex 7.2 Additional Problems

Exercise 7.2 Class 10 Samacheer Kalvi Question 1.

Solution:

11th Maths Matrices And Determinants Pdf Question 2.

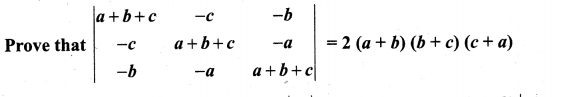
Solution:

Question 3.

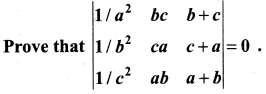
Solution:

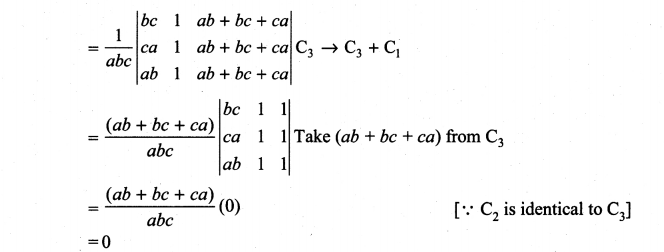
Question 4.

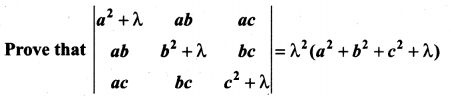
Solution:

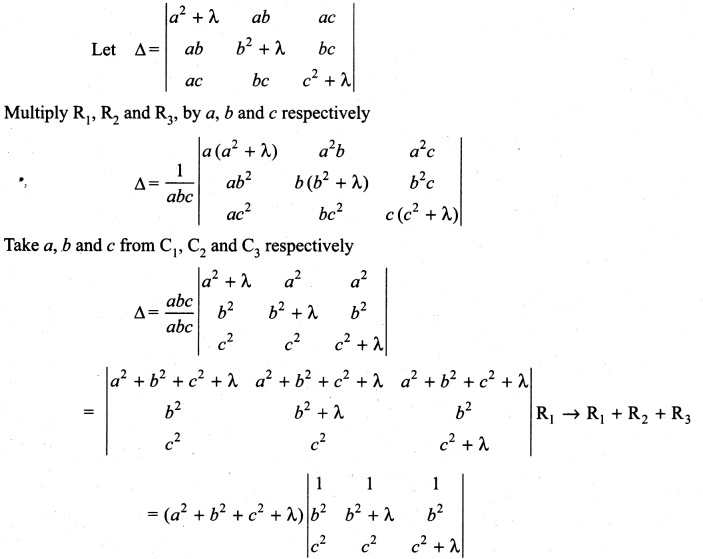
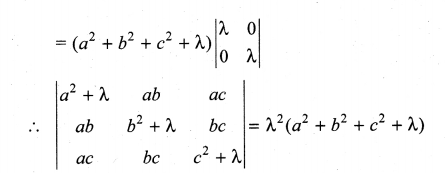
Question 5.

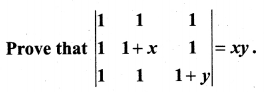
Solution:

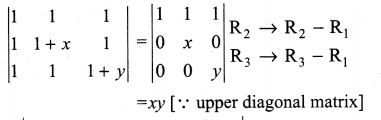
Question 6.

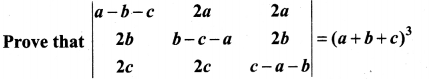
Solution:

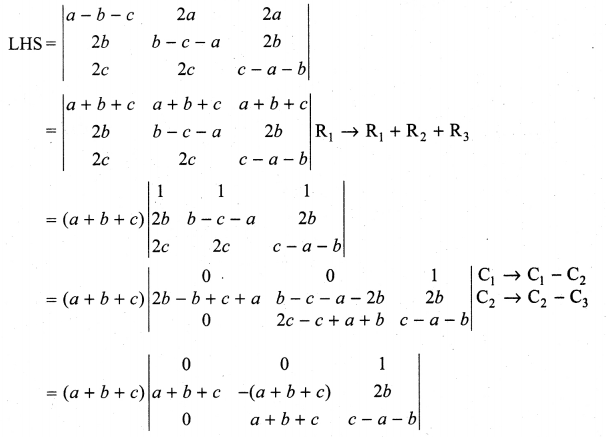
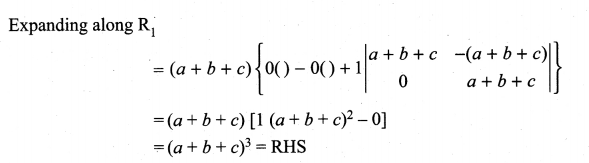
Question 7.

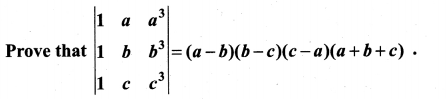
Solution:

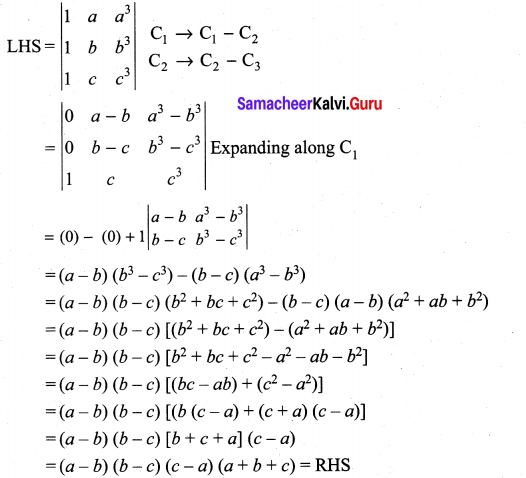
Question 8.

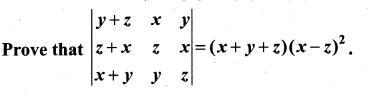
Solution:

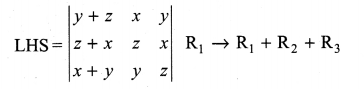
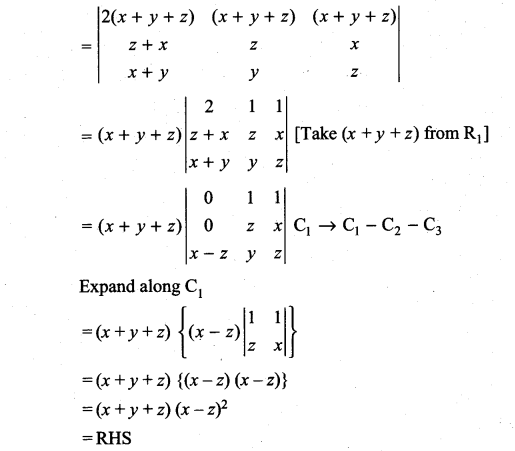
Question 9.

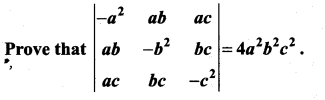
Solution: