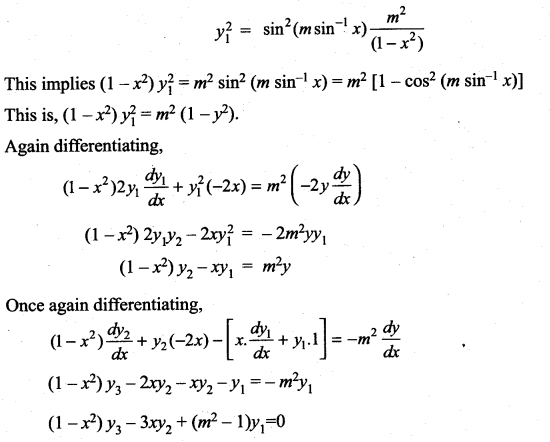Samacheer Kalvi 11th Maths Solutions Chapter 10 Differentiability and Methods of Differentiation Ex 10.4

You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 10 Differentiability and Methods of Differentiation Ex 10.4

Find the derivatives of the following functions
Question 1.
y = x^{cos x}
Solution:
y = x^{cos x}
Taking log on both sides
log y = log x^{cos x} = cos x log x
differentiating w.r.to x we get

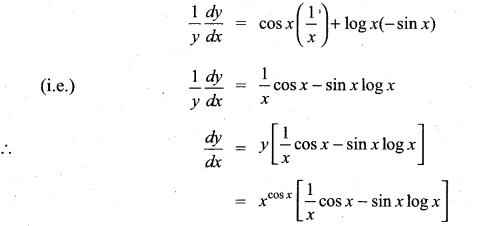
Question 2.
y = x^logx + (logx)^x
Solution:
y = x^logx + (logx)^x
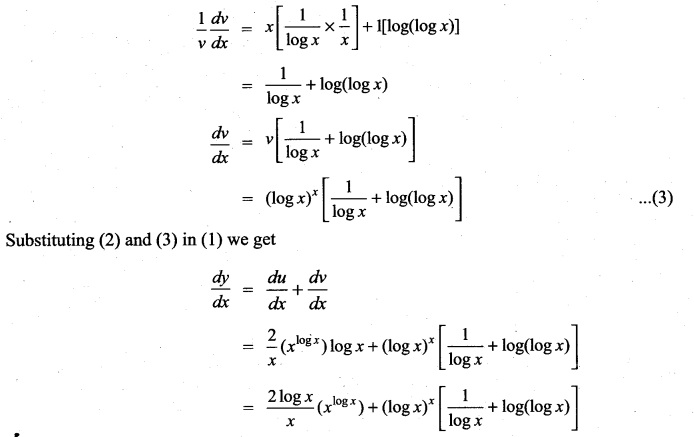
Let y = u + v
Then \(\frac{d y}{d x}=\frac{d u}{d x}+\frac{d v}{d x}\)
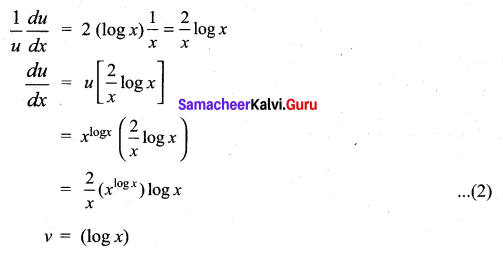
u = x^logx
Taking log on both sides
log u = log x log x = log (x)²
differentiating w.r.to x

Taking log on both sides
log u = log (logx)^x = x log (log x)
differentiating w.r.to x

Question 3.
\(\sqrt{x y}\) = e^{(x – y)}
Solution:

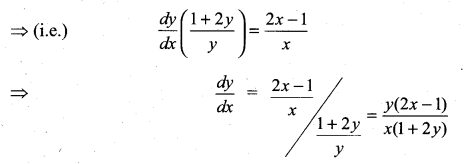
Question 4.
x^y = y^x
Solution:
x^y = y^x
Taking log on both sides
logx^y = logy^x
(i.e.) y log x = x log y
differentiating w.r.to x

Question 5.
(cos x)^{log x}
Solution:
y = (cos x)^{log x}
Taking log on both sides
log y = log (cos x)^{log x} = log x (log cos x)

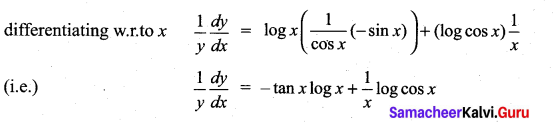
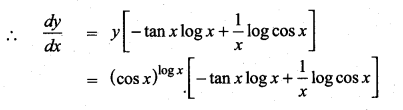
Question 6.
\(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1
Solution:
\(\frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}\) = 1
Differentiating w.r.to x

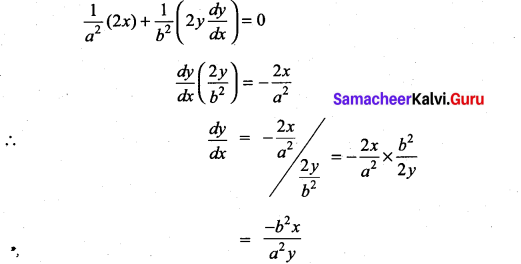
Question 7.
\(\sqrt{x^{2}+y^{2}}=\tan ^{-1}\left(\frac{y}{x}\right)\)
Solution:
\(\sqrt{x^{2}+y^{2}}=\tan ^{-1}\left(\frac{y}{x}\right)\)
Differentiating w.r.to x

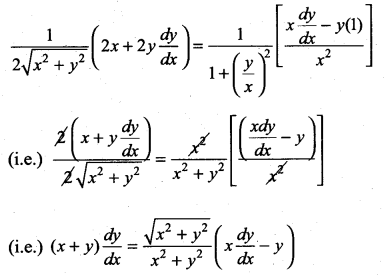
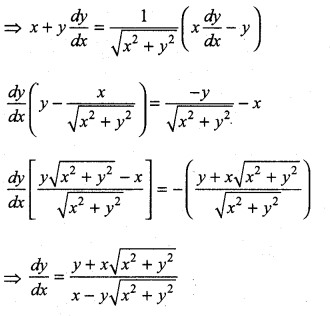
Question 8.
tan (x + y) + tan (x – y) = x
Solution:
tan (x + y) + tan (x – y) = x
Differentiating w.r.to x we get

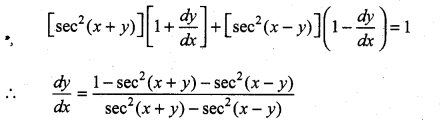
Question 9.
If cos (xy) = x, show that \(\frac{d y}{d x}=\frac{-(1+y \sin (x y))}{x \sin x y}\)
Solution:
cos (xy) = x
Differentiating w.r.to x

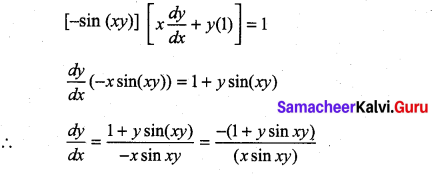
Question 10.
\(\tan ^{-1} \sqrt{\frac{1-\cos x}{1+\cos x}}\)
Solution:

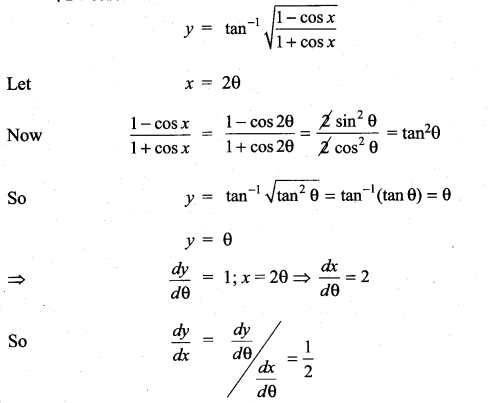
Question 11.
\(\tan ^{-1}\left(\frac{6 x}{1-9 x^{2}}\right)\)
Solution:

Question 12.
cos [2 \(\tan ^{-1} \sqrt{\frac{1-x}{1+x}}\)]
Solution:

Question 13.
x = a cos³t; y = a sin²t
Solution:

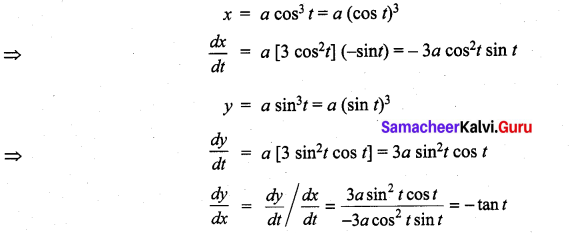
Question 14.
x = a (cos t + t sin t); y = a [sin t – t cos t]
Solution:

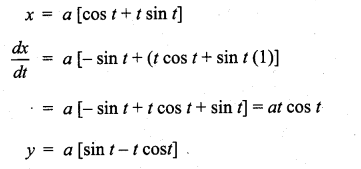
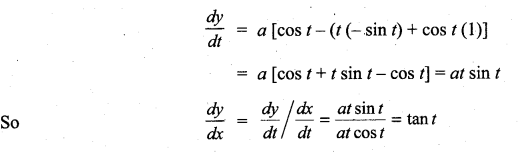
Question 15.
x = \(\frac{1-t^{2}}{1+t^{2}}\); y = \(\frac{2 t}{1+t^{2}}\)
Solution:

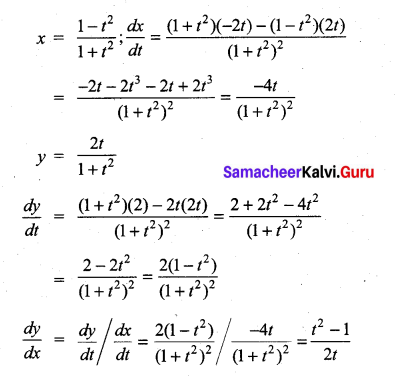
Question 16.
\(\cos ^{-1}\left(\frac{1-x^{2}}{1+x^{2}}\right)\)
Solution:

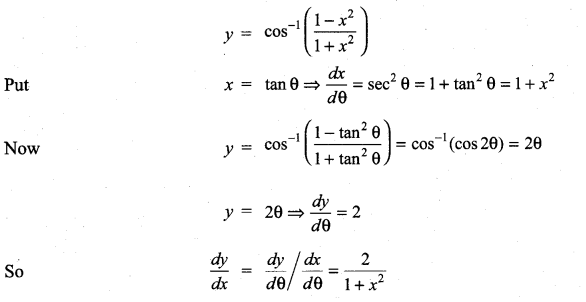
Question 17.
sin^-1 (3x – 4x³)
Solution:

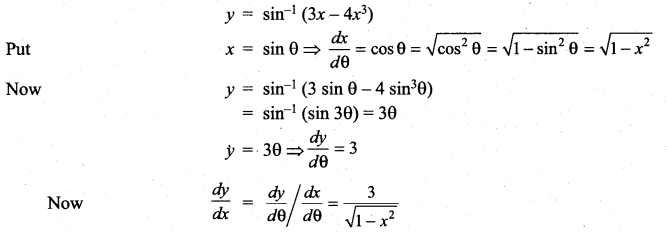
Question 18.
\(\tan ^{-1}\left(\frac{\cos x+\sin x}{\cos x-\sin x}\right)\)
Solution:

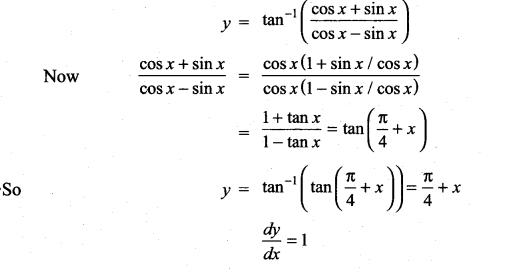
Question 19.
Find the derivative of sin x² with respect to x²
Solution:

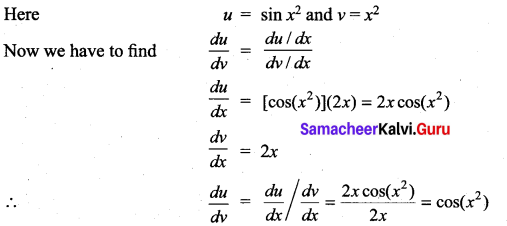
Question 20.
Find the derivative of \(\sin ^{-1}\left(\frac{2 x}{1+x^{2}}\right)\) with respect to tan^-1 x.
Solution:
Let u = \(\sin ^{-1}\left(\frac{2 x}{1+x^{2}}\right)\) and v = tan^-1 x
Now we have to find \(\frac{d u}{d v}\)

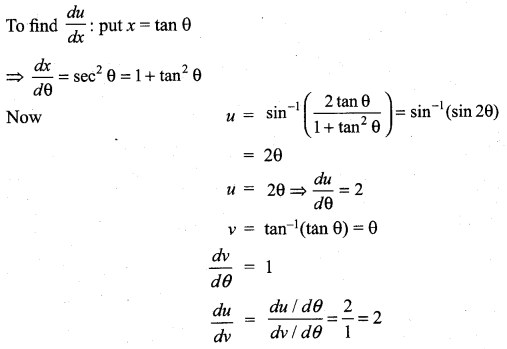
Question 21.

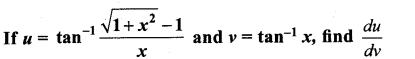
Solution:

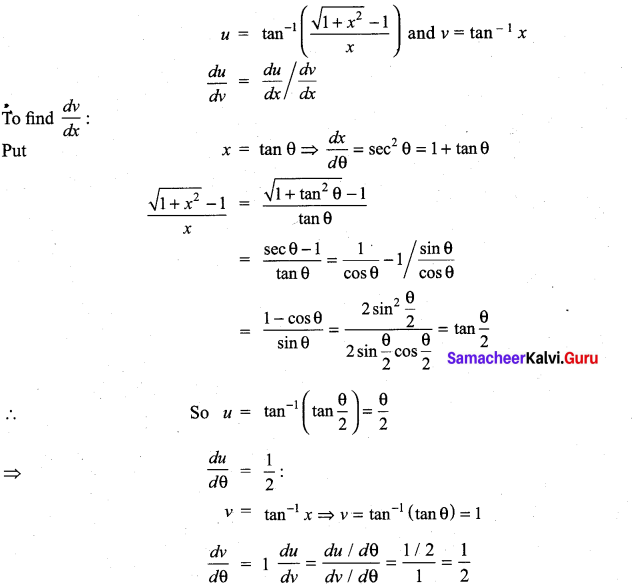
Question 22.
Find the derivative with 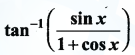with respect to
Solution:

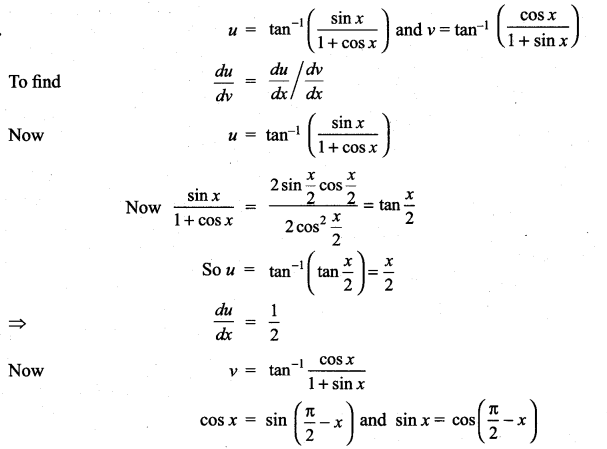
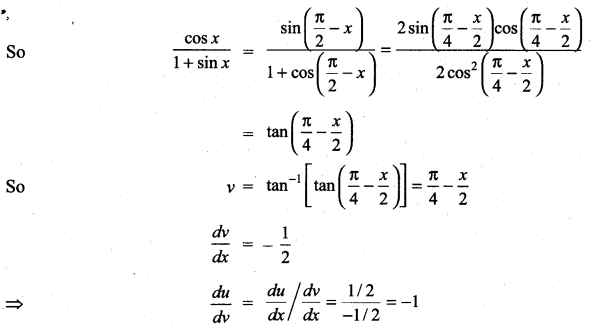
Question 23.
If y = sin^-1 then find y”.
Solution:

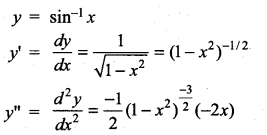
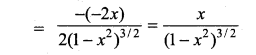
Question 24.
If y = e^tan-1x, show that (1 + x²) y” + (2x – 1) y’ = 0
Solution:
y = e^tan-1x
y = e^tan-1x \(\left(\frac{1}{1+x^{2}}\right)\)
⇒ y’ = \(\frac{y}{1+x^{2}}\) ⇒ y'(1 + x²) = y
differentiating w.r.to x
y’ (2x) + (1 + x²) (y”) = y’
(i.e.) (1 + x²) y” + y’ (2x) – y’ = 0
(i.e.) (1 + x²) y” + (2x – 1) y’ = 0

Question 25.
If y = \(\frac{\sin ^{-1} x}{\sqrt{1-x^{2}}}\) show that (1 – x²) y₂ – 3xy₁ – y = 0
Solution:

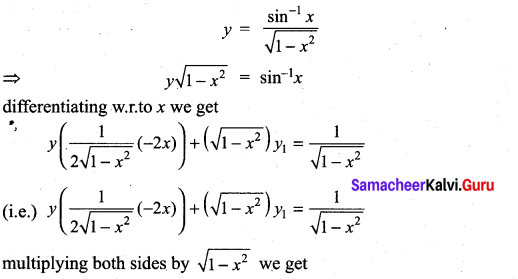
-xy + (1 – x²) y₁ = 1
differentiating both sides again w.r.to x
-[x y₁ + y (1)] + (1 – x²) (y₂) + y₁ (-2x) = 0
(i.e.) -xy₁ – y + (1 – x²) y₂ – 2xy₁ = 0
(1 – x²) y₂ – 3xy₁ – y = 0

Question 26.
If x = a (θ + sin θ), y = a (1 – cos θ) then prove that at θ = \(\frac{\pi}{2}\), y” = \(\frac{1}{a}\)
Solution:

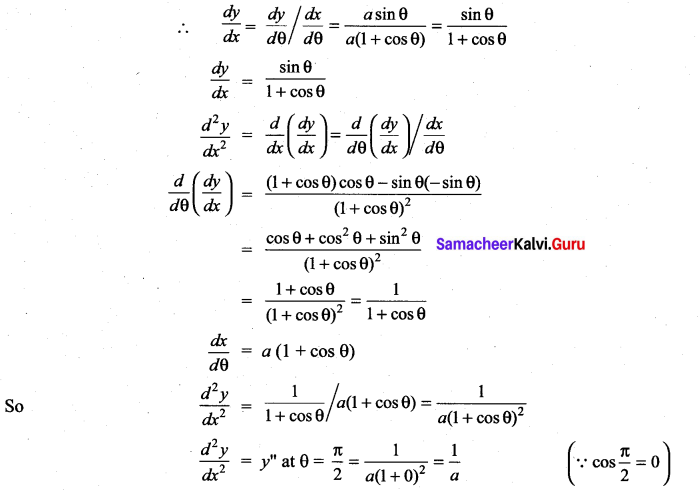
Question 27.
If sin y = x sin (a + y) Then prove that \(\frac{d y}{d x}=\frac{\sin ^{2}(a+y)}{\sin a}\), a ≠ nπ
Solution:

Question 28.
If y = (cos^-1 x)², prove that (1 – x²) \(\frac{d^{2} y}{d x^{2}}-x \frac{d y}{d x}\) – 2 = 0. Hence find y₂ when x = 0.
Solution:

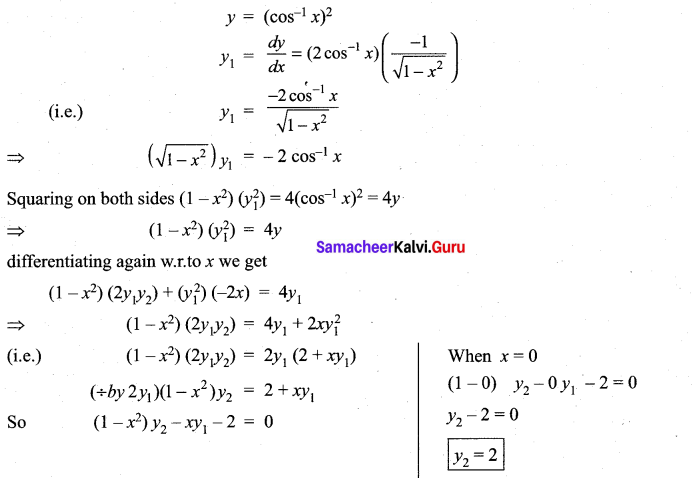
Samacheer Kalvi 11th Maths Solutions Chapter 10 Differentiability and Methods of Differentiation Ex 10.4 Additional Problems

Question 1.
If y = A cos4x + B sin 4x, A and B are constants then Show that y₂ + 16y = 0
Solution:

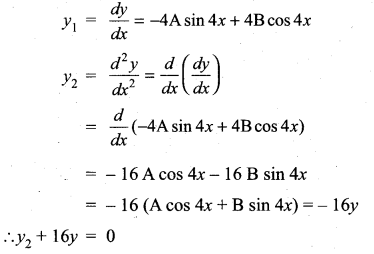
Question 2.
If y = cos (m sin^-1 x), prove that (1 – x²) y₃ – 3xy₂ + (m² – 1) y₁ = 0
Solution:
We have y = cos (m sin^-1 x)
y₁ = sin (m sin^-1x). \(\frac{m}{\sqrt{1-x^{2}}}\)