You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 11 Integral Calculus Ex 11.13
Choose the correct or most suitable answer from given four alternatives.
Question 1.
If \(\int f(x) d x\) = g(x) + c, then \(\int f(x) g^{\prime}(x) d x\)
![]()
Solution:
(a)
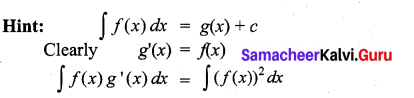
Question 2.
If 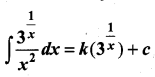 , then the value of k is ……………
, then the value of k is ……………
(a) log 3
(b) -log 3
(c) \(-\frac{1}{\log ^{3}}\)
(d) \(\frac{1}{\log 3}\)
Solution:
(c)
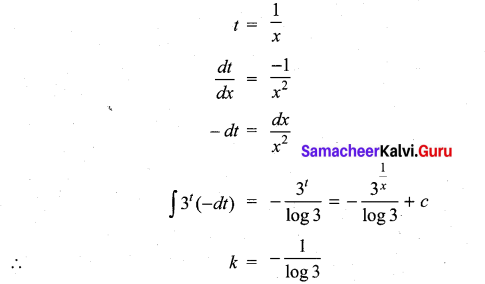
Question 3.
If \(\int f^{\prime}(x) e^{x^{3}} d x\) = (x – 1)ex2, then f(x) is …………………

Solution:
(d)
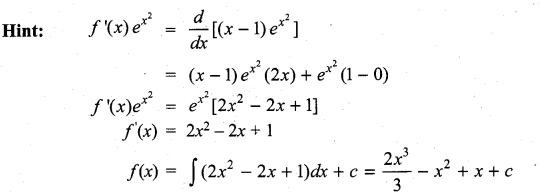
Question 4.
The gradient (slope) of a curve at any point (x, y) is \(\frac{x^{2}-4}{x^{2}}\). If the curve passes through the point(2, 7), then the equation of the curve is ………….
(a) y = x + \(\frac{4}{x}\) + 3
(b) y = x + \(\frac{4}{x}\) + 4
(c) y = x2 + 3x + 4
(d) y = x2 – 3x + 6
Solution:

![]()
Question 5.
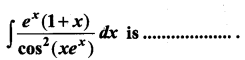
(a) cot (xex) + c
(b) sec (xex) + c
(c) tan (xex) + c
(d) cos (xex) + c
Solution:
(c)
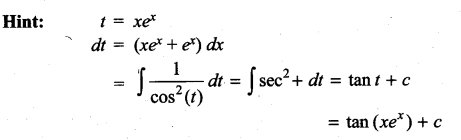
Question 6.
\(\int \frac{\sqrt{\tan x}}{\sin 2 x} d x\) is ……………..
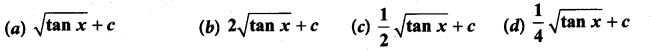
Solution:
(a)

Question 7.
\(\int \sin ^{3} x d x\) is …………….

Solution:
(c)
Hint: sin3x = \(\frac{1}{4}\) (3 sin x – sin 3x)
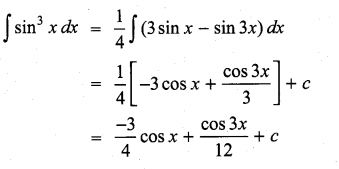
Question 8.
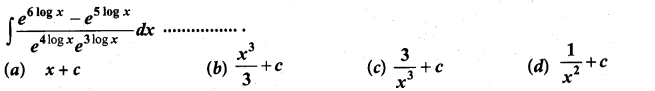
Solution:
(b)
Question 9.
(a) tan-1 (sin x) + c
(b) 2 sin-1 (tan x) + c
(c) tan-1 (cos x) + c
(d) sin-1 (tan x) + c
Solution:
(d)
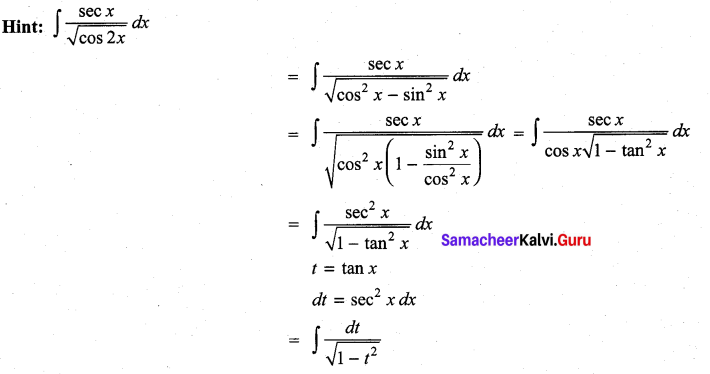
= sin-1 (t) + c
= sin-1 (tan x) + c
![]()
Question 10.

(a) x2 + c
(b) 2x2 + c
(c) \(\frac{x^{2}}{2}\) + c
(d) \(-\frac{x^{2}}{2}\) + c
Solution:
(c)
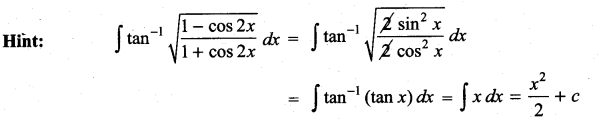
Question 11.
\(\int 2^{3 x+5} d x\) is ……………

Solution:
(d)
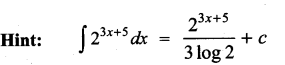
Question 12.
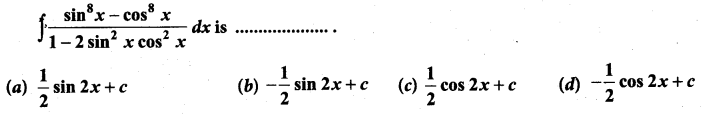
Solution:
(b)
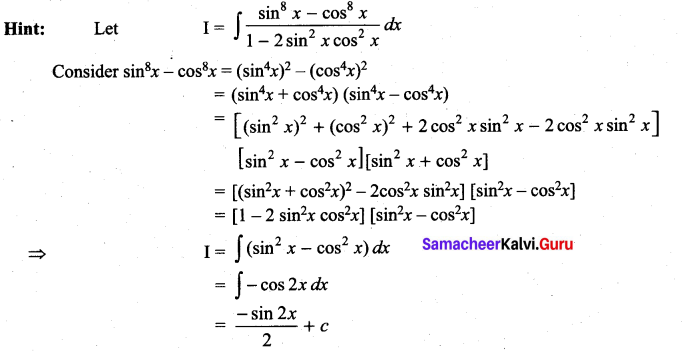
Question 13.
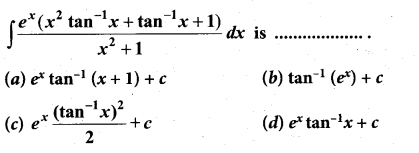
Solution:
(d)
Question 14.
\(\int \frac{x^{2}+\cos ^{2} x}{x^{2}+1}\) cosec2xdx is …………….
(a) cot x + sin-1 x + c
(b) -cot x + tan-1 x + c
(c) -tan x + cot-1 x + c
(d) -cot x – tan-1 x + c
Solution:
(d)

Question 15.
\(\int x^{2} \cos x d x\) is ……………
(a) x2 sin x + 2x cos x – 2 sin x + c
(b) x2 sin x – 2x cos x – 2 sin x + c
(c) -x2 sin x + 2x cos x + 2 sin x + c
(d) -x2 sin x – 2x cos x + 2 sin x + c
Solution:
(a)
Hint: \(\int x^{2} \cos x d x\)
By Bernoullis formula dv = cosxdx
u = x2 v = sinx
u’ = 2x v1 = -cos x
u” = 2 v2 = -sinx
= uv – u’v1 + u”v2
= x2sin x + 2x cos x – 2 sin x + c
![]()
Question 16.
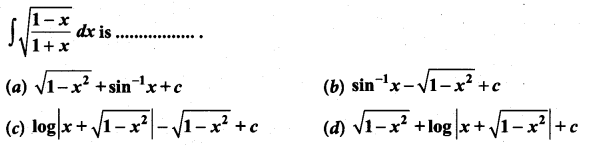
Solution:
(b)
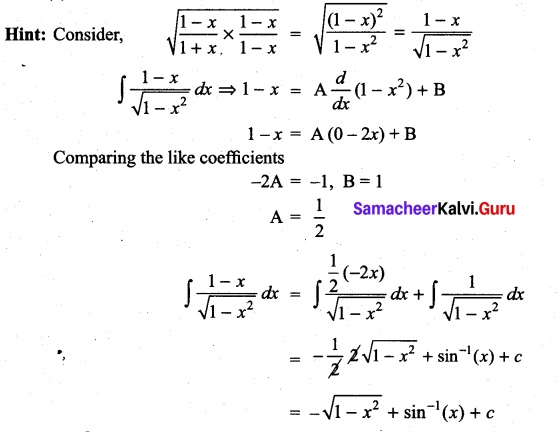
Question 17.
\(\int \frac{d x}{e^{x}-1}\) is …………….
(a) log |ex| – log |ex – 1| + c
(b) log |ex| + log |ex – 1| + c
(c) log |ex – 1| – log |ex| + c
(d) log |ex + 1| – log |ex| + c
Solution:
(c)

Question 18.
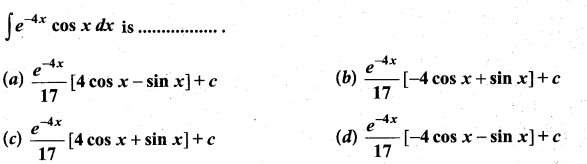
Solution:
(b)
We know that
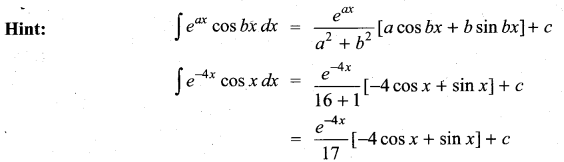
Question 19.

Solution:
(d)
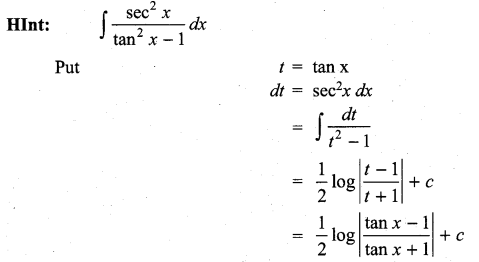
Question 20.

Solution:
(a)
We know that
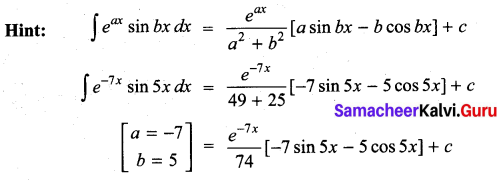
![]()
Question 21.
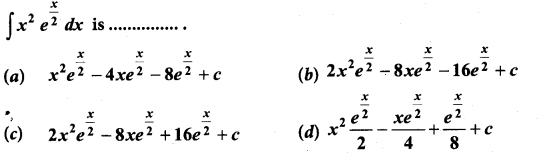
Solution:
(c)
Hint:
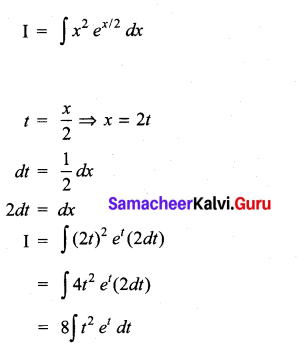
By Bernoullis formula,
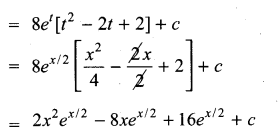
Question 22.
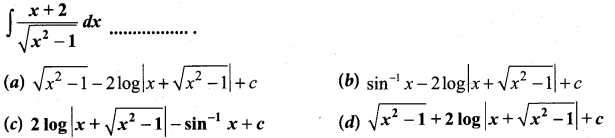
Solution:
(d)
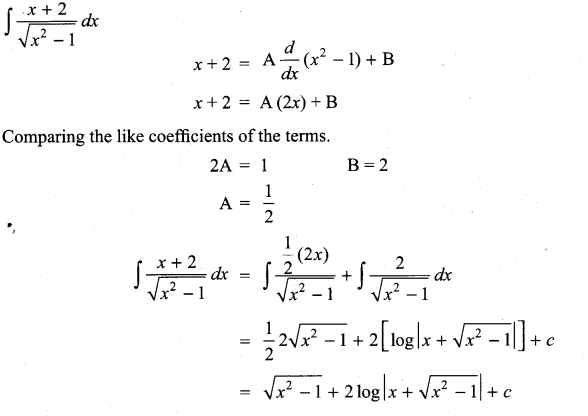
Question 23.
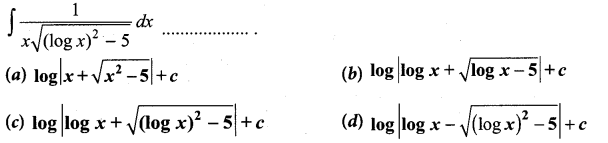
Solution:
(c)
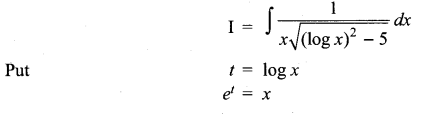
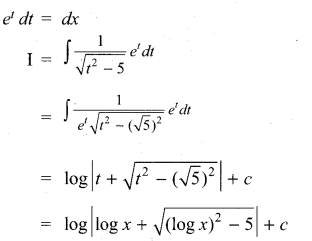
Question 24.
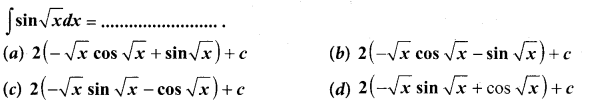
Solution:
(a)
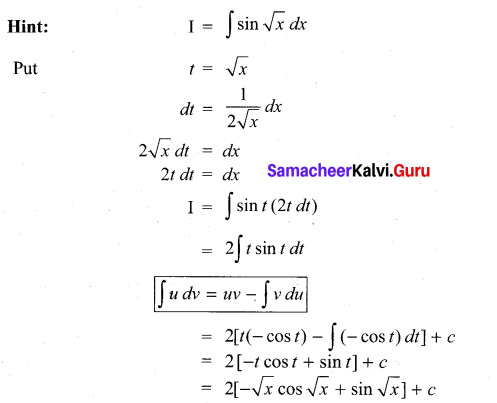
Question 25.
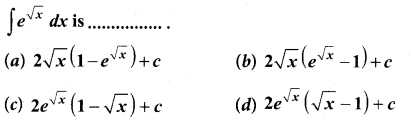
Solution:
(d)
Hint: Let I = \(\int e^{\sqrt{x}} d x\)
t = \(\sqrt{x}\)

Must Follow: