Samacheer Kalvi 8th Maths Solutions Term 3 Chapter 1 Numbers Ex 1.4

Students can Download Maths Chapter 1 Numbers Ex 1.4 Questions and Answers, Notes Pdf, Samacheer Kalvi 8th Maths Book Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 8th Maths Solutions Term 3 Chapter 1 Numbers Ex 1.4

Samacheer Kalvi 8th Maths Book Solutions Exercise 1.4 Question 1.
Fill in the blanks:
(i) (-1 )^{even integer} is…………
(ii) For a ≠ 0, a° is……….
(iii) 4^-3 × 5^-3 =…………
(iv) (-2)⁷ =………….
(v) \((-\frac{1}{3})^{-5}\) =…………
Solution:
(i) 1
(ii) 1
(iii) 20^-3
(iv) \(\frac{-1}{128}\)
(v) -243

Samacheer Kalvi 8th Maths Solutions Term 3 Pdf Question 2.
Say True or False:
(i) If 8^x = \(\frac{1}{64}\), the value of x is -2
(ii) The simplified form of (256)^-1/4 is \(\frac{1}{4}\)
(iii) The standard form of 2 x 10^-4 is 0.0002.
(iv) The scientific form of 123.456 is 1.23456 x 10^-2.
(v) The multiplicative inverse of (3)^-7 is 3⁷.
Solution:
(i) True
(ii) True
(iii) True
(iv) False
(v) True

8th Maths Exercise 1.4 Samacheer Kalvi Question 3.
Evaluate:
(i) \(\left(\frac{1}{2}\right)^{3}\)
Solution:
\(\left(\frac{1}{2}\right)^{3}=\frac{1^{3}}{2^{3}}=\frac{1}{2 \times 2 \times 2}=\frac{1}{8}\)

(ii) \(\left(\frac{1}{2}\right)^{-5}\)
Solution:
\(\left(\frac{1}{2}\right)^{-5}=\frac{1^{-5}}{2^{-5}}=\frac{1}{2^{-5}}=2^{5}\) = 2 × 2 × 2 × 2 × 2 = 32

(iii) (-3)^-3
Solution:
(-3)^-3 = \(\frac{1}{(-3)^{3}}=\frac{1}{-3 \times-3 \times-3}=\frac{1}{-27}=\frac{-1}{27}\)

(iv) (-3)⁴
Solution:
(-3)⁴ = -3 × -3 × -3 × -3 = 81

(v) \(\left(\frac{-5}{6}\right)^{-3}\)
Solution:
\(\left(\frac{-5}{6}\right)^{-3}=\frac{(-5)^{-3}}{6^{-3}}=\frac{6^{3}}{(-5)^{3}}=\frac{6 \times 6 \times 6}{-5 \times-5 \times-5}=-\frac{216}{125}\)

(vi) \(\left( { 2 }^{ -5 }\div { 2 }^{ 7 } \right) \times { 2 }^{ -2 }\)
Solution:
\(\left( { 2 }^{ -5 }\div { 2 }^{ 7 } \right) \times { 2 }^{ -2 }\) = \(\left( { 2 }^{ -5-7 }\right)\times { 2 }^{ -2 }\)
\(2^{-12} \times 2^{-2}=2^{-12+(-2)}=2^{-14}\)

(vii) \(\left( { 2 }^{ -1 }\times { 3 }^{ -1 } \right) \div 6^{ -2 }\)
Solution:
\(\left( { 2 }^{ -1 }\times { 3 }^{ -1 } \right) \div 6^{ -2 }\) = \((2 \times { 3})^{ -1 } \div 6^{ -2 }\)
= \((6)^{-1} \div 6^{-2}=6^{(-1)-(-2)}=6^{1}=6\)

(viii) \(\left(-\frac{1}{3}\right)^{-2}\)
Solution:
\(\left(-\frac{1}{3}\right)^{-2}=\left(-\frac{3}{1}\right)^{2}=\frac{(-3)^{2}}{1^{2}}=\frac{-3 \times-3}{1}\) = 9

Samacheer Kalvi 8th Maths Book Solutions Question 4.
Evaluate:
(i) \(\left(\frac{2}{5}\right)^{4} \times\left(\frac{2}{5}\right)^{2}\)
Solution:
\(\left(\frac{2}{5}\right)^{4} \times\left(\frac{2}{5}\right)^{2}\) = \(\left(\frac{2}{5}\right)^{4+2}=\left(\frac{2}{5}\right)^{6}\)

(ii) \(\left(\frac{4}{5}\right)^{-2} \times\left(\frac{4}{5}\right)^{-3}\)
Solution:
\(\left(\frac{4}{5}\right)^{-2} \times\left(\frac{4}{5}\right)^{-3}\) = \(\left(\frac{4}{5}\right)^{-2+(-3)}=\left(\frac{4}{5}\right)^{-5}\)

(iii) \(\left(\frac{1}{2}\right)^{-3} \times\left(\frac{1}{2}\right)^{7}\)
\(\left(\frac{1}{2}\right)^{-3} \times\left(\frac{1}{2}\right)^{7}\) = \(\left(\frac{1}{2}\right)^{-3+7}=\left(\frac{1}{2}\right)^{4}\)

Samacheer Kalvi Guru 8th Maths Question 5.
Evaluate:
(i) \(\left( { 5 }^{ 0 }+{ 6 }^{ -1 } \right) \times { 3 }^{ 3 }\)
Solution:

(ii) \(\left( 2^{ -1 }\times { 3 }^{ -1 } \right) \div { 6 }^{ -1 }\)
Solution:
\(\left( 2^{ -1 }\times { 3 }^{ -1 } \right) \div { 6 }^{ -1 }\) = \((2 \times 3)^{-1} \div 6^{-1}=6^{-1}+6^{-1}=6^{-1-(-1)}=6^{0}\) = 1

(iii) \(\left( 3^{ -1 }+{ 4 }^{ -2 }+{ 5 }^{ -3 } \right) ^{ 0 }\)
Solution:
\(\left( 3^{ -1 }+{ 4 }^{ -2 }+{ 5 }^{ -3 } \right) ^{ 0 }\) = 1 [ ∴ a° = 1 where a ≠ 0]

Samacheer Kalvi Guru 8th Maths Book Solutions Question 6.
Simplify
(i) \(\left(3^{2}\right)^{3} \times\left(2 \times 3^{5}\right)^{-2} \times(18)^{2}\)
Solution:

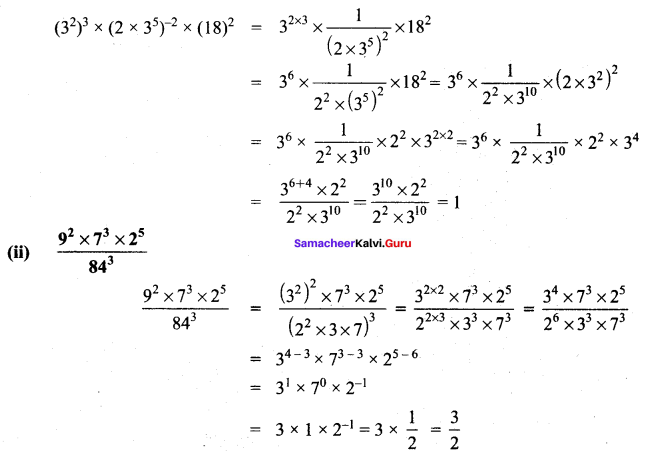
Samacheer Kalvi.Guru 8th Maths Question 7.
Solve for x.
(i) \(\frac{10^{x}}{10^{-3}}=10^{9}\)
Solution:
\(\frac{10^{x}}{10^{-3}}=10^{9}\)
\({10^{x+3}}=10^{9}\)
Equating the powers of same base 10
x + 3 = 9
x + 3 – 3 = 9 – 3
x = 6

(ii) \(\frac{2^{2x-1}}{2^{x+2}}\) = 4
Solution:
\(2^{2x-1-1(x+2)}\) = 2²
\(2^{2x-1-x-2}\) = 2²
\(2^{x-3}\) = 2²
Equating the powers of the same base 2.
x – 3 = 2
x – 3 + 3 = 2 + 3
x = 5

(iii) \(\frac{5^{5} \times 5^{-4} \times 5^{x}}{5^{12}}=5^{-5}\)
Solution:
\(\frac{5^{5} \times 5^{-4} \times 5^{x}}{5^{12}}=5^{-5}\) = \(5^{-5} \Rightarrow \frac{5^{5-4+x}}{5^{12}}=5^{-5}\)
\(\Rightarrow \frac{5^{1+x}}{5^{12}}\) = 5^-5
⇒ \(5^{1+x-12}\) = 5^-5
⇒ \(5^{x-11}\) = 5^-5
Equating the powers of same base 5.
x – 11 = -5
x – 11 + 11 = -5 + 11
x = 6

Samacheer Kalvi Guru 8th Maths Guide Question 8.
Expand using exponents:
(i) 6054.321
(ii) 897.14
Solution:

Samacheerkalvi.Guru 8th Maths Question 9.
Find the number is standard form:
(i) 8 x 10⁴ + 7 x 10³ + 6 x 10² + 5 x 10¹+ 2 x 1 + 4 x 10^-2 + 7 x 10^-4
Solution:
8 x 10⁴ + 7 x 10³ + 6 x 10² + 5 x 10¹ + 2 x 1 + 4 x 10^-2 + 7 x 10^-4
= 8 x 10000 + 7 x 1000 + 6 x 100 + 5 x 10 + 2 x 1 + 4 x \(\frac{1}{100}\) + 7 x \(\frac{1}{10000}\)
= 80000 + 7000 + 600 + 50 + 2 + \(\frac{4}{100}\) + \(\frac{7}{10000}\)
= 87652.0407

(ii) 5 x 10³ + 5 x 10¹ + 5 x 10^-1 + 5 x 10^-3
Solution:
5 x 10³ + 5 x 10¹ + 5 x 10^-1 + 5 x 10^-3
= 5 x 1000 + 5 x 10 + 5 x \(\frac{1}{10}\) + 5 x \(\frac{1}{1000}\)
= 5000 + 50 + \(\frac{5}{10}\) + \(\frac{5}{1000}\) = 5050.505

8th Maths Exercise 1.4 Question 10.
The radius of a hydrogen atom is 2.5 x 10 11 m. Express this number in standard notation.
Solution:
Radius of a hydrogen atom = 2.5 x 10¹¹ m
= 2.5 x \(\frac{1}{10^{11}}\) m = \(\frac{2.5}{10^{11}}\) m = 0.000000000025 m

Samacheer Kalvi Guru 8 Maths Question 11.
Write each number in scientific notation:
(i) 467800000000
(ii) 0.000001972
Solution:

Samacheer Kalvi 8th Maths Solutions Question 12.
Write in scientific notation:
(i) Earth’s volume is about 1,083,000,000,000 cubic kilometers.
Solution:

Earth’s volume = 1.083 x 10¹² cubic kilometers.

(ii) If you fill a bucket with dirt, the portion of the whole Earth that is in the bucket will be 0.0000000000000000000000016 kg.
Solution:
Portion of earth in the bucket =  kg = 1.6 x 10^-24 kg.

Objective Type Questions

Samacheer Kalvi 8th Maths Book Solution Question 13.
By what number should (-4)^-1 be multiplied so that the product becomes 10^-1?
(a) \(\frac{2}{3}\)
(b) \(\frac{-2}{5}\)
(c) \(\frac{5}{2}\)
(d) \(\frac{-5}{2}\)
Solution:
(b) \(\frac{-2}{5}\)
Hint:

Question 14.
0.0000000002020 in scientific form is
(a) 2.02 x 10⁹
(b) 2.02 x 10^-9
(c) 2.02 x 10^-8
(d) 2.02 x 10^-10
Solution:
(d) 2.02 x 10^-10
Hint:

Question 15.
(-2)^-3 x (-2)^-2 is
(a) \(\frac{-1}{32}\)
(b) \(\frac{1}{32}\)
(c) 32
(d) -32
Solution:
(a) \(\frac{-1}{32}\)

Question 16.
Which is not correct?
(a) \(\left(\frac{-1}{4}\right)^{2}\) = 4^-2
(b) \(\left(\frac{-1}{4}\right)^{2}\) = \(\left(\frac{1}{2}\right)^{4}\)
(c) \(\left(\frac{-1}{4}\right)^{2}\) = 16^-1
(d) \(-\left(\frac{1}{4}\right)^{2}\) = 16^-1
Solution:
(d) \(-\left(\frac{1}{4}\right)^{2}\) = 16^-1
Hint:
\((-2)-3 x(-2)-2=(-2)-3-2=(-2)-5\left(-\frac{1}{2}\right) 5=-\frac{1}{32}\)

Question 17.
If \(\left(\frac{p}{q}\right)^{1-3 x}=\left(\frac{q}{p}\right)^{\frac{1}{2}}\), then x is
(a) 4^-1
(b) 3^-1
(c) 2^-1
(d) 1^-1
Solution:
(c) 2^-1
Hint: